If the function / satisfies the relation f(x+y)+f(x−y)=2f(x),f(y)∀x,y∈Randf(0)≠0 , then
f(x) is an even function
f(x) is an odd function
If f(2)=a, then f(−2)=a
If f(4)=b, then f(−4)=−b
The correct Answer is:A, C
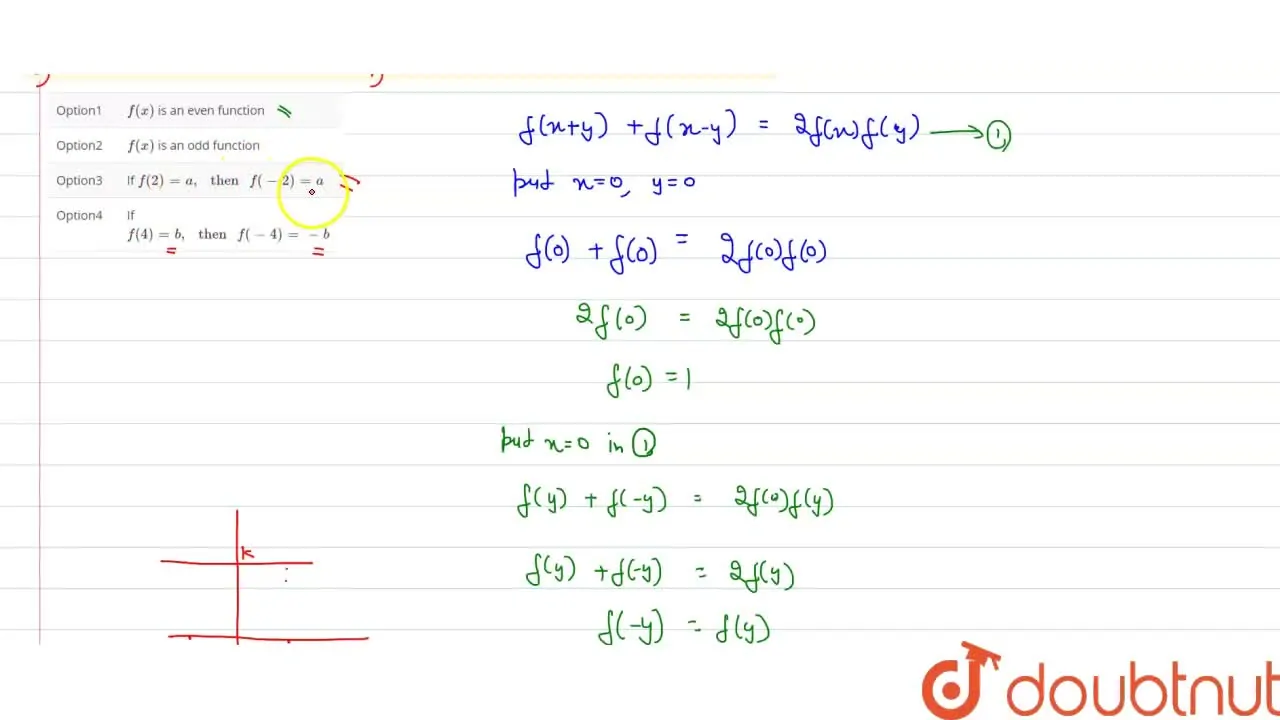
f(x+y)+f(x−y)=2f(x)⋅f(y) (1)
Put x=0. Then f(y)+f(−y)=2f(0)f(y) (2)
Put x=y=0. Then f(0)+f(0)=2f(0)f(0)
∴f(0)=1[as f(0)≠0]
∴f(−y)=f(y) [from (2)]
Hence, the function is even, Then f(−2)=f(2)=a.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Linked Comprehension Type32videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Matrix Match Type9videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Single Correct Answer Type125videos
RELATIONS AND FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)3videos
PROPERTIES AND SOLUTIONS OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.311videos
SCALER TRIPLE PRODUCTS
Similar Questions
If the function fsatisfies the relation f(x+y)+f(x−y)=2f(x),f(y)∀x,y∈Randf(0)≠0 , then f(x)isanevenfunction f(x)isanoddfunction Iff(2)=a,thenf(−2)=a Iff(4)=b,thenf(−4)=−b
View SolutionLet f(x+y)+f(x−y)=2f(x)f(y)∀x,y∈R and f(0)=k, then
View SolutionA function f : R→R satisfies the equation f(x)f(y)−f(xy)=x+y∀x,y∈Randf(1)>0, then
View SolutionSuppose the function f(x) satisfies the relation f(x+y3)=f(x)+f(y3).∀x,y∈R and is differentiable for all x. Statement 1: If f′(2)=a,thenf′(−2)=a Statement 2: f(x) is an odd function.
View SolutionA function f(x) satisfies the relation f(x+y)=f(x)+f(y)+xy(x+y),∀x,y∈R. If f'(0) = - 1, then
View Solutiony=f(x) , where f satisfies the relation f(x+y)=2f(x)+xy(y)+y√f(x)∀x,y∈Randf′(0)=0. Then f(6) is equal of f(−3) is ________
View SolutionLet f(x+y) + f(x-y) = 2f(x)f(y) for x, y∈R and f(0)≠0. Then f(x) must be
View SolutionIf a function satisfies (x−y)f(x+y)−(x+y)f(x−y)=2(x2y−y3)∀x,y∈Randf(1)=2, then
View SolutionIf a function 'f' satisfies the relation f(x)f''(x)−f(x)f′(x)−f′(x)2=0∀x∈R and f(0)=1=f′(0). Then find f(x).
View SolutionIf f is polynomial function satisfying 2+f(x)f(y)=f(x)+f(y)+f(xy)∀x,y∈R and if f(2)=5, then find the value of f(f(2)).
View Solution