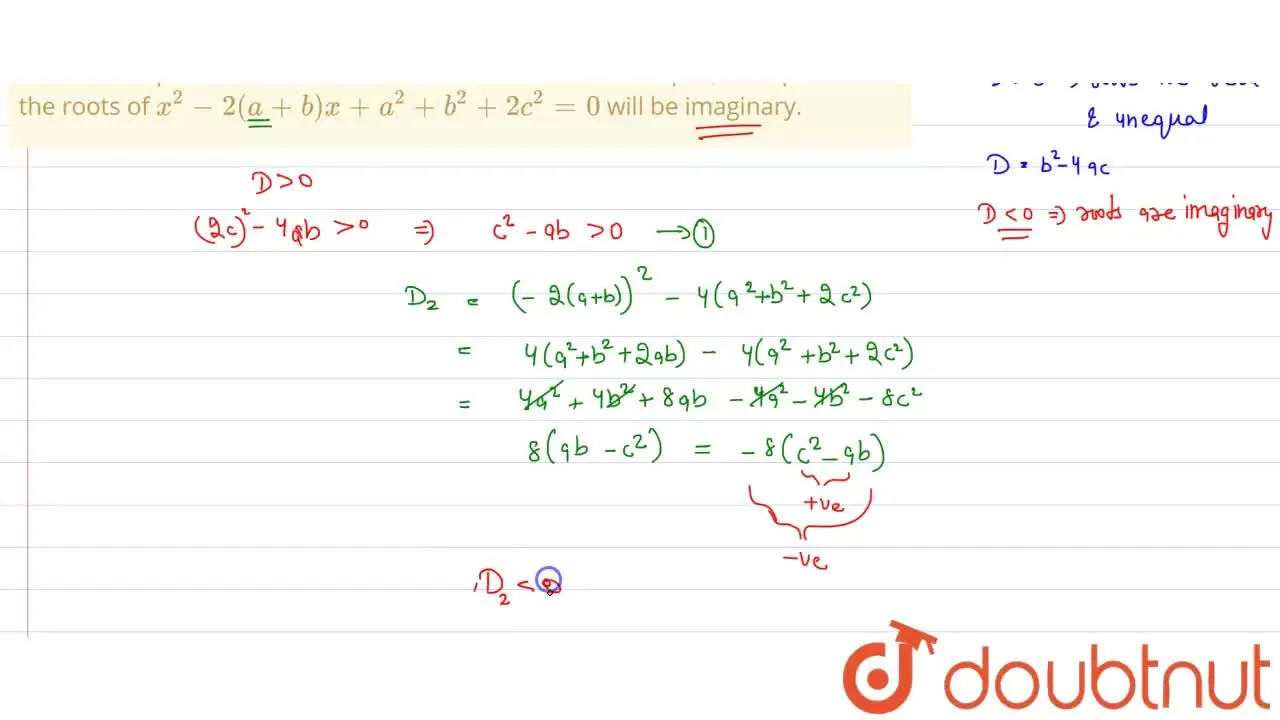
If roots of equation x3−2cx+ab=0 are real and unequal, then prove that the roots of x2−2(a+b)x+a2+b2+2c2=0 will be imaginary.
Roots pf eqution x2−2cx+ab=0 are real and unequal
∴D1>0
∴4c2−4ab>0
or c2−ab>0 (1)
For equation x2−2(a+b)x+(a2+b2+2c2)=0
D2=4(a+b)2−4(a2+b2+2c2)
= −8((c2−ab)<0(om(1))
Therefoue , roots are imaginary.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - SOLVED EXAMPLES14videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - CONCEPT APPLICATION EXERCISE 2.13videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Multiple Correct Answer6videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)1videos
STRAIGHT LINES
- BOOK - CENGAGE ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions294videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
If roots of equation x2−2cx+ab=0 are real and unequal, then prove that the roots of x2−2(a+b)x+a2+b2+2c2=0 will be imaginary.
View SolutionIf the roots of the equation x2+2cx+ab=0 are real and unequal, then the roots of the equation x2−2(a+b)x+(a2+b2+2c2)=0 are: (a) real and unequal (b) real and equal (c) imaginary (d) rational
View SolutionIf the roots of the equation , x2+2cx+ab=0 are real and unequal, then the roots of the equation, x2−2(a+b)x+(a2+b2+2c2)=0 are: a. real and unequal b. real and equal c. imaginary d. Rational
View SolutionIf the roots of the equation x2+2cx+ab=0 are real unequal, prove that the equation x2−2(a+b)x+a2+b2+2c2=0 has no real roots.
View SolutionIf the roots of the equation qx2+2px+2q=0 are real and unequal, prove that the roots of the equation (p+q)x2+2qx+(p−q)=0 are imaginary.
View SolutionIf the roots of the equation (b−c)x2+(c−a)x+(a−b)=0 are equal, then prove that 2b=a+c.
View SolutionIf the roots of the equation (b−c)x2+(c−a)x+(a−b)=0 are equal, then prove that 2b=a+c
View SolutionIf the roots of ax2+x+b=0 be real and unequal, show that the roots of x2+1x=4√ab are imaginary.
View SolutionIf the roots of equation a(b−c)x2+b(c−a)x+c(a−b)=0 be equal prove that a,b,c are in H.P.
View SolutionColumn I, Column II If a,b,c,andd are four zero real numbers such that (d+a−b)2+(d+b−c)2=0 and he root of the equation a(b−c)x2+b(c−a)x=c(a−b)=0 are real and equal, then, p. a+b+c=0 If the roots the equation (a2+b2)x2−2b(a+c)x+(b2+c2)=0 are real and equal, then, q. a,b,care∈A.P. If the equation ax2+bx+c=0andx3−3x2+3x−0 have a common real root, then, r. a,b,care∈G.P. Let a,b,c be positive real number such that the expression bx2+(√(a+b)2+b2)x+(a+c) is non-negative ∀x∈R, then, s. a,b,care∈H.P.
View Solution