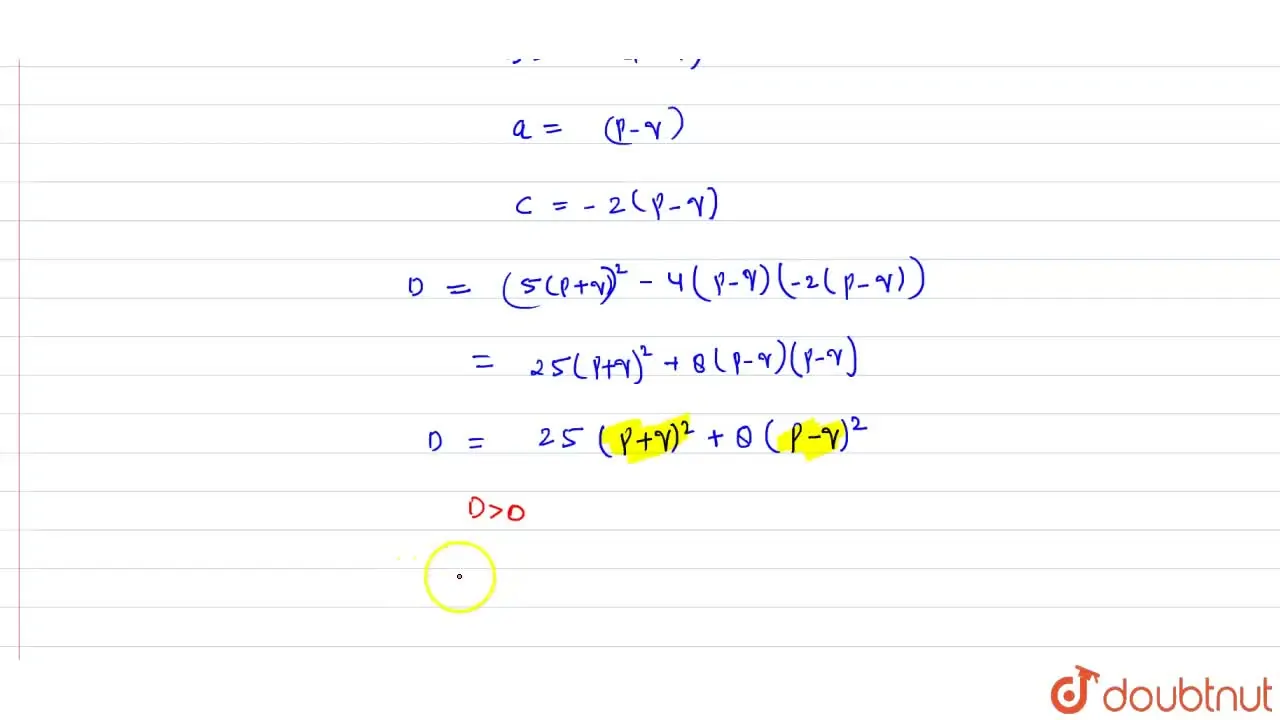
If p,q are real p≠q, then show that the roots of the equation (p−q)x2+5(p+q)x−2(p−q)=0 are real and unequal.
Step by step video & image solution for If p ,q are real p!=q , then show that the roots of the equation (p-q)x^2+5(p+q)x-2(p-q)=0 are real and unequal. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
Similar Questions
If p,q are real p≠q, then show that the roots of the equation (p−q)x2+5(p+q)x−2(p−q)=0 are real and unequal.
View SolutionIf the roots of the equation qx2+2px+2q=0 are real and unequal, prove that the roots of the equation (p+q)x2+2qx+(p−q)=0 are imaginary.
View SolutionIf p,q,r,s are real and pr>4(q+s) then show that at least one of the equations x2+px+q=0andx2+rx+s=0 has real roots.
View SolutionIf p,q,rands are real numbers such that pr=2(q+s), then show that at least one of the equations x2+px+q=0 and x2+rx+s=0 has real roots.
View SolutionIf the roots of the equation (1−q+p22)x2+p(1+q)x+q(q−1)+p22=0 are equal, then show that p2=4q
View SolutionIf p,q,r are positive and are in A.P., the roots of quadratic equation px2+qx+r=0 are all real for a.∣∣∣rp−7∣∣∣≥4√3 b. ∣∣pr−7∣∣≥4√3 c. all p and r d. no p and r
View SolutionIf 2+√3i is a root of the equation x2+px+q=0 , then write the values of p and q .
View SolutionShow that if p,q,r and s are real numbers and pr=2(q+s), then atleast one of the equations x2+px+q=0 and x2+rx+s=0 has real roots.
View SolutionIf a,b,p,q are non zero real numbers, then how many comman roots would two equations: 2a2x2−2abx+b2=0andp2x2+2pqx+q2=0 have?
View SolutionShow that the sequence (p+q)2,(p2+q2),(p−q)2… is an A.P.
View Solution