If limx→1x3−1x−1=limx→kx4−k4x3−k3, find the value of k.
Video Solution
Text Solution
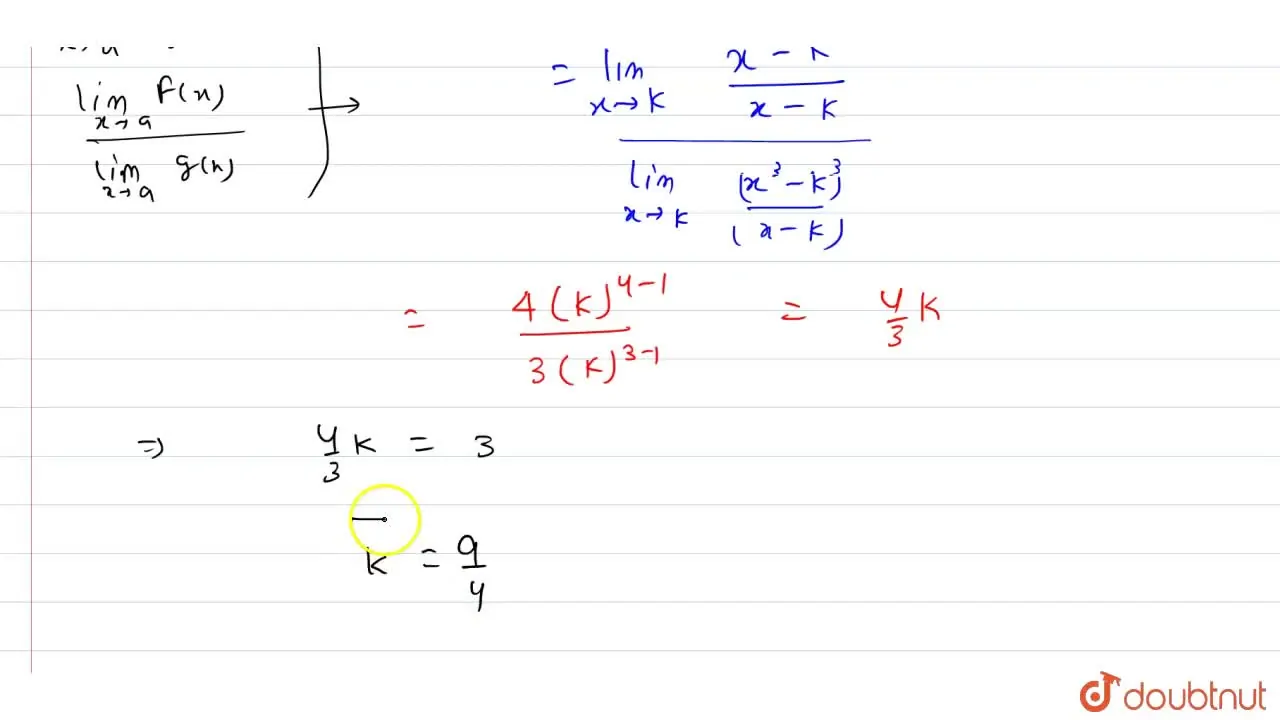
Firstly, we have limx→1x3−1x−1=limx→1(x)3−(1)3x−1=3(1)2=3
Similarly, limx→kx4−k4x3−k3=limx→kx4−k4x−kx3−k3x−k=limx→kx4−k4x−k/limx→kx3−k3x−k=4(k)3/3(k)2=43k
Now, limx→1x3−1x−1=limx→kx4−k4x3−k3⇒3=43K⇒k=94
|
Updated on:21/07/2023
Related Playlists
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LIMITS AND DERIVATIVES EXERCISE - Try yourself64videos
LIMITS AND DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LIMITS AND DERIVATIVES EXERCISE - SECTION - A50videos
LIMITS AND DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - LIMITS AND DERIVATIVES EXERCISE - Section - j3videos
LIMITS AND DERIVATIVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - ASSIGNMENT (SECTION - J)(ANKASH CHALLENGERS QUESTIONS)4videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MATHEMATICAL REASONINGEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)15videos
MATHEMATICAL REASONING
Similar Questions
Evaluate, limx→1x4−1x−1=limx→kx3−k3x2−k2 , then find the value of k.
View SolutionIf Limx→1x4−1x−1=Limx→kx3−k3x2−k2 , find the value of k .
View SolutionEvaluate limx→1x3−1x−1
View SolutionIf limx→ax√x−a√ax−1=limx→3x3−27x−3, find the value of a.
View SolutionIf limx→2x−23√x−3√2=limx→kx2−k2x−k find the value of K
View Solutionlimx→13xx−1
View SolutionEvaluate limx→1[3x4+4]
View SolutionEvauate limx→1x4−3x4+2x3−5x2+3x+1.
View SolutionIf limx→0(4x−1)13+a+bxx=13 then find the values of a and b.
View SolutionIf limx→2tan(x−2).(x2+(k−2)x−2k)(x2−4x+4)=5, then find the value of k.
View Solution