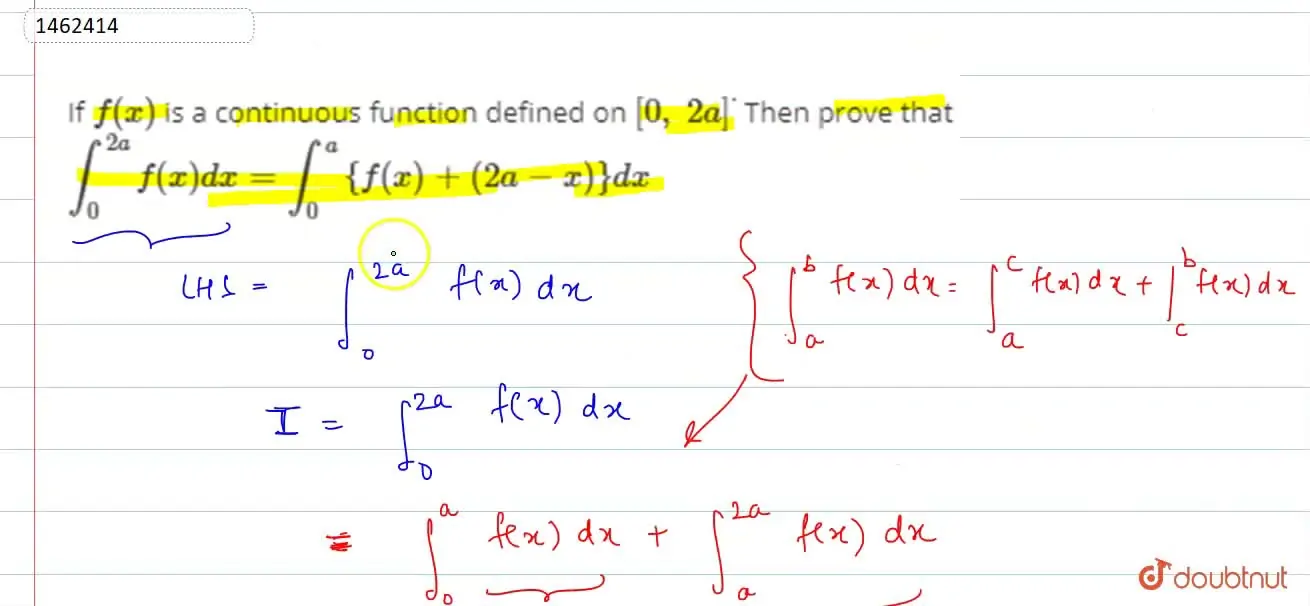
If f(x) is a continuous function defined on [0, 2a]. Then prove that ∫2a0f(x)dx=∫a0{f(x)+(2a−x)}dx
Video Solution
Text Solution
We have to proof ∫2a0f(x)dx=∫a0{f(x)+(2a−x)}dx
Take , L.H.S
I=∫2a0f(x)dx
As, ∫baf(x)dx=∫caf(x)dx+∫bcf(x)dx
I=∫a0f(x)dx+∫2aaf(x)dx
I=I1+I2
I2=∫2aaf(x)dx
Let 2a−x=t⇒x=2a−t⇒dx=−dt
And limits changes as x=a⇒t=a and x=2a⇒t=0
I2=−∫0af(2a−t)(dt)
I2=∫a0f(2a−t)dt
I2=∫a0f(2a−x)dx
∫20af(x)dx=∫a0f(x)dx=∫a0f(2a−x)dx
∫20af(x)dx=∫a0[f(x)+f(2a−x)]dx
Hence proved
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Prove that: ∫2a0f(x)dx=∫2a0f(2a−x)dx.
View SolutionProve that ∫aof(x)dx=∫aof (a−x)dx.
View SolutionProve that ∫2a0f(x)dx=∫aa[f(a−x)+f(a+x)]dx
View Solution∫a0f(a−x) dx=
View SolutionProperty 6: If f(x) is a continuous function defined on [0,2a] then ∫2a0f(x)dx=∫a0f(x)dx+∫a0f(2a−x)dx
View Solution∫2a0f(x)dx−∫a0f(x)dx=
View Solution∫2a0f(x)dx−∫a0f(x)dx=
View SolutionProve that ∫t0f(x)g(t−x)dx=∫t0g(x)f(t−x)dx
View SolutionProve that ∫2−2f(x4)dx=2∫20f(x4)dx
View SolutionProperty 5: If f(x) is a continuous function defined on [0,a] then ∫a0f(x)dx=∫a0f(a−x)dx
View Solution