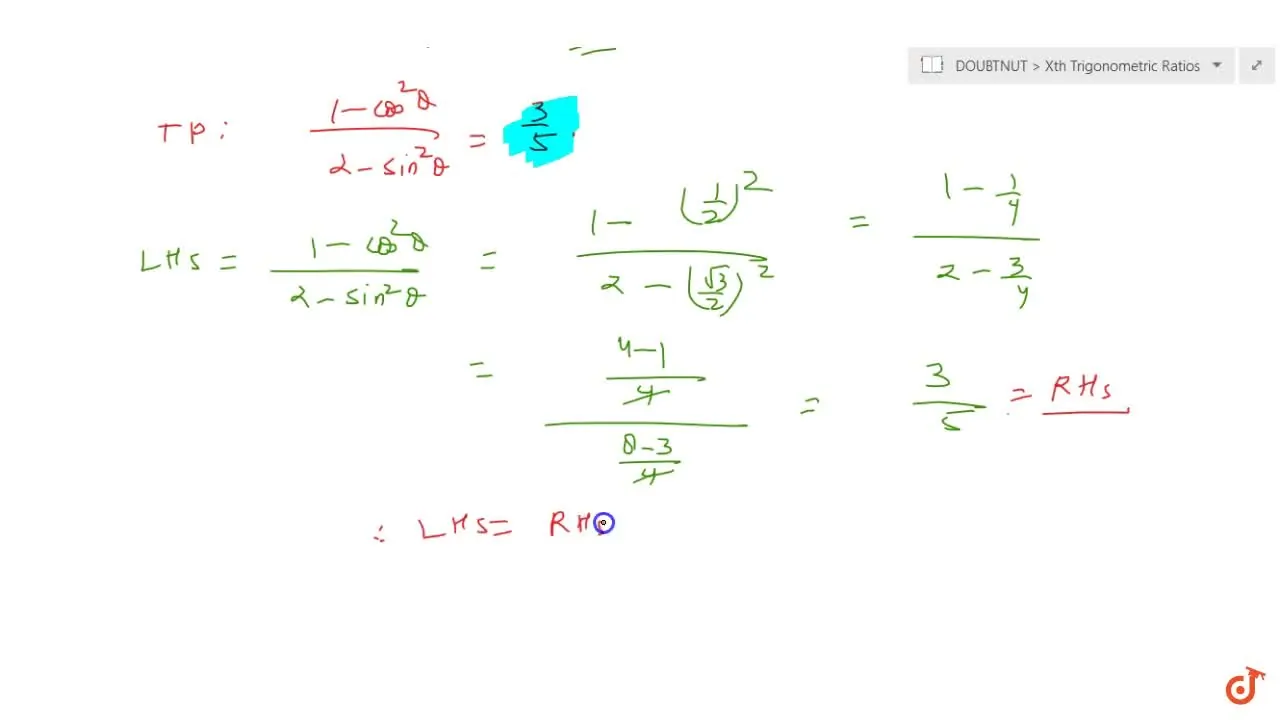
If cotθ=1√3 , show that 1−cos2θ2−sin2θ=35
Video Solution
|
Answer
Step by step video & image solution for If cottheta=1/(sqrt(3)) , show that (1-cos^2theta)/(2-sin^2theta)=3/5 by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
If cotθ=1√3 , show that 1−cos2θ2−sin2θ=35
View SolutionIf cotθ=1√3 , find the value of 1−cos2θ2−sin2θ
View SolutionIf cosθ+sinθ=√2cosθ, show that cosθ−sinθ=√2sinθ
View SolutionIf cosθ+sinθ=√2cosθ then show that cosθ−sinθ=√2sinθ
View SolutionIf cosθ+sinθ=√2cosθ; show that cosθ−sinθ=√2sinθ
View SolutionIf 3cotθ=4 then show that 1−tan2θ1+tan2θ=(cos2θ−sin2θ)
View SolutionIf sinθ=12, show that (3cosθ−4cos3θ)=0
View SolutionIf 3cotθ=2 , show that (4sinθ−3cosθ)(2sinθ+6cosθ)=13
View SolutionIf tanθ=1√3, prove that 7sin2θ+3cos2θ=4
View SolutionIfsinθ=34, then prove that √cosec2θ−cot2θsec2θ−1=√73
View Solution