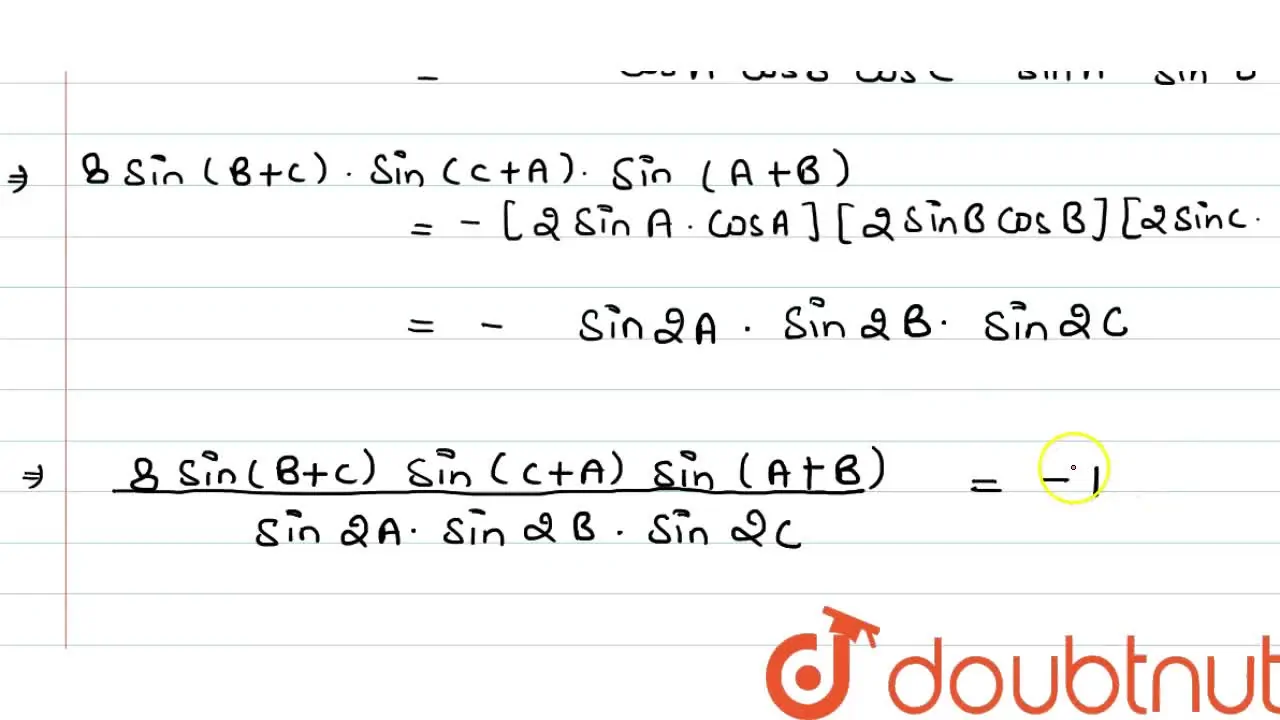
If cos(A+B+C)=cosAcosBcosC, then find the value of 8sin(B+C)sin(C+A)sin(A+B)sin2Asin2Bsin2C
8
4
−3
−1
The correct Answer is:D
Step by step video & image solution for If cos(A+B+C)=cosAcosBcosC , then find the value of (8sin(B+C)sin(C+A)sin(A+B))/(sin2Asin2Bsin2C) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
If any triangle ABC, find the value of asin(B−C)+bsin(C−A)+csin(A−B).
View SolutionIf A+B+C=π then sin2A+sin2B+sin2C=
View SolutionIn triangle ABC, prove that sin(B+C−A)+sin(C+A−B)+sin(A+B−C)=4sinAsinBsinC.
View Solutionsin(A−C)+2sinA+sin(A+C)sin(B−C)+2sinB+sin(B+C) is equal to
View SolutionProve that:
sin(A−C)+2sinA+sin(A+C)sin(B−C)+2sinB+sin(B+C)=sinAsinBView SolutionIn any triangle ABC , prove that following: a2sin(B−C)sinA+b2sin(C−A)sinB+c2sin(A−B)sinC=0
View SolutionLet a, b and c denote the sides BC, CA and AB respectively of ABC . If |1ab1ca1bc|=0, then find the value of sin2A+sin2B+sin2C .
View SolutionA triangle ABC is right angled at B. Find the value of secA.sinC−tanA.tanCsinB
View Solution19. Prove that sin(A+B)sin(A−B)+sin(B+C)sin(B−C)+sin(C+A)sin(C−A)=0
View SolutionIf in triangle ABC,∠C=450 then find the range of the values of sin2A+sin2B.
View Solution