If a circle and the rectangular hyperbola xy=c2 meet in four points 't'1,'t'2,'t'3 and 't'4 then prove that t1t2t3t4=1.
To prove that t1t2t3t4=1 for the points of intersection of the circle and the rectangular hyperbola xy=c2, we can follow these steps:
Step 1: Set up the equations
The equation of the rectangular hyperbola is given by:
xy=c2
We can express y in terms of x:
y=c2x
Step 2: General equation of the circle
The general equation of a circle can be written as:
x2+y2+2gx+2fy+c1=0
For our case, we can assume c1=0 (without loss of generality).
Step 3: Substitute y into the circle's equation
Substituting y=c2x into the circle's equation, we have:
x2+(c2x)2+2gx+2f(c2x)=0
This simplifies to:
x2+c4x2+2gx+2fc2x=0
Step 4: Multiply through by x2 to eliminate the fractions
Multiplying the entire equation by x2 gives:
x4+c4+2gx3+2fc2x=0
This can be rearranged to:
x4+2gx3+2fc2x+c4=0
Step 5: Identify the roots
Let the roots of this polynomial equation be t1,t2,t3,t4. By Vieta's formulas, we know that for a polynomial of the form:
x4+ax3+bx2+cx+d=0
the product of the roots t1t2t3t4 is given by:
t1t2t3t4=d1
where d is the constant term.
Step 6: Find the constant term
In our equation:
x4+2gx3+0⋅x2+2fc2x+c4=0
The constant term d=c4.
Step 7: Evaluate the product of the roots
Thus, we have:
t1t2t3t4=c41=c4
Step 8: Relate back to the hyperbola
Since t1,t2,t3,t4 are the coordinates of intersection points of the hyperbola xy=c2, we can express the product of the roots in terms of c2:
t1t2t3t4=1
This is because if we consider the transformation t=cx, it leads to the conclusion that the product of the transformed coordinates is equal to 1.
Conclusion
Thus, we have shown that:
t1t2t3t4=1
The equation of the rectangular hyperbola is given by:
xy=c2
We can express y in terms of x:
y=c2x
The general equation of a circle can be written as:
x2+y2+2gx+2fy+c1=0
For our case, we can assume c1=0 (without loss of generality).
Substituting y=c2x into the circle's equation, we have:
x2+(c2x)2+2gx+2f(c2x)=0
This simplifies to:
x2+c4x2+2gx+2fc2x=0
Multiplying the entire equation by x2 gives:
x4+c4+2gx3+2fc2x=0
This can be rearranged to:
x4+2gx3+2fc2x+c4=0
Let the roots of this polynomial equation be t1,t2,t3,t4. By Vieta's formulas, we know that for a polynomial of the form:
x4+ax3+bx2+cx+d=0
the product of the roots t1t2t3t4 is given by:
t1t2t3t4=d1
where d is the constant term.
In our equation:
x4+2gx3+0⋅x2+2fc2x+c4=0
The constant term d=c4.
Thus, we have:
t1t2t3t4=c41=c4
Since t1,t2,t3,t4 are the coordinates of intersection points of the hyperbola xy=c2, we can express the product of the roots in terms of c2:
t1t2t3t4=1
This is because if we consider the transformation t=cx, it leads to the conclusion that the product of the transformed coordinates is equal to 1.
Thus, we have shown that:
t1t2t3t4=1
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - HYPERBOLAEXERCISE - ASSIGNMENT PROBLEMS ( SUBJECTIVE) Level - II11videos
HYPERBOLA
- BOOK - FIITJEECHAPTER - HYPERBOLAEXERCISE - ASSIGNMENT PROBLEMS ( OBJECTIVE) Level - I47videos
HYPERBOLA
- BOOK - FIITJEECHAPTER - HYPERBOLAEXERCISE - Exercise - 36videos
HYPERBOLA
- BOOK - FIITJEECHAPTER - HEIGHTS & DISTANCE EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-II20videos
HEIGHTS & DISTANCE
- BOOK - FIITJEECHAPTER - INDEFINTE INTEGRALEXERCISE - EXERCISE-81videos
INDEFINTE INTEGRAL
Similar Questions
Knowledge Check
- Question 1 - Select One
Statement :1 If a parabola y2=4ax intersects a circle in three co-normal points then the circle also passes through the vertr of the parabola. Because
Statement : 2 If the parabola intersects circle in four points t1,t2,t3andt4 then t1+t2+t3+t4=0 and for co-normal points t1,t2,t3 we have t1+t2+t3=0.AStateme-1 is True, Statement-2 is True, Statemetn-2 is correct explanation for Statement-1BStatement-1 is True, Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1CStatement-1 is True, Statement-2 is FalseDStatement-1 is False, Statement-2 is ture - Question 2 - Select One
If a circle cuts a rectangular hyperbola xy=1 in four points P,Q,R,S and the parameters of these four points be t1,t2,t3andt4 respectively and −20t1t2t3t4=k, then value of k equals
A1B−1C−4Dnone - Question 3 - Select One
If the normal to the rectangular hyperbola xy=4 at the point (2t,2t1) meets the curve again at (2t2,2t2), then
At13t2=1Bt13t2=−1Ct23t1=1Dt1t32=−1
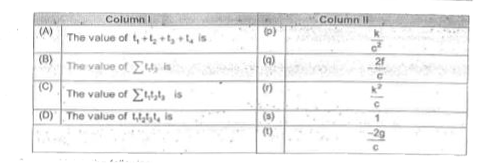
Let a circle x2+y2+2gx+2fy+k=0 cuts a rectangular hyperbola xy=c2 in (ct1,ct1),(ct2,ct2),(ct3,ct3) and (ct4,ct4) .
 View Solution
View SolutionShow that the normal to the rectangular hyperbola xy=c2 at the point t meets the curve again at a point t' such that t3t'=−1.
View SolutionIf a circle intersects the parabola y2=4ax at points A(at21,2at1),B(at22,2at2),C(at23,2at3),D(at24,2at4), then t1+t2+t3+t4 is
View SolutionPoints A,B,C,D, on y2=4ax have parameters t1,t2,t3,t4 respectively . If AB ⊥ CD then (t1+t2)(t3+t4)=
View SolutionThe point of intersection of tangents at 't'1 and 't'2 to the hyperbola xy=c2 is
View Solution
