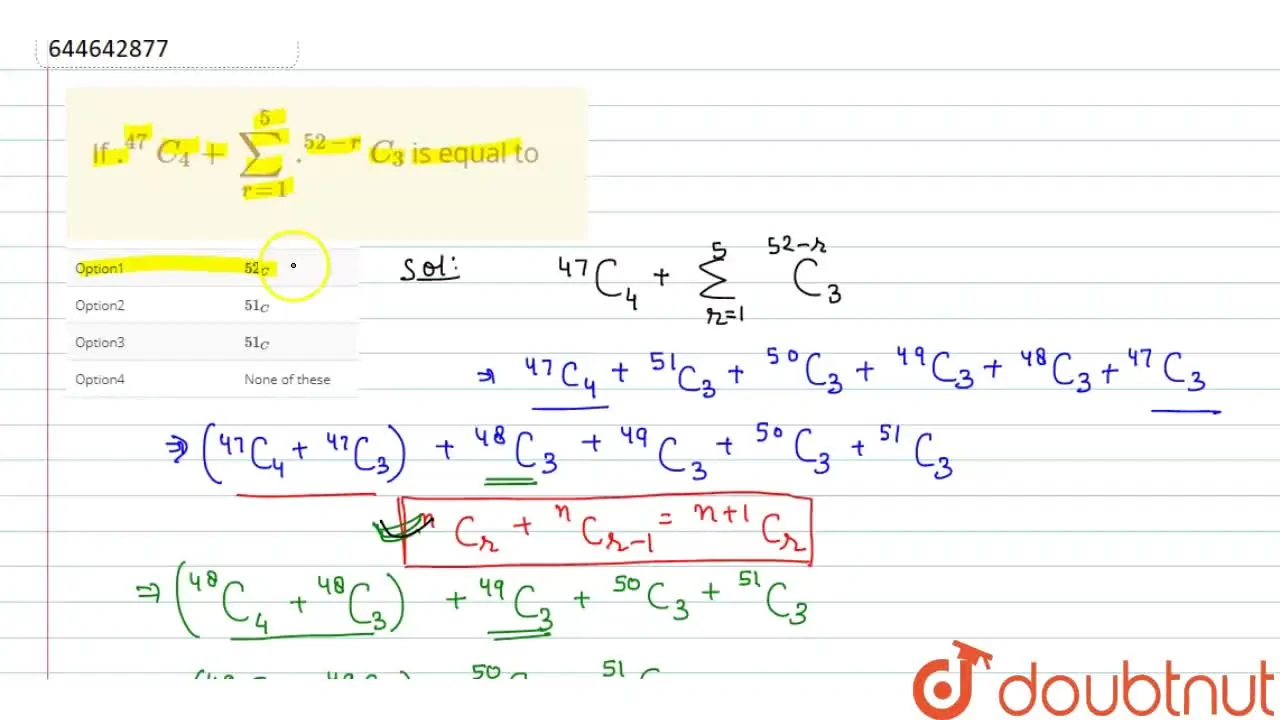
If .47C4+5∑r=1.52−rC3 is equal to
52C
51C
51C
None of these
The correct Answer is:A
Step by step video & image solution for If .^(47)C_(4)+underset(r=1)overset(5)(sum).^(52-r)C_(3) is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - NUMERICAL VALUE TYPE FOR JEE MAIN14videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - JEE Archive56videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - LEVEL 189videos
BINOMIAL THEOREM
- BOOK - VMC MODULES ENGLISHCHAPTER - CIRCLESEXERCISE - JEE ADVANCED ( ARCHIVE )68videos
CIRCLES
Similar Questions
n−1∑r=1cos2rπn is equal to
View Solutionn∑r=1r(.nCr−.nCr−1) is equal to
View SolutionWhat is .47C4+.51C3+5∑j=2.52−jC3 equal to ?
View SolutionFind the value of 47C4+5∑r=152−rC3
View SolutionThe value of 50C4+6∑r=156−rC3 is
View Solutionlet 2010∑r=0arxr=(1+x+x2+x3+x4+x5)402and2010∑r=0ar=a, then the value of (∑2010r=0r.ar∑2010r=0ar) is equal to____.
View Solutionlimn→∞ 3n∑r=2n+1nr2−n2 is equal to
View SolutionIf (1+a)(1+a2)(1+a4)....(1+a128)=n∑r=0ar, then n is equal to
View SolutionIf Cr stands for .nCr=n!r!n−r!andn∑r=1r.C2r=λ for n≥2, then λ is divisible by
View SolutionIf (1−x3)n=n∑r=0arxr(1−x)3n−2r, then the value of ar, where n∈N is
View Solution