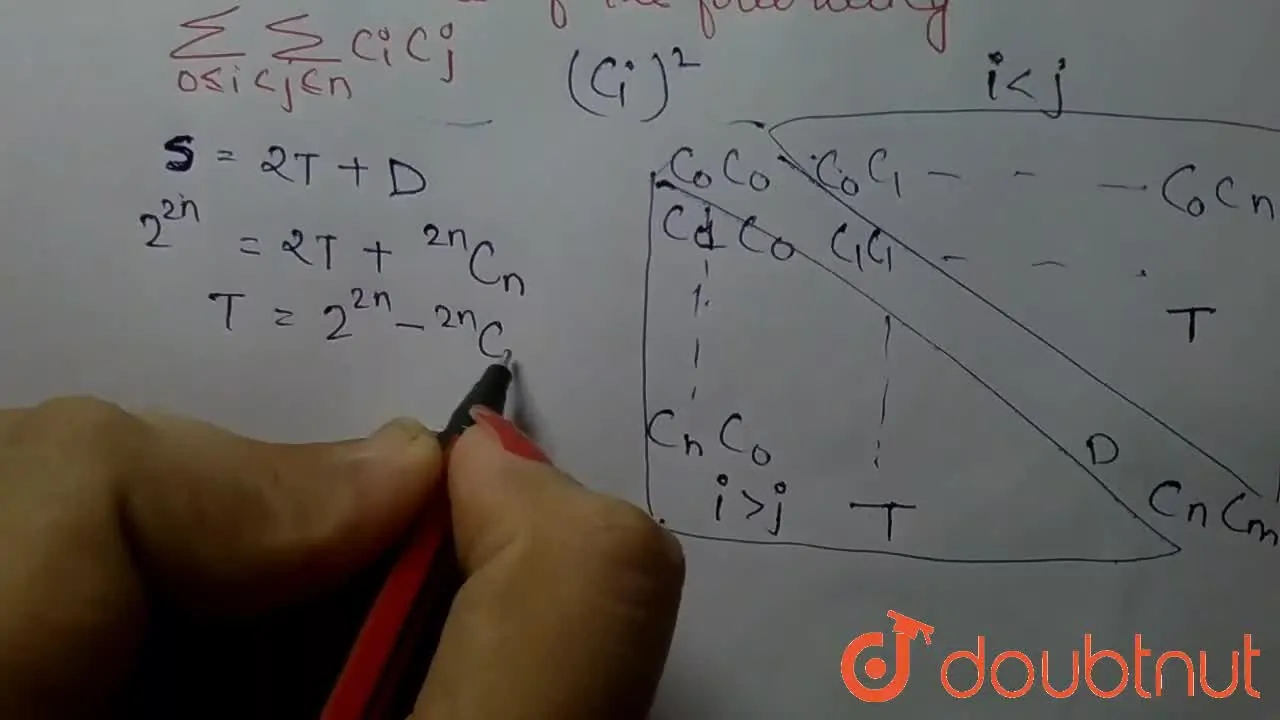
If ,
find the values of the following
[from corollary I]
.
Related Playlists
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - JEE Type Solved Example : (Matching Type Questions )2videos
BIONOMIAL THEOREM
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise For Session 17videos
BIONOMIAL THEOREM
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - AREA OF BOUNDED REGIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)23videos
AREA OF BOUNDED REGIONS
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)16videos
CIRCLE
Similar Questions
If (1+x)n=C0=C1x+C2x2+…+Cnxn , find the values of the following (∑∑)0≤i<j≤njCi
View SolutionIf (1+x)n=C0=C1x+C2x2+…+Cnxn ,
find the values of the following
(∑∑)0≤i≤j≤n(i+j)(Ci±Cj)2View SolutionIf (1+x)n=C0+C1x+C2x2+…+Cnxn , find the values of the following . n∑i=0n∑j=0(i+j)CiCj
View SolutionIf (1+x)n=C0+C1x+C2x2+C3x3+…+Cnxn , find the sum of the series
C02−C16+C210+C314−...+(−1)nCn4n+2.View SolutionIf (1+x)n=C0+C1x+C2x2
+C3x3+C4x4+..., find the values of
(i) C0−C2+C4−C0+…
(ii) C1−C3+C5−C7+…
(iii) C0+C3+C6+…View SolutionIf (1+x)n=C0+C1x+C2x2+….+Cnxn, then the value of ∑∑0≤r<s≤n(Cr+Cs)2 is :
View SolutionIf (1+x)n=C0+C1x+C2x2+….+Cnxn, then the value of ∑∑0≤r<s≤n(r+s)(Cr+Cs) is :
View SolutionIf (x+a1)(x+a2)(x+a3)…(x+an)=xn+S1xn−1+S2xn−2+…+Sn
where , S1=n∑i=0ai,S2=(∑∑)1≤i<j≤naiaj,S3(∑∑∑)1≤i<k≤naiajak
and so on .
If (1+x)n=C0+C1x+C2x2+...+Cnxn the
cefficient of xn in the expansion of
(x+C0)(x+C1)(x+C2)...(x+Cn) isView SolutionIf (1+x)n=C0+C1x+C2x2+…+Cnxn, prove that C0Cn−C1Cn−1+C2Cn−2−…+(−1)nCnC0=0 or (−1)n/2n!(n/2)!(n/2)! , according as n is odd or even .
View SolutionIf (1+x)n=C0+C1x+C2x2+…+Cnxn , prove that
C0+2C1+3C2+…+(n+1)Cn=(n+2)2n−1 .View Solution