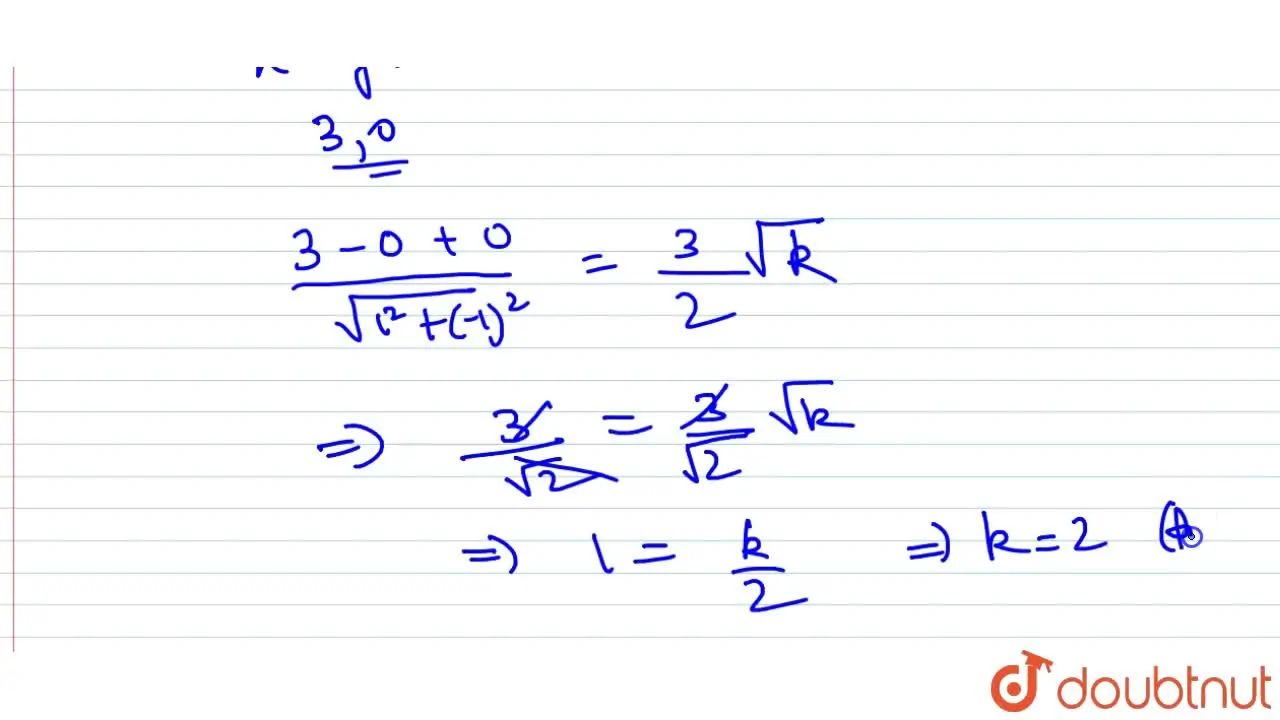
Given that shortest distance of the bisector of xy = 0 from the point (3 , 0) is 32√k Then , the value of k is :Solution in Bengali
[2]
Related Playlists
Similar Questions
The number of terms in the expansion of (x+1x)29is
04:29View SolutionProve that the radii of circles x2+y2=1,x2+y2−2x−6y=6 and x2+y2−4x−12y=9 are in A.p.
01:58View SolutionThe shortest distance between the point (32,0) and the curve y=√x,(x>0), is
02:01View SolutionThe shortest distance of the point (1, 3, 5) from
x2+y2=0 is08:30View Solutionयदि बिंदु (3, k) तथा (k, 5) से बिंदु (0, 2) की दूरियाँ समान हों, तो k का मान ज्ञात करें।
07:50View Solutionयदि बिन्दु (x, y) की दूरी बिन्दु (-3, 0) और (3, 0) से 4 इकाई हो, तो x, y का मान ज्ञात कीजिये ।
06:15View SolutionFind the coordiantes of the point in the xy-plane which is equiistant from the points A ( 0 , 0 , 1) , B ( 2 , 0 , 3) and C ( 0 , 3 , 2) .
08:20View SolutionXY - समतल पर वह बिंदु ज्ञात कीजिए जो बिंदुओं A (2 , 0 , 3) , B ( 0 , 3 , 2) तथा C ( 0 ,0 ,1) से समदूरस्थ है ।
02:49View SolutionLet K be a positive real number and
A=⎡⎢ ⎢⎣2k−12√k2√k2√k1−2k−2√k2k−1⎤⎥ ⎥⎦ and ⎡⎢ ⎢⎣02k−1√k1−2k02−√k−2√k0⎤⎥ ⎥⎦
If det (adjA)+det(adjB)=106 , then [k] is equal to03:05View Solution(-2, 5) ও (4, 3) বিন্দু দুটির সংযোজক সরলরেখা (k, 0) ও (2,3k) বিন্দু দুটির সংযােজক সরলরেখার ওপর লম্ব, k-এর মান নির্ণয় করাে।
04:00View Solutionयदि बिन्दु (3,k) तथा (4,5) से बिन्दु (0, 2) की दूरियाँ समान हों, तो का मान ज्ञात करें
05:11View SolutionThe shortest distance between the point (32,0) and the curve y=√x,(x>0), is
05:23View SolutionFind the values of x, y if the distances of the point (x, y) from (−3, 0) as well as from (3, 0) are 4.
01:14View SolutionLet A be a positive real number and value A=[(2k-1,2sqrtk,2sqrtk),(2sqrtk,1,-2k),(-2sqrtk,2k,-1)] And B=[(0,2k-1,sqrtk),(1-2k,0,2sqrtk),(-sqrtk,-2sqrtk,0)] If det(adjA)+det(adjB)=10^(6) So the value of [k] is
02:00View SolutionDistance of the point (3, 4, 5) from the origin (0, 0, 0) is
07:58View Solution