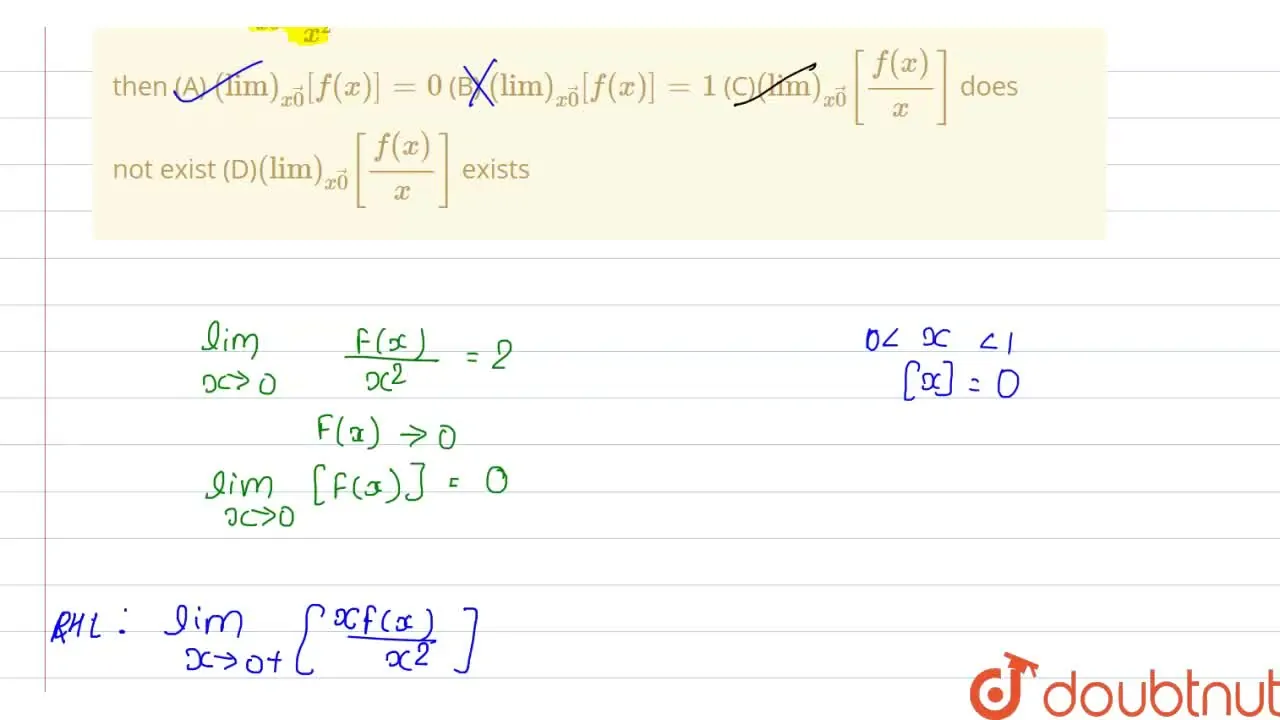
Given (lim)x→0f(x)x2=2,where [.] denotes the greatest integer function, then (A) (lim)x→0[f(x)]=0 (B) (lim)x→0[f(x)]=1 (C)(lim)x→0[f(x)x] does not exist (D)(lim)x→0[f(x)x] exists
Step by step video & image solution for Given ("lim")_(xvec0)(f(x))/(x^2)=2, \where\ [dot] denotes the greatest integer function, then (A) ("lim")_(xvec0)[f(x)]=0 (B) ("lim")_(xvec0)[f(x)]=1 (C)("lim")_(xvec0)[(f(x))/x] does not exist (D)("lim")_(xvec0)[(f(x))/x] exists by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - All Questions68videos
LINEAR INEQUALITIES
Similar Questions
Given limx→0f(x)x2=2, where [.] denotes the greatest integer function, then
View SolutionEvaluate: (lim)x→0tanxx where [.] represents the greatest integer function
View SolutionEvaluate: (lim)x→0sinxx,where[.] represents the greatest integer function.
View SolutionEvaluate: (lim)x→0ex+e−x−2x2
View SolutionLet f(x)=x2−9x+20x−[x] (where [x] is the greatest integer not greater than x. Then (A)(lim)x→5f(x)=1 (B) (lim)x→5f(x)=0 (C) (lim)x→5f(x) does not exist. (D)none of these
View SolutionIf [.] denotes the greatest integer function, then (lim)x→0xa[bx] a. ba b. 0 c. ab d. does not exist
View SolutionEvaluate: (lim)x→0ex^2−cosxx2
View SolutionEvaluate: (lim)x→0tanx−sinxx3
View SolutionIf f(x)=x(e|x|+[x]−2|x|+[x]) then (where [.] represents the greatest integer function) (lim)x→0+f(x)=−1 b. (lim)x→0−f(x)=0 c. (lim)x→0□f(x)=−1 d. (lim)x→0□f(x)=0
View SolutionEvaluate: (lim)x→0x2x−x1−cosx
View Solution