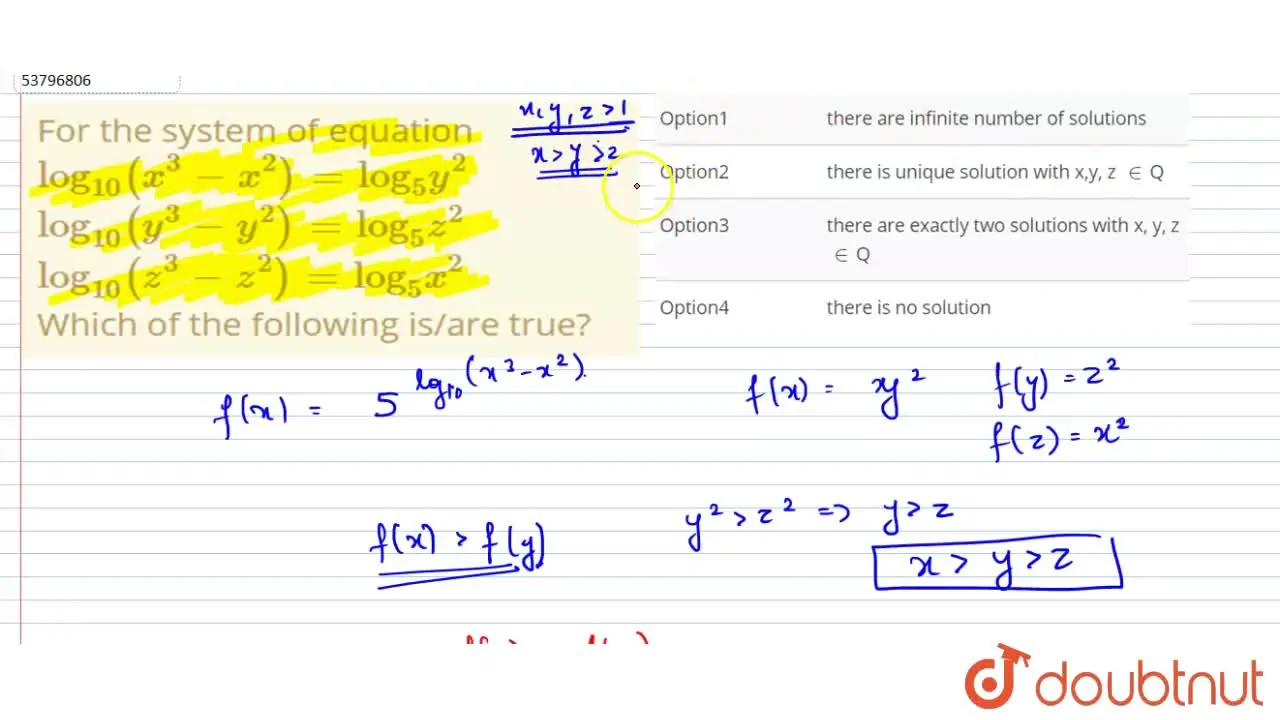
For the system of equation
log10(x3−x2)=log5y2
log10(y3−y2)=log5z2
log10(z3−z2)=log5x2
Which of the following is/are true?

A

there are infinite number of solutions

B

there is unique solution with x,y, z ∈Q

C

there are exactly two solutions with x, y, z ∈Q

D

there is no solution

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

The given equations are meaningful for x, y, z > 1.
Let f(x)=5log10(x3−x2). Then, the given equations are
f(x)=y2,f(y)=z2 and f(z)=x2
For x>1, we find that x3−x2 is an increasing function. So, f(x) is an increasing function.
Letx>y. Then,
f(x)>f(y) [∵fis increasing]
⇒y2>z2⇒y>z
∴x>z
But, y>z
⇒f(y)>f(z) [∵f is increasing]
⇒z2>x2⇒z>x, which is a contradiction.
therefore x cancel(gt) y
Similarly, x<y leads us to a contradiction. Therefore, x cancel(lt) y.
Hence, x = y
Thus, we have x= y =z.
Now,
log10(x3−x2)=log5y2
⇒log10(x3−x2)=log5x2
Let log5x=t.i.e.,x=5'. Then,
log10(x3−x2)=log5x2
⇒log10(53t−52t)=2t
⇒53t−52t=102t⇒5t−1=4t⇒5t−4t=1
Now, 5t−4t is an increasing function of t.
∴5t−4t=1⇒t=1
Putting t = 1 in x =5t we obtain x = 5.
Hence, x = y = z = 5 is the unique solution of the given system of equations.

Updated on:21/07/2023
|
Class 11MATHSLOGARITHM AND ITS APPLICATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • If log10x=y, then log103x2 equals

    A13y
    B23y
    C32y
    D3y

  • If logyx=logzy=logxz, then

    Ax<y<z
    Bx>y≥z
    Cx<y≤z
    Dx=y=z

  • The solution set of the system of equations logxx+log3y=2+log32 and log27(x+y)=23 is

    A(6,3)
    B(3,6)
    C(6,12)
    D(12,6)