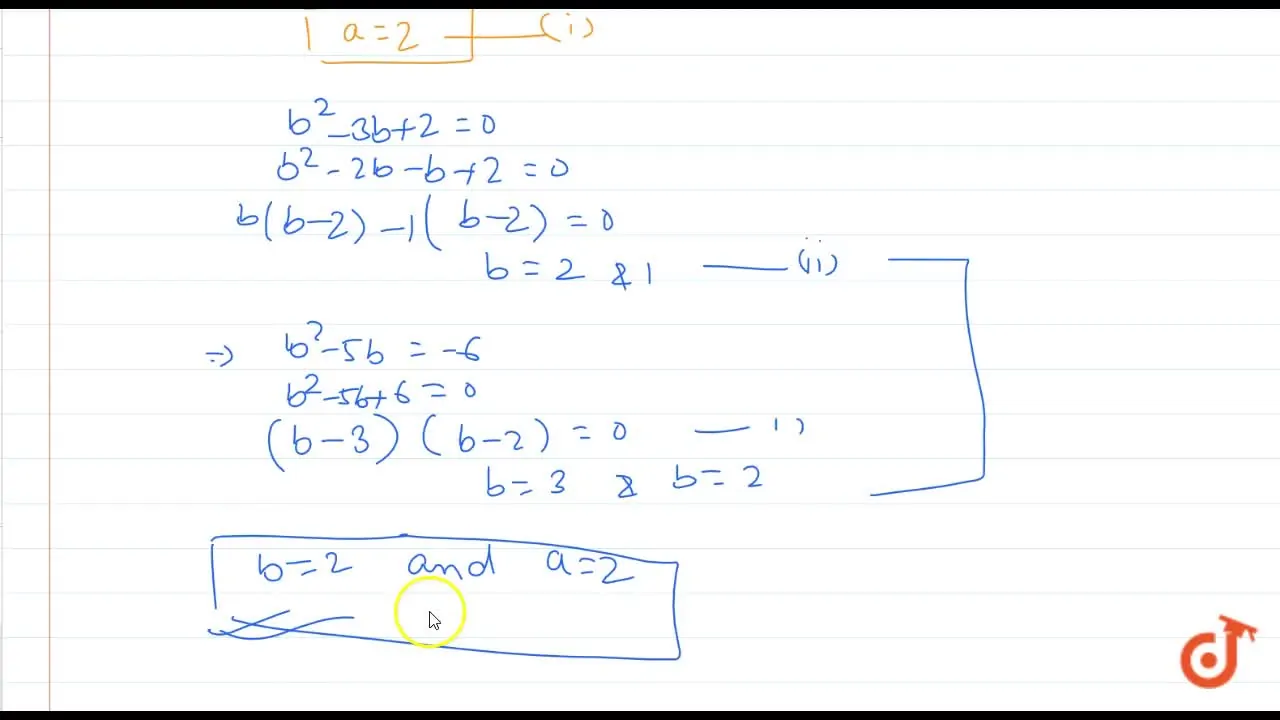
Find the values of a and b if A=B, where A=[a+43b8−6],B=[2a+2b2+28b2−56]
Video Solution
Text Solution
As the given matrices A and B are equal, therefore, their corresponding elements must be equal. Comparing the corresponding elements, we get
a+4=2a+2
8=8
3b=b2+2
−6=b2−5b
On simplifying, we get
a=2 and the common value of b=2.
Hence, the values of a and b are 2,2 .
|
Updated on:21/07/2023
Related Playlists
Similar Questions
Find the values of a and b if A=B , where A=[a+43b8−6] , B=[2a+2b2+28b2−56]
View SolutionFind the values of a and b, if A=B,where
A=[a+43b8−6]andB=[2a+2b2+28b2−5b]View SolutionIf a:b=3:8, find the value of (5a−3b)/(2a+b). (a) 9/14 (b) 14/9 (c) - 9/14 (d) -14/9
View SolutionFind a matrix X of order 3×2 such that 2A+3X=58B, where A=⎡⎢⎣804−236⎤⎥⎦,B=⎡⎢⎣2−242−51⎤⎥⎦
View SolutionIf a=2and b=8, then find the value of
ba+9View SolutionFind the matrix X such that A+3B+X=0, where A=[2658] and B=[12−23]
View Solutionif [a+43b8−6]=[2a+2b+28a−4b], then find the value of a−2b is
View SolutionFind matrices A and B ,if 2A−B=[6−60−421] and A−2B=[328−21−7]
View SolutionFind Value of K, for which A =[K842K] is a singular matrix is: .
View SolutionIf 2a+ba+4b=3 then find the value of a+ba+2b
View Solution