Find the shrotest distance between the lines →r=ˆi+ˆj+λ(2ˆi−ˆj+ˆk) and →r=2ˆi+ˆj−ˆk+μ(2ˆi−ˆj+ˆk).
Given lines are parallel
Here →a=ˆi+ˆj,→a2=2ˆi+ˆj+ˆk and →b=2ˆi−ˆj+ˆk
Now→a2−→a1=(2ˆi+ˆj−ˆk)−(ˆi+ˆj)=ˆi−ˆk
→b×(→a2−→a1)=∣∣
∣
∣∣ˆiˆjˆk2−1110−1∣∣
∣
∣∣
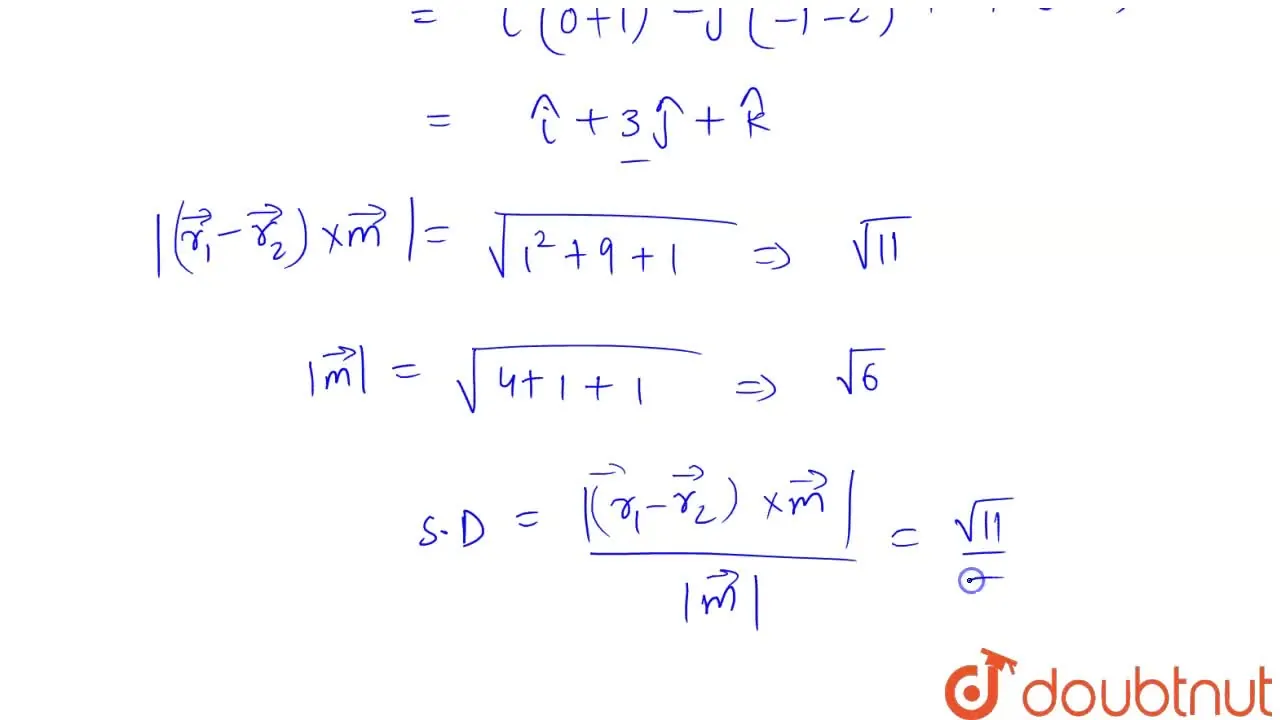
=ˆi(1−0)−ˆj(−2−1)+ˆk(0+1)
=ˆi+3ˆj+ˆk
∣∣→b×(→a2−→a1)∣∣=√1+9+1=√11
∣∣→b∣∣=√4+1+1=√16
Shortest distance betwene the parallel lines
=∣∣
∣∣→b×(→a2−→a1)→b∣∣
∣∣=√116=√666 units.
Related Playlists
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 11 A22videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - Exercise 11 B30videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Miscellaneous Exercise19videos
RELATIONS AND FUNCTIONS
- BOOK - NAGEEN PRAKASHAN ENGLISHCHAPTER - VECTORSEXERCISE - Miscellaneous Exercise19videos
VECTORS
Similar Questions
Find the shortest distance between the lines →r=ˆi+ˆj+λ(2ˆi−ˆj+ˆk) and →r=(2ˆi+ˆj−ˆk)+μ(3ˆi−5ˆj+2ˆk)
View SolutionFind the shortest distance between the lines →r=3ˆi+5ˆj+7ˆk+λ(ˆi−2ˆj+ˆk)and→r=−ˆi+ˆj−ˆk+μ(2ˆi−6ˆj+ˆk)
View SolutionFind the shortest distance between the lines →r=(ˆi+2ˆj+ˆk)+λ(2ˆi+ˆj+2ˆk)and→r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk).
View SolutionFind the shortest distance between the lines →r=(ˆii+2ˆj+ˆk)+λ(2ˆi+ˆj=2ˆk)and→r=2ˆi−ˆj−ˆk+μ(2ˆi+ˆj+2ˆk).
View SolutionFind the shortest distance between the lines
→r=ˆi+ˆj+ˆk+λ(3ˆi−ˆj)and→r=4ˆi−ˆk+μ(2ˆi+3ˆk)View SolutionShortest distance between the lines:→r=(4ˆi−ˆj)+λ(ˆi+2ˆj−3ˆk) and →r=(ˆi−ˆj+2ˆk)+u(2ˆi+4ˆj−5ˆk)
View SolutionFind the shortest distance between lines →r=6ˆi+2ˆj+2ˆk+λ(ˆi−2ˆj+2ˆk) and →r=−4ˆi−ˆk+μ(3ˆi−2ˆj−2ˆk).
View SolutionIf d is the shortest distance between the lines →r=(3ˆi+5ˆj+7ˆk)+λ(ˆi+2ˆj+ˆk) and
→r=(−ˆi−ˆj−ˆk)+μ(7ˆi−6ˆj+ˆk) then 125d2 is equal to ____________.View SolutionFind the angle between the lines
→r=(ˆi+ˆj)+λ(ˆi+ˆj+ˆk)and→r=(2ˆi−ˆj)+t(2ˆi+3ˆj+ˆk)View SolutionFind the shortest distance between the following pair of line: →r=ˆi+ˆj+λ(2ˆi−ˆj+2ˆk),→r=2ˆi+ˆj−ˆk+μ(3ˆi−5ˆj+2ˆk)
View Solution