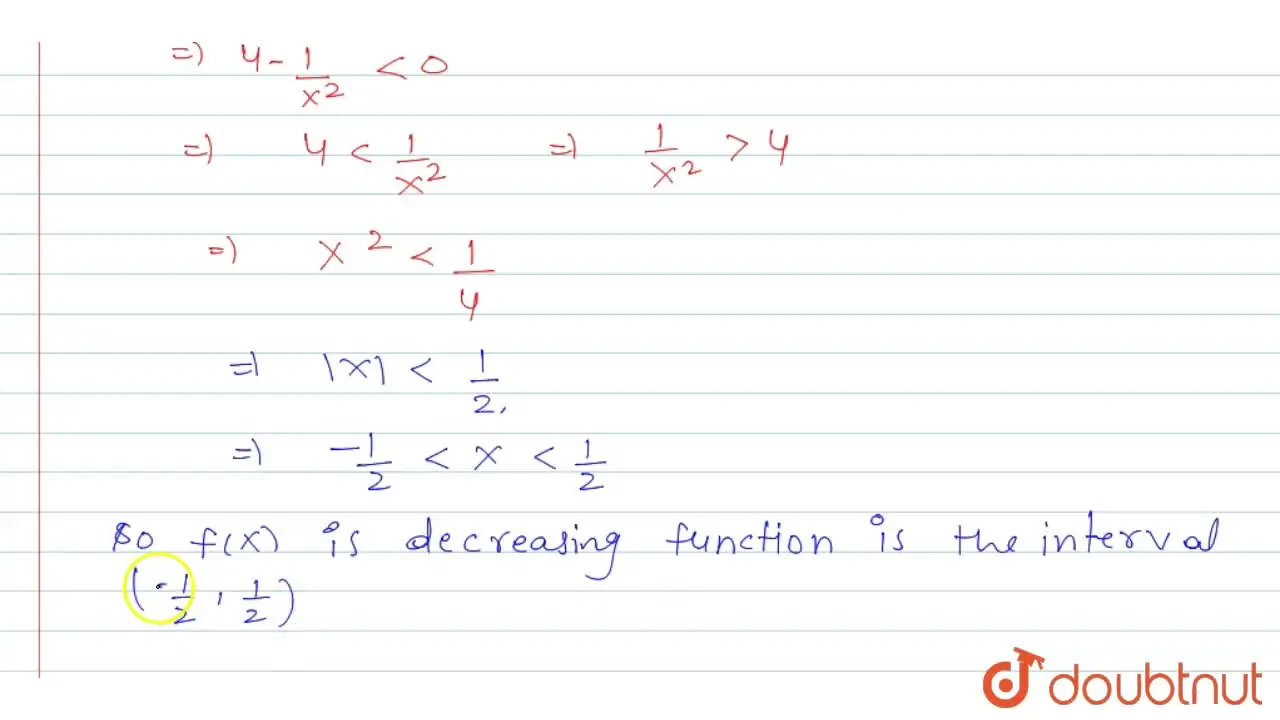
find the interval(s) in which the function f(x)=4x2+1x, is decreasing.
The correct Answer is:(−12,12)
Step by step video, text & image solution for find the interval(s) in which the function f(x)= (4x^(2)+1)/(x), is decreasing. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ICSECHAPTER - MODEL TEST PAPER-11EXERCISE - SECTION-B10videos
MODEL TEST PAPER-11
- BOOK - ICSECHAPTER - MODEL TEST PAPER-11EXERCISE - SECTION-C9videos
MODEL TEST PAPER-11
- BOOK - ICSECHAPTER - MODEL TEST PAPER 20 EXERCISE - SECTION C10videos
MODEL TEST PAPER 20
- BOOK - ICSECHAPTER - MODEL TEST PAPER-12 EXERCISE - SECTION-C10videos
MODEL TEST PAPER-12
Similar Questions
Find the intervals in which the function f(x)=log(1+x)−2x2+x is increasing or decreasing.
View SolutionFind the interval in which the function f(x) =2 log (x-2)−x2+4x+1 is increasing .
View SolutionFind the interval in which the function f(x)=x2+2x,x<0 is decreasing.
View SolutionDetermine the intervals in which the function f(x)=x4−8x3+22x2−24x+21 is decreasing or increasing.
View SolutionDetermine the intervals in which the function f(x)=x4−8x3+22x2−24x+21 is decreasing or increasing.
View SolutionFind the intervals in which the function f(x)=x4−x33 is increasing or decreasing.
View SolutionFind the intervals in which the function f(x)=x4−x33 is increasing or decreasing.
View SolutionThe interval on which the function f(x)=2x3+9x2+12x−1 is decreasing is
View SolutionFind the intervals in which the function f(x)=2x3+9x2+12x+20 is (i) increasing (ii) decreasing
View SolutionFind the intervals in which the function f(x)=2x3−9x2+12x+15 is increasing and decreasing.
View Solution