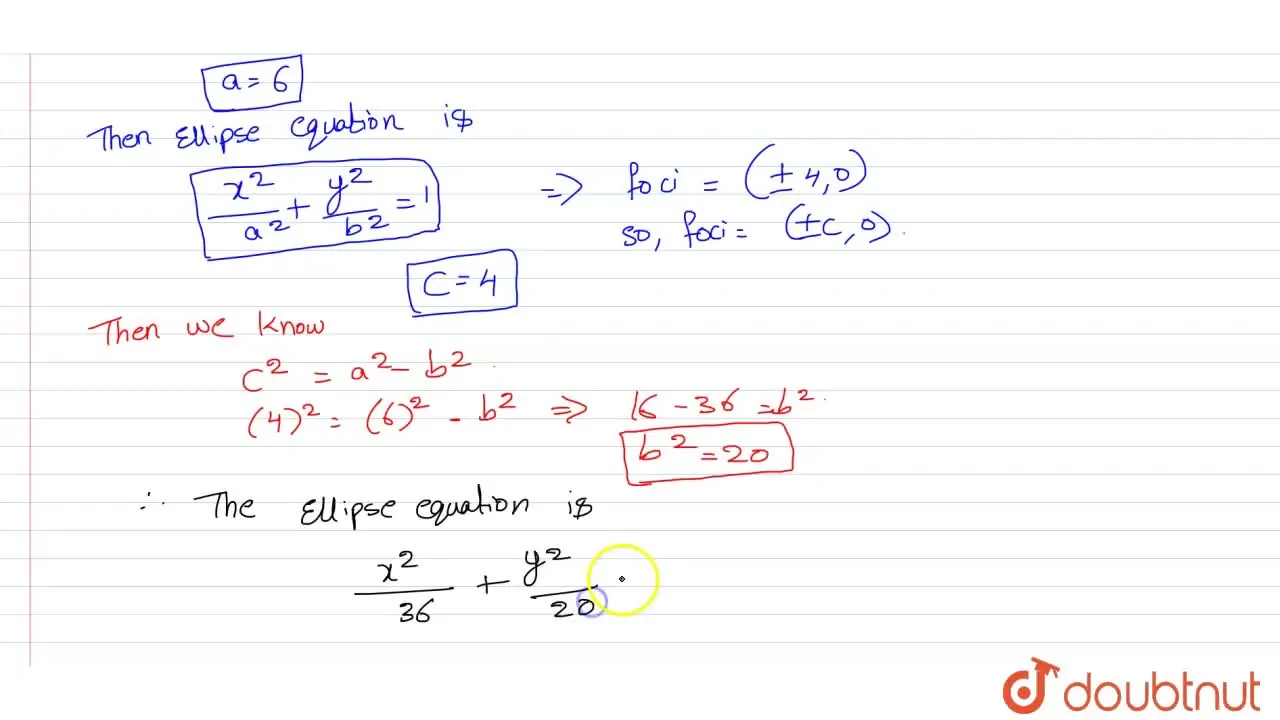
Find the equation of the ellipse whose vetices are (±6,0) and foci are (±4,0).
Equation of ellipse can be given as
x2a2+y2b2=1
Here, Foci of ellipse =(±4,0)
So, X-axis is major axis and c=4
Vertices of ellipse =(±6,0)
∴a=6⇒a2=36
∴b2=a2−c2=36−16=20
So, equation of required ellipse will be,
x236+y220=1.
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - SOLVED EXAMPLES19videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - MISCELLANEOUS EXERCISE9videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - EXERCISE 11.414videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions75videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - NCERT ENGLISHCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - EXERCISE 12.14videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
Find the equation of the ellipse whose vertices are (±2,0) and foci are (±1,0).
View SolutionFind the equation of the ellipse whose vertices are (0,±4) and foci are (0,±3).
View SolutionFind the equation of ellipse whose vetices are (5,0) and foci are (±4,0)
View SolutionFind the equation of the ellipse whose vertices are (±13,0)and foci are (±5,0).
View SolutionFind the equation of ellipse whose vertices are (0,±13) and foci (0,±5).
View SolutionFind the equation of the ellipse whose foci are (0±3) and e=34
View SolutionFind the equation of the hyperbola whose vertices are (±3,0) and foci at (±5,0)
View SolutionFind the equation of hyperbola whose vertices are (0,±5) and focii are (0,±10)
View SolutionFind the equation of ellipse having vertices at (±5,0) and foci (±4,0).
View SolutionFind the equation of the ellipse whose major axis is 12 and foci are (±4,0).
View Solution