find the equation of hyperabola where foci are (0,12) and (0,-12)and the length of the latus rectum is 36
Here, Foci of hyperbola =(0,±12)
That means the transverse axis of the hyperbola is Y-axis.
So, the equation will be of the type,
y2a2−x2b2=1→(1)
Also, c=12
Length of latus rectum =36
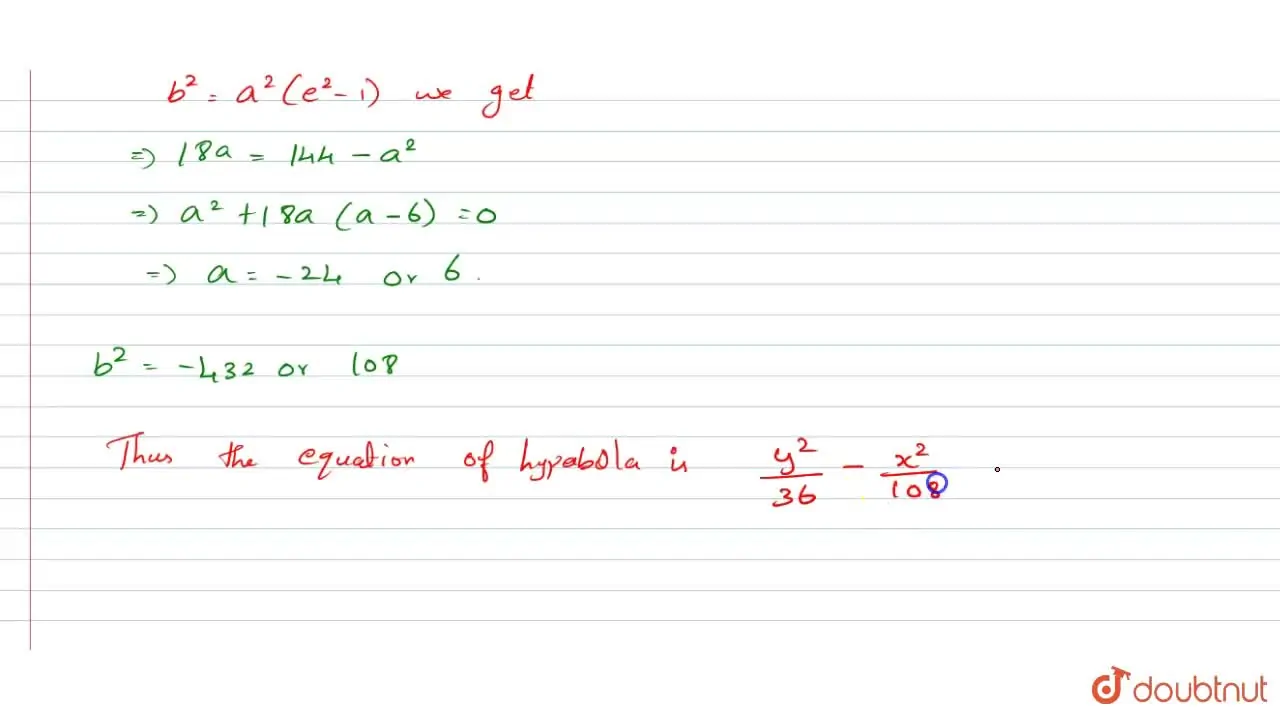
∴2b2a=36⇒b2=18a
In a hyperbola, c2=a2+b2
Putting value of c and b2,
∴122=a2+18a
⇒a2+18a−144=0
⇒a2+24a−6a−144=0
⇒(a+24)(a−6)=0
∴a=−24anda=6
But, a can not be negative, so,
a=6⇒a2=36
b2=18a=18∗6=108
Putting values of a2 and b2 in (1),
y236−x2108=1
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - MISCELLANEOUS EXERCISE9videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - EXERCISE 11.115videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - EXERCISE 11.320videos
CONIC SECTIONS
- BOOK - NCERT ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions75videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - NCERT ENGLISHCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - EXERCISE 12.14videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
The equation of the hyperbola whose foci are (±5,0) and length of the latus rectum is 92 is
View SolutionFind the equation of the hyperbola with foci at (±7,0) and length of the latus rectum as 9.6 units.
View SolutionFind the equation of hyperbola with foci at the points (−3,5) and (5,5) and length of latus rectum =2√8 units.
View SolutionThe equation of the hyperbola whose foci are (±4,0) and length of latus rectum is 12 is
View SolutionFind the equation of the hyperbola whose foci are (0,±4) and latus rectum is 12.
View SolutionFind the equation of parabola whose focus is (4,5) and vertex is (3,6). Also find the length of the latus rectum.
View SolutionFind the ellipse if its foci are (±2,0) and the length of the latus rectum is 103.
View SolutionThe equation of the latus rectum of a parabola is x+y=8 and the equation of the tangent at the vertex is x+y=12. Then find the length of the latus rectum.
View SolutionThe equation of the latus rectum of a parabola is x+y=8 and the equation of the tangent at the vertex is x+y=12. Then find the length of the latus rectum.
View SolutionFind the equation of the hyperbola whose foci are (±3,√5,0) and latus rectum is of length 8.
View Solution