Find the domain and the range of the function, f(x)=x−2x−3.
Video Solution
Text Solution
We have, f(x)=x−2x−3
Clearly, f(x) is defined for all real values of x for which x−3≠0, i.e., x≠3.
∴dom (f)=R−{3}.
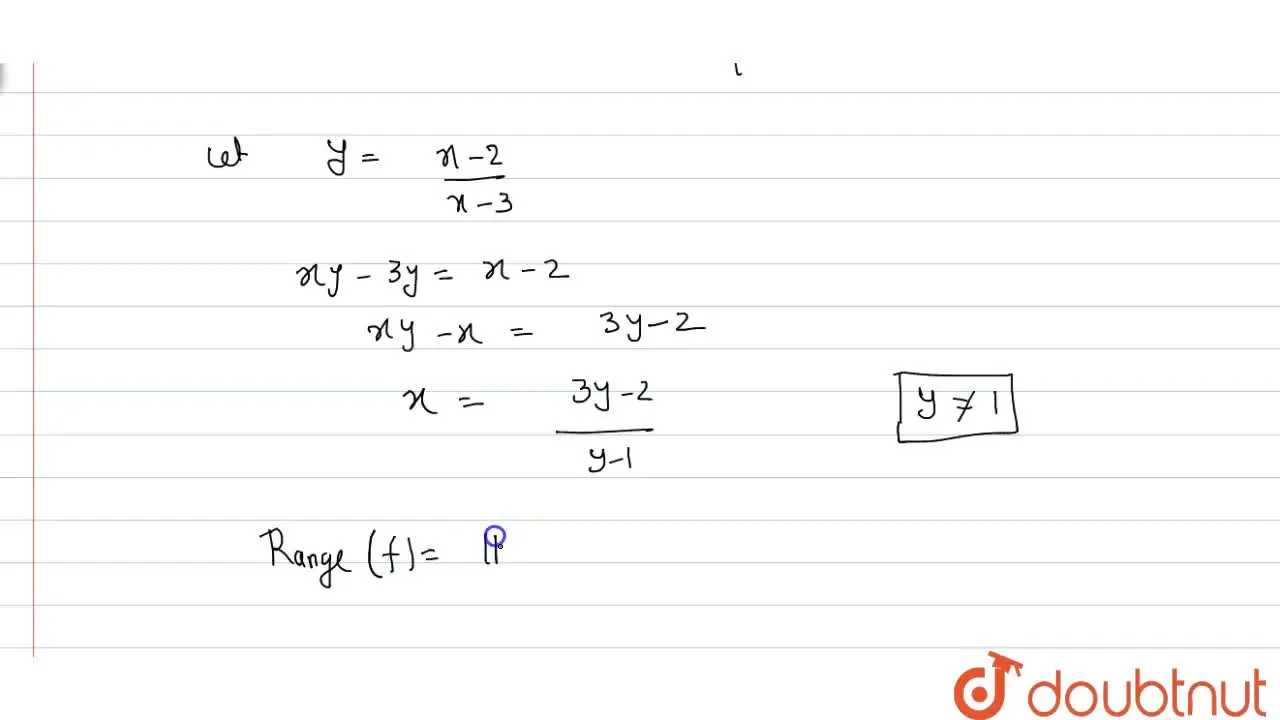
Let y=f(x). Then,
y=x−2x−3⇒xy−3y=x−2
⇒x(y−1)=3y−2
⇒x=3y−2y−1. ....(i)
It follows from (i) that x assumes real values for all y except that for which y-1=0, i.e., y=1.
∴range (f)=R−{1}. Hence, dom (f)=R−{3}and range (f)=R−{1}.
|
Updated on:21/07/2023
Related Playlists
- BOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - SOLVED EXAMPLES20videos
FUNCTIONS
- BOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - EXERCISE 3A20videos
FUNCTIONS
- BOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - EXERCISE 3F VERY-SHORT-ANSWER QUESTIONS21videos
FUNCTIONS
- BOOK - RS AGGARWALCHAPTER - ELLIPSEEXERCISE - EXERCISE26videos
ELLIPSE
- BOOK - RS AGGARWALCHAPTER - GEOMETRICAL PROGRESSIONEXERCISE - EXERCISE 12H (Very Short Answer Type Questions)13videos
GEOMETRICAL PROGRESSION
Similar Questions
Find the domain and the range of the function, f(x)=x2−25x−5.
View SolutionFind the domain and the range of the real function, f(x)=x−3x−5.
View SolutionFind the domain and range of the function f(x)=x2−9x−3
View SolutionFind the domain and the range of the real function, f(x)=1x+3.
View SolutionFind the domain and the range of the real function, f(x)=3−xx−3.
View SolutionFind the domain and range of the function f(x)=x|x|
View SolutionFind the domain and range of the function
f(x)=√x−1View SolutionFind the domain and range of the function f(x)=√x−5.
View SolutionFind the domain and the range of the real function, f(x)=|x−3|(x−3).
View SolutionFind the domain and range of the function f(x)=x1+x2
View Solution