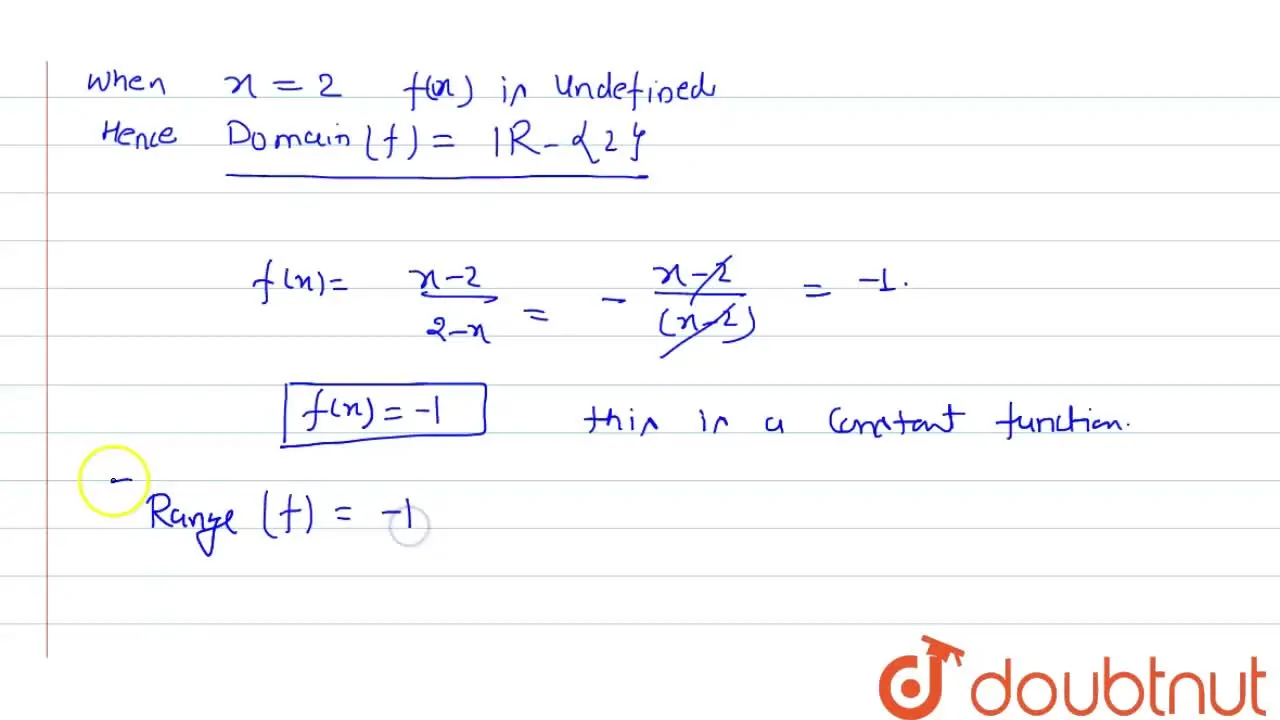
Find the domain and range of each of the following real valued function: f(x)=x−22−x .
Since, f(x) is defined for all real values of x, except for the case when 2–x=0 or x=2.
Domain (f)=R–{2}
Now, we have,
f(x)=x−22−x
f(x)=−2−x2−x=–1
When x≠2,f(x)=–1
∴ Range (f)={–1}
Related Playlists
Similar Questions
Find the domain and range of each of the following real valued function: f(x)=√x−3 .
View SolutionFind the domain and range of each of the following real valued function: f(x)=ax−bcx−d .
View SolutionFind the domain and range of each of the following real valued function: f(x)=ax+bbx−a .
View SolutionFind the domain of the range of each of the following real functions:
f(x)=3x−2x+2View SolutionFind the domain of the range of each of the following real functions:
f(x)=x−32−xView SolutionFind the domain of each of the following real valued function: f(x)=1x−7
View SolutionFind the domain of the range of each of the following real functions:
f(x)=√3x−5View SolutionFind the domain of each of the following real valued function: f(x)=2x−3x2−3x+2
View SolutionFind the domain of each of the following real valued function: f(x)=x−1x−3
View SolutionFind the domain of the range of each of the following real functions:
f(x)=1−|x−2|View Solution