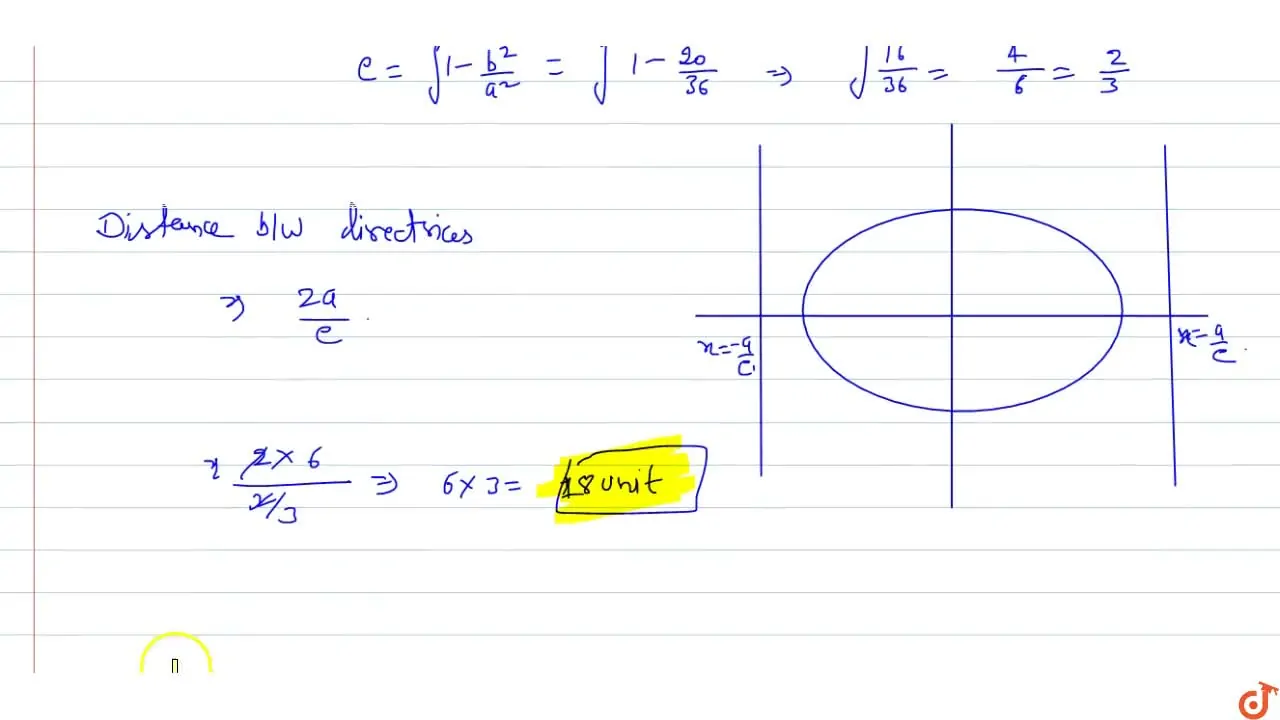
Find the distance between the directrices of the ellipse x236+y220=1.
x236+y220=1
a2=36,b2=20
a=±6,b=±2√5
e=√36−206=23
e=23
Distance between directrics=6/(2/3)=9.
Related Playlists
Similar Questions
If the distance between the foci and the distance between the directrices of the hyperbola x2a2−y2b2=1 are in the ratio 3:2,then a:b is
View SolutionFind the eccentricity of the ellipse x24=y225=1.
View SolutionIf the distance between the foci and the distance between the directrices of the hyperbola x2a2−y2b2=1 are in the ratio 3:2
View SolutionFind the eccentricity of the ellipse x236+y216=1.
View SolutionDistance between the directrices of the hyperbola x249−y216 =1 is
View SolutionFind the eccentricity of the ellipse x2169+y2144=1.
View SolutionThe distance between the directrices of the ellipse (4x−8)2+16y2=(x+√3y+10)2 is k, then k2 is
View SolutionFind the eccentricity of the ellipse x249+y236=1.
View SolutionIf the distance between the parallel tangents to the ellipse x24+y21=1 is 3 units then the slope is
View SolutionFind the equation of the directrices of the ellipse x236+y216=1
View Solution