Find the coordinates of the points which divides the line segment joining the points (6, 3) and (−4, 5) in the ratio 3 :2 internally.
(i) Given points are A (6,3) and B(-4,5). Let poitn P(x,y) divide AB internally in the ratio 3:2

∴(x,y)≡(3(−4)+2(6)3+2,3(5)+2(3)3+2)≡(0,215)
(ii) P(x,y) divides AB externally in the ratio 3:2.
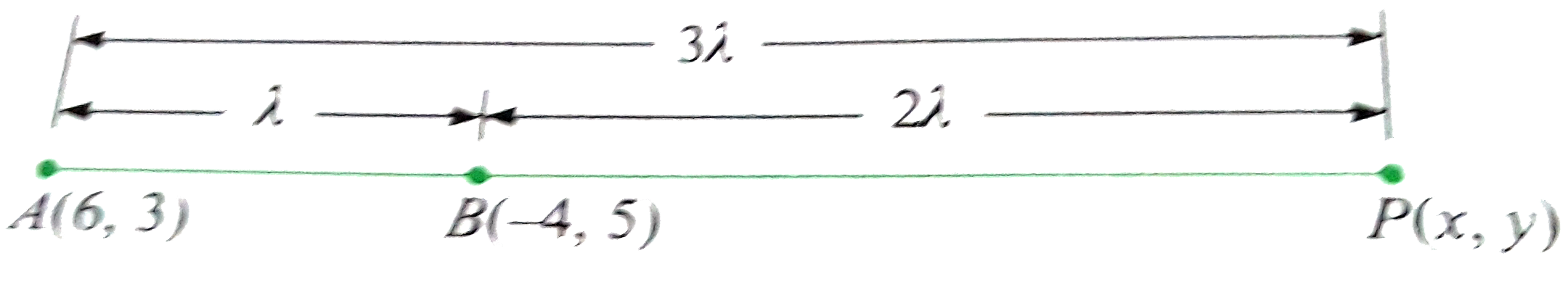
∴(x,y)≡(3(−4)−2(6)3−2,3(5)−2(3)3−2)≡(−24,9)
Alternatively, ABBP=(12) (From the figure)
∴(−4,5)≡(3(6)+1(x)2+1,2(3)+1(y)2+1)≡(12+x3,6+y3)
∴(x,y)≡(−24,9)
Topper's Solved these Questions
Similar Questions
Find the co ordinates of the point which divides the line segment joining the points (6, 3) and (-4, 5) in the ratio 3 : 2 internally
View SolutionFind the coordinates of the point which divides the line segment joining the points (4, 3) and (8, 5)in the ratio 3 : 1 internally.
View SolutionFind the coordinates of the points which trisect the line segment joining the points (2,3)and(6,5).
View SolutionFind the coordinates of the point which divies the line segment joining the points (1, 3, 5) and
(-2, 1, 0) in the ratio 1 : 2 internally.View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segments joining the points (6,3) and (−4,5) in the ratio 3:2 (i) internally and (ii) externally.
View SolutionFind the coordinates of the point which divides the line segment joining the points (1, 5, 3) and
(-2, 3, 4) in the ration 3:4 (i) internally, and (ii) externally.View SolutionFind the coordinates of the point which divides the line segment joining the point, (−2,3,5) and (1,−4,6) in the ratio.
2 : 3 internally,View SolutionFind the coordinates of the point which divides the line segment joining the points (5,−2)and(9,6) internally in the ratio3 : 1
View SolutionFind the coordinates of the point which divides the line segment joining the points (2,3,5)and (1,4,6)in the ratio (i) 2:3internally, (ii) 2:3externally.
View Solution
