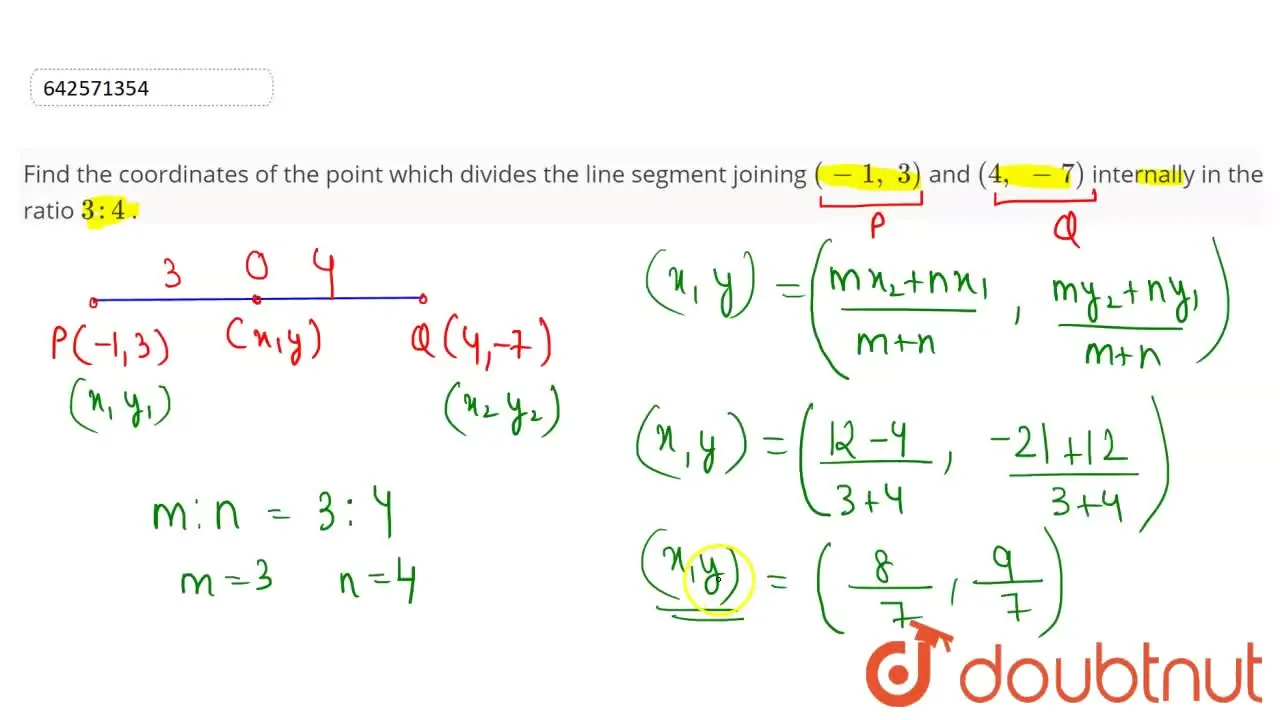
Find the coordinates of the point which divides the line segment joining (−1, 3) and (4, −7) internally in the ratio 3:4 .
Step by step video & image solution for Find the coordinates of the point which divides the line segment joining (-1,\ 3) and (4,\ -7) internally in the ratio 3:4 . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
Similar Questions
Find the coordinates of the point which divides the line segment joining the points (5,−2)and(9,6) internally in the ratio3 : 1
View SolutionFind the coordinates of points which trisect the line segment joining (1,−2)and(−3,4).
View SolutionFind the coordinates of the points which divides the line segment joining the points (6, 3) and (−4, 5) in the ratio 3 :2 internally.
View SolutionFind the co ordinates of the point which divides the line segment joining the points (6, 3) and (-4, 5) in the ratio 3 : 2 internally
View SolutionFind the point which divides the line segment joining
(-1,2), (4,-5) in the ratio 3 : 2View SolutionFind the co-ordinates of a point which divides the line segment joining P(5, 4, 2) and Q(-1, -2, 4) in the ratio 2 : 3.
View SolutionFind the coordinates of the points of trisection of the line segment joining (4, −1) and (−2, −3).
View SolutionWrite the coordinates of the point dividing line segment joining points (2, 3) and (3, 4) internally in the ratio 1 :5 .
View SolutionFind the coordinates of the point which divides the line segment joining the points (4, 3) and (8, 5)in the ratio 3 : 1 internally.
View SolutionFind the coordinates of the point which divides the line segment joining (2,4) and (6,8) in the ratio1:3 internally and externally.
View Solution