Find the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
Given curves arey2=4x ....(1)
4x2+4y2=9
...(2)
⇒x2+y2=94
Center of circle is (0,0) and radius of circle is 3/2
Putting the value from equation (1) in equation (2),
4x2+16x−9=0
⇒x=12,−92
So, x=12
⇒y=±√2
So, the points of intersection of both the curves are (12,√2)and(12,−√2).
Since, the area OABCO is symmetrical about x-axis.
∴Area OABCO=2×Area OBC
Area OBCO=Area OMC+Area MBC
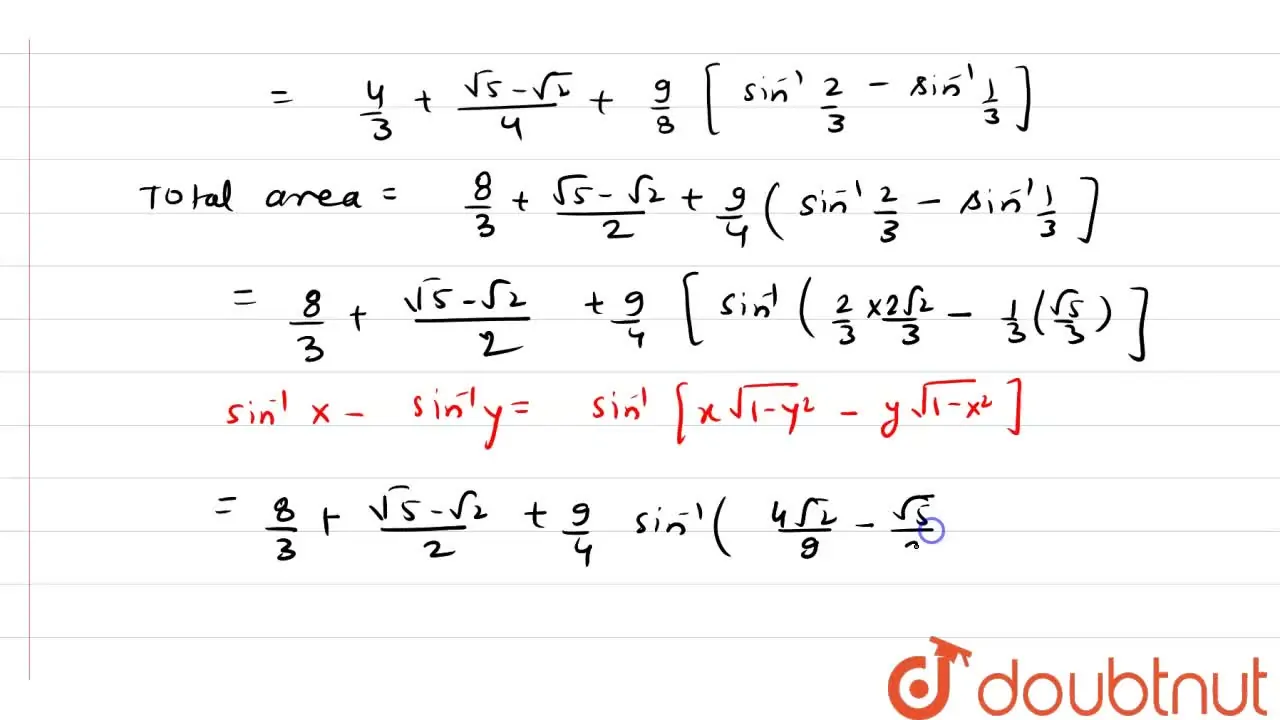
=∫1202√xdx+∫321212√9−4x2dx
=2∫120x3232+∫3212
⎷(32)2−x2dx
Required Area =√23+9π16−√24−98sin−113
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - SOLVED EXAMPLES15videos
APPLICATION OF INTEGRALS
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Exercise 8.113videos
APPLICATION OF INTEGRALS
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - SOLVED EXAMPLES51videos
APPLICATION OF DERIVATIVES
- BOOK - NCERT ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5.717videos
CONTINUITY AND DIFFERENTIABILITY
Similar Questions
Find the areas of the region {(x,y):y2≤4x,4x2+4y2≤9}, using integration.
View SolutionUsing integration ,find the area of the region {(x,y):y2≤4x,4x2+4y2≤9}
View SolutionFind the area of the region {(x,y):x2≤y≤|x|}.
View SolutionFind the area of the region {(x,y) :x2+y2≤4, x+y ≥2}
View SolutionFind the area of the region {(x,y) :x2+y2≤4, x+y ≥2}
View SolutionFind the area of the region {(x,y):x2+y2≤1≤x+y}.
View SolutionUsing integration find the area of the region {(x,y):x2+y2≤2ax,y2≥ax,x,y≥0}.
View SolutionDraw a rough sketch of the region {(x,y):y2≤5x, 5x2+5y2≤36} and find the area enclosed by the region using method of integration
View SolutionFind the area of the region {(x, y):x2a2+y2b2≤1≤xa+yb}
View SolutionFind the area of the region {(x,y):x2+y2≤4,x+y≥2}, using the method of integration.
View Solution