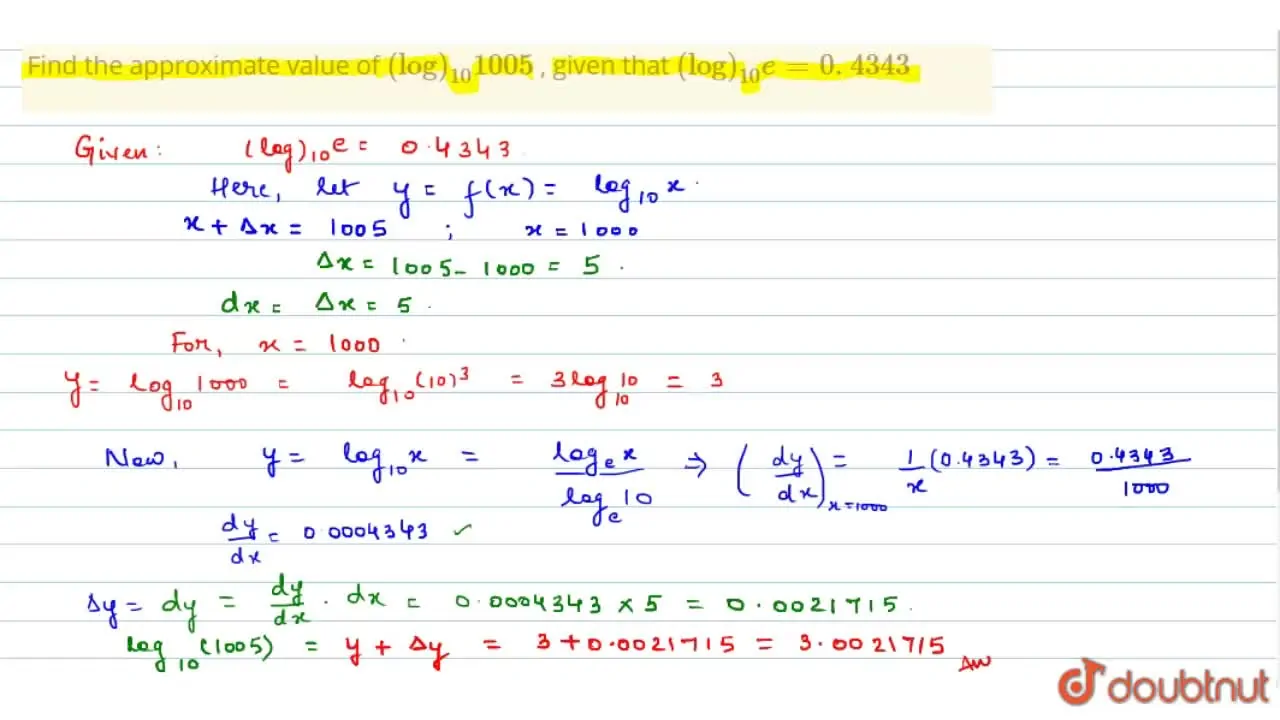
Find the approximate value of (log)101005 , given that (log)10e=0.4343
Here, we will use the following rule,
f(x+Δx)=f(x)+f'(x)Δx
Here, f(x+Δx)=log101005,f(x)=log10x,x=1000,Δx=5
∴log10(x+Δx)=log10x+ddx(log10x)Δx
∴log(x+Δx)=log10x+ddx(logexloge10)Δx
⇒log10(1005)=log10(1000)+1loge10(1x)(5)
=3+log10e(11000)∗5
=3+0.4343∗11000∗5
=3+0.0021
=3.0021
∴log10(1005)=3.0021
Related Playlists
Similar Questions
Using differentials, find the approximate value of (log)1010.1 , it being given that (log)10e=0.4343 .
View SolutionUsing differentials, find the approximate value of log1010.1 when log10e=0.4343.
View SolutionUse differentials to find the approximate value of (log)e(4.01), having given that (log)e4=1.3863 .
View SolutionUse differentials to find the approximate value of (log)e(4.01), having given that (log)e4=1.3863 .
View SolutionUsing differentials, find the approximate value of (log)e4.04 , it being given that (log)104=0.6021 and (log)10e=0.4343 .
View SolutionUsing differentials, find the approximate value of (log)e10.02 , it being given that (log)e10=2.3026 .
View SolutionUsing differentials, find the approximate value of loge (4.01), given that loge4=1.3863.
View SolutionFind the value of :
log100.001View SolutionFind the value of :
log50.2View SolutionFind the value of :
log2,0.125View Solution