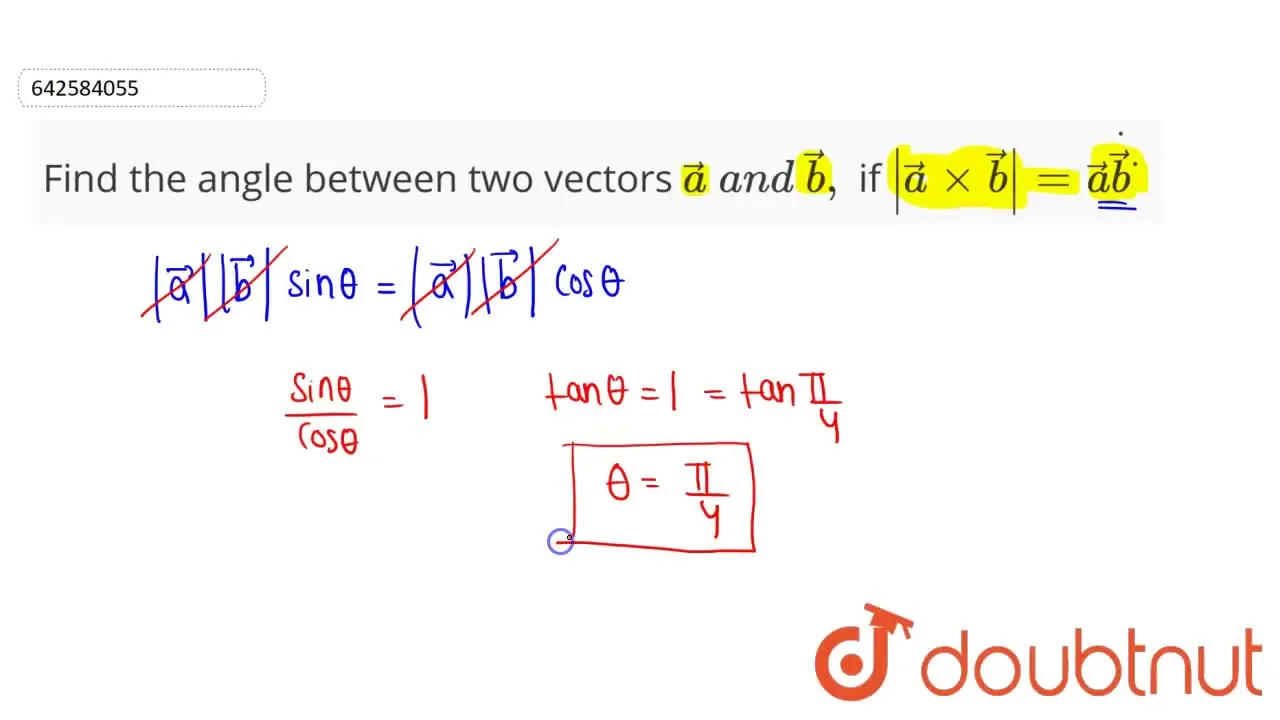
Find the angle between two vectors →a and →b, if ∣∣→a×→b∣∣=→a.→b.
Step by step video & image solution for Find the angle between two vectors vec a\ a n d\ vec b , if | vec axx vec b|= vec adot vec bdot by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Find the angle between two vectors →a and →b, if ∣∣→a×→b∣∣=→a.→b .
View SolutionFind the angle between two vectors →a and →b, if∣∣∣→a|=3, |→b∣=3 and →a.→b=1
View SolutionFor any two vectors →a and →b, fin d\ (→a×→b).→b.
View SolutionIf θ is the angle between any two vectors →a and →b , then →a.→b=∣∣→a×→b∣∣ when θ is equal to (a) 0 (B) π4 (C) π2 (d) π
View SolutionIf |→a|=10, ∣∣→b∣∣=2 and ∣∣→a×→b∣∣=16 find →a.→b.
View SolutionFind the angle between two vectors →a and →b with magnitudes 1 and 2 respectively and when ∣∣→a×→b∣∣=√3.
View SolutionIf |→a|=√26, ∣∣→b∣∣=7 and ∣∣→a×→b∣∣=35 ,find →a.→b.
View SolutionIf →a,→b,→c are unit vectors such that →a.→b=0=→a.→c and the angle between →b and →c is π3, then find the value of ∣∣→a×→b−→a×→c∣∣.
View SolutionWhat is the angle between vectors →a and →b with magnitudes 2 and 3 respectively ? Given →a.→b=√3 .
View SolutionFind ∣∣→a−→b∣∣,if two vector →aand →bare such that |→a|=2,∣∣→b∣∣=3and →a.→b=4.
View Solution