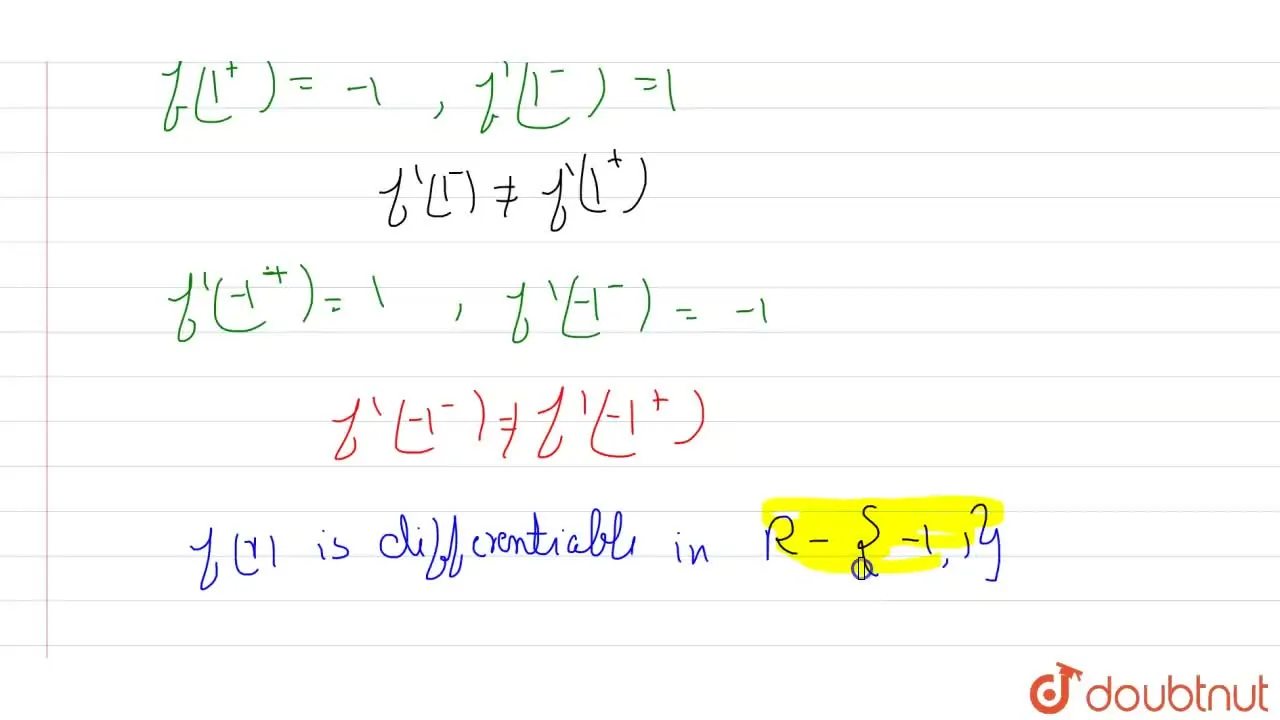
Discuss the differentiability of f(x)=sin−1(2x1+x2)
Step by step video & image solution for Discuss the differentiability of f(x) = sin^(-1)((2x)/(1+x^(2))) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Discuss the differentiability of f(x)=sin|x|
View SolutionDiscuss the differentiability of f(x=cos−1(1−x21+x2)
View SolutionDiscuss the differentiability of f(x)=x3atx=1
View SolutionDiscuss the differentiability of f(x)=|x−1|+|x−2|
View SolutionDiscuss the differentiability of f(x)=|x−1|+|x+1|
View SolutionDiscuss the differentiability of f(x)=∣∣∣∣x2−4∣∣−12∣∣.
View SolutionDiscuss the differentiability of f(x)={xsin(lnx2)x≠00x=0 at x=0
View SolutionDiscuss the differentiability of f(x)=⎧⎨⎩(x−e)2−2(1(e−x)),x≠e and 0 at x=e . at the point x=e
View SolutionDiscuss the differentiability of f(x)=|px[x]|,−1<x≤2
View SolutionDiscuss differentiability of f(x)=(e|x|−1−1)∣∣x4−x2∣∣.
View Solution