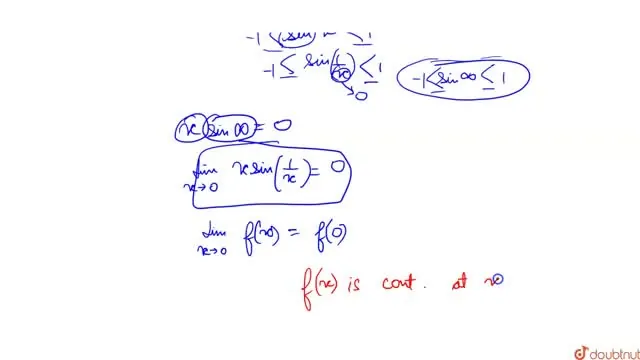
Discuss the continuity of the functions at the points shown against them . If a function is discontinuous , determine whether the discontinuity is removable . In this case , redefine the function , so that it becomes continuous : f(x)=xsin(1x) , for x≠0=0 , for x=0⎫⎬⎭ at x=0
f(0)=0 …(Given)…(1)
limx→0f(x)=limx→0xsin1x
Since −1≤sin1x≤1 asx≠0
∴−x≤xsin1x≤x
∴limx→0(−x)≤limx→0xsin1x≤limx→0(x)
∴0≤limx→0f(x)≤0
∴limx→0f(x)=0 ...(2)
From (1) and (2) , limx→0f(x)=f(0)
∴f is continuous at x=0.
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CONTINUITY EXERCISE - EXAMPLES FOR PRACTICE26videos
CONTINUITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CONTINUITY EXERCISE - MULTIPLE CHOICE QUESTIONS6videos
CONTINUITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - BINOMIAL DISTRIBUTIONEXERCISE - MULTIPLE CHOICE QUESTIONS8videos
BINOMIAL DISTRIBUTION
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - DEFINITE INTEGRALSEXERCISE - MULTIPLE CHOICE QUESTIONS10videos
DEFINITE INTEGRALS
Similar Questions
Discuss the continuity of the functions at the points shown against them . If a function is discontinuous , determine whether the discontinuity is removable . In this case , redefine the function , so that it becomes continuous :
F(x)=4x−ex6x−1 , for x≠0=log(23) , for x=0⎫⎪⎬⎪⎭ at x=0.View SolutionDiscuss the conjinuity of the functions at the points shown against them. If a function is discontinuous, determine whether the discontinuity is removable. In this case, redefine the function, so that it becomes continuous : f(x)=log(100(0.01+x))3x,forx≠0,forx=0}at=0.=1003
View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=e5x−e2xsin3x,forx≠0=1,forx=0}atx=0.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=1−cos3xxtanx,forx≠0=9,forx=0}atx=0.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=e5x−e2xsin3x,forx≠0=1,forx=0}atx=0.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)sinx−cosx,forx≠0=−1,forx=0}atx=0.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=log(2+x)−log(2−x)tanx,forx≠0=1,forx=0}atx=0.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=logx−log7x−7,forx≠7=7,forx=7}atx=7.View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous : f(x)=x2−4x√x2+9−5,forx≠4=3,forx=4⎫⎬⎭atx=4.
View SolutionDiscuss the continuity of the functions at the points given against them. If a function is discontinuous, determine whether the discontiunity is removable. In this case, redefine the function, so that it becomes continuous :
f(x)=(3sinx−1)2xlog(1+x),forx≠0=2log3,forx=0⎫⎪⎬⎪⎭atx=0.View Solution