Differentiate the following w.r.t. x:
sin(tan−1e−x)
Video Solution
Text Solution
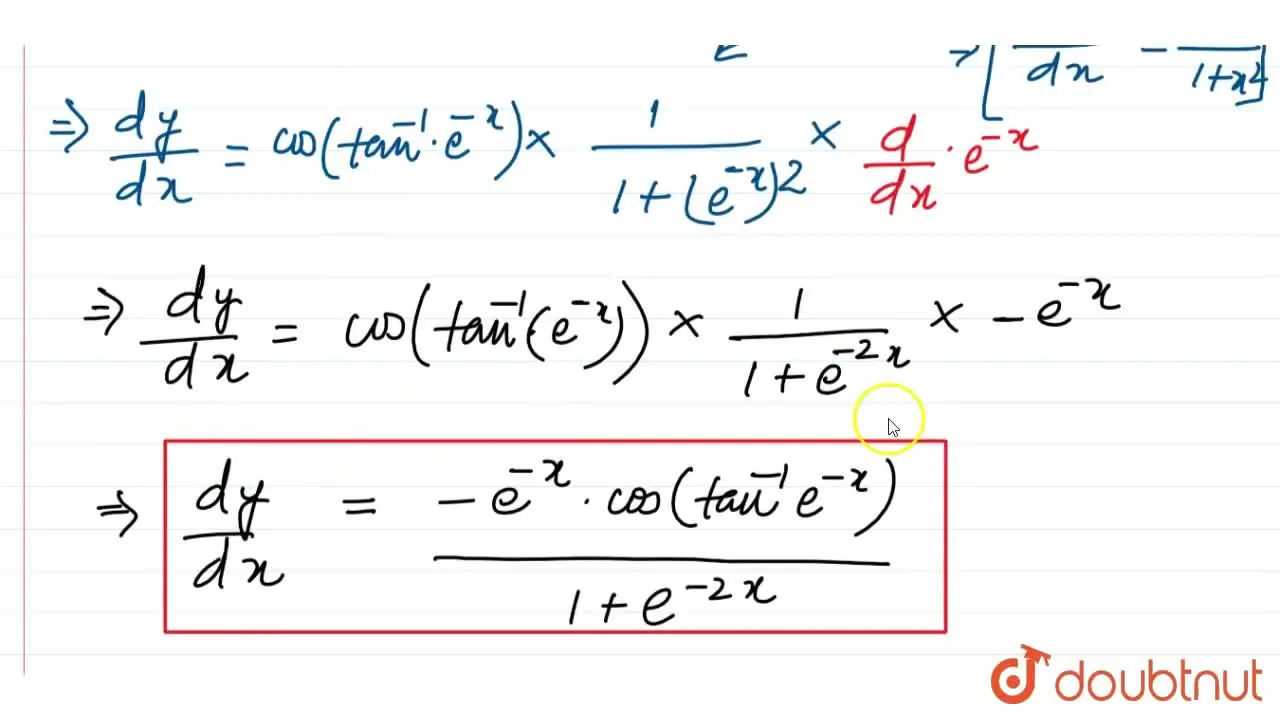
Let y=sin(tan−1)ex
Now, take differentiation on both sides w.r.t. x get
1√1−y2(dydx)=(11+(e−x)2)⋅ddx(e−x)
dydx=√1−y2×11+e−2x⋅(−e−x)
so, dydx=−√1−sin2(tan−1e−x)1+e−2x×e−x
or, dydx=−cos(tan−1e−x)1+e−2x×e−x
|
Updated on:21/07/2023
Related Playlists
- BOOK - NCERT ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5.315videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - NCERT ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5.86videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - NCERT ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 5.134videos
CONTINUITY AND DIFFERENTIABILITY
- BOOK - NCERT ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - EXERCISE 8.27videos
APPLICATION OF INTEGRALS
- BOOK - NCERT ENGLISHCHAPTER - DETERMINANTSEXERCISE - All Questions116videos
DETERMINANTS
Similar Questions
Differentiate the following w.r.t x
πeView SolutionDifferentiate the following w.r.t. x:
esin−1xView SolutionDifferentiate the following w.r.t x
π2View SolutionDifferentiate the following w.r.t x
2020View SolutionDifferentiate the following w.r.t.x.
xxView SolutionDifferentiate the following w.r.t.x. sin3x
View SolutionDifferentiate the following w.r.t x
e−5View SolutionDifferentiate the following w.r.t x
cos 3xView SolutionDifferentiate the following w.r.t x
x−4View SolutionDifferentiate the following w.r.t.x.
x√xView Solution