Differentiate tan−1(1−x1+x) with respect to √1−x2 , if −1<x<1 .
Video Solution
Text Solution
Let, u=tan−1(1−x1+x)
Put x=tanθ
⇒θ=tan−1x
⇒u=tan−1(1−tanθ1+tanθ)
⇒u=tan−1[tan(π4−θ)]...(i)
Here,
−1<x<1
⇒−1<tanθ<1
⇒−π4<θ<π4
⇒π4>−θ>π4
⇒−π4<−θ<π4
⇒0<π4−θ<π2
So, from equation (i),
u=π4−θ[ Since , tan−1(tanθ)=θ, if θ∈(−π2,π2)]
⇒u=π4−tan−1x
Differentiating it with respect to x,
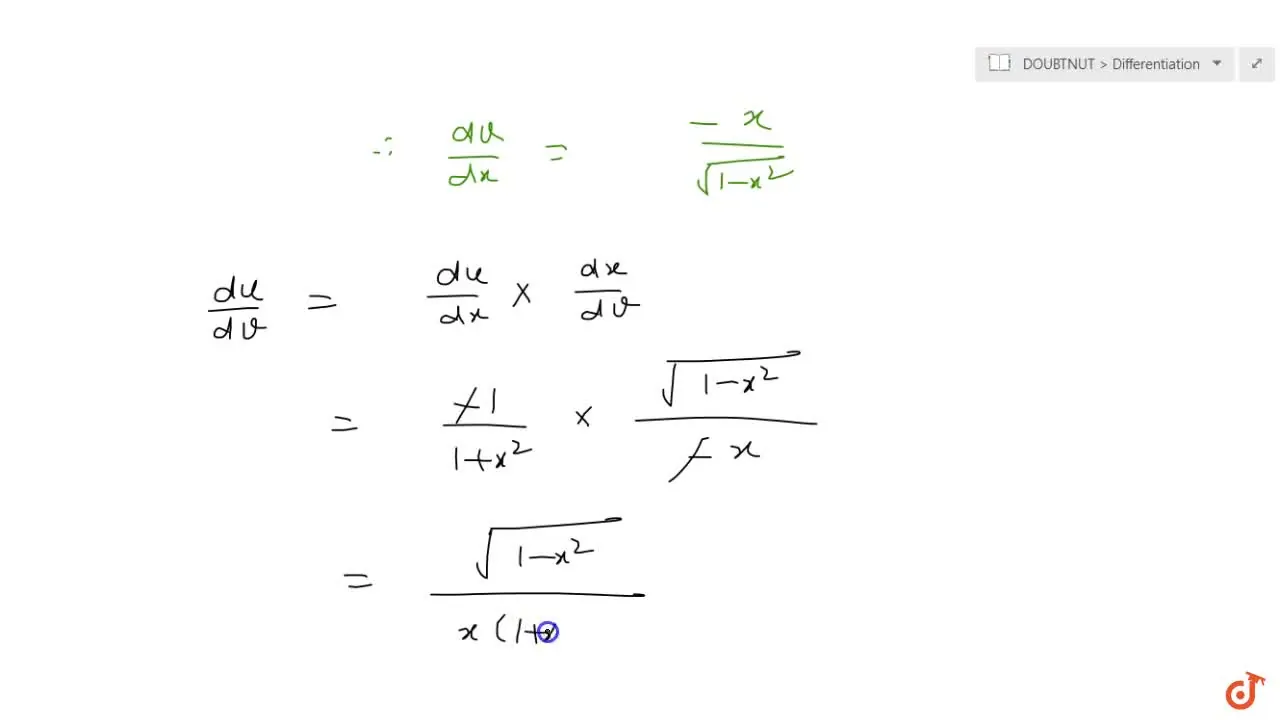
dudx=0−(11+x2)
⇒dudx=−11+x2...(ii)
And let, {v}=√1−x2
Differentiating it with respect to X,
dvdx=12√1−x2×ddx(1−x2)
⇒dvdx=12√1−x2(−2x)
⇒dvdx=−x√1−x2...(iii)
Dividing equation (ii)by (iii),
dudxdvdx=−11+x2×√1−x2−x
∴dudv=√1−x2x(1+x2)
|
Updated on:21/07/2023
Related Playlists
- BOOK - RD SHARMACHAPTER - DIFFERENTIALS, ERRORS AND APPROXIMATIONSEXERCISE - Solved Examples And Exercises102videos
DIFFERENTIALS, ERRORS AND APPROXIMATIONS
- BOOK - RD SHARMACHAPTER - DIRECTION COSINES AND DIRECTION RATIOSEXERCISE - Solved Examples And Exercises67videos
DIRECTION COSINES AND DIRECTION RATIOS
Similar Questions
Differentiate tan−1(a+x1−ax) with respect to x
View SolutionDifferentiate tan−1(x−ax+a) with respect to x
View SolutionDifferentiate tan−1(1+2x1−2x) with respect to √1+4x2 .
View SolutionDifferentiate tan−1(1+2x1−2x)withrespeco√1+4x2
View SolutionDifferentiate √tan−1(x2) with respect to x :
View SolutionDifferentiate tan−1(1+cosxsinx) with respect to x.
View SolutionDifferentiate tan−1(1+ax1−ax) with respect to √1+a2x2.
View SolutionDifferentiate tan−1(1+ax1−ax) with respect to √1+a2x2 .
View SolutionDifferentiate tan−1(11−x+x2)
View SolutionDifferentiate tan−1(2x+11−4x) with respect to x.
View Solution