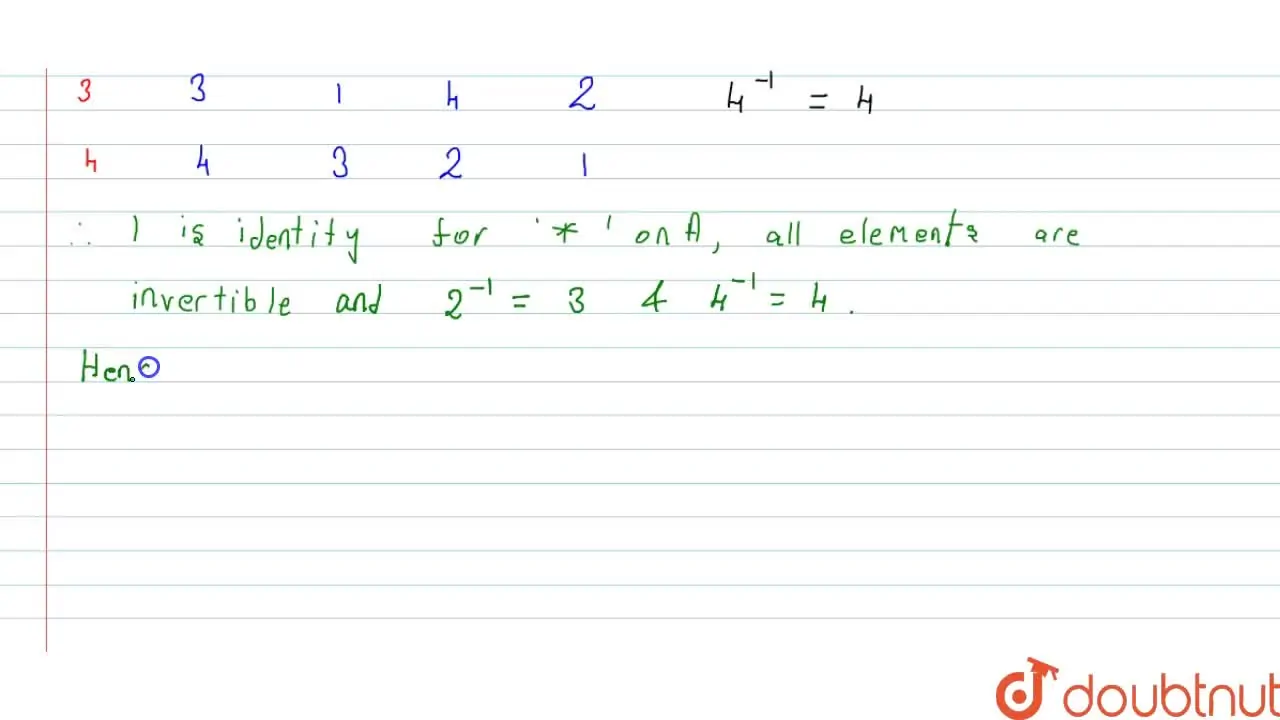
Define a binary operation ∗ on the set A={1, 2, 3,4} as a∗b=ab (mod 5) . Show that 1 is the identity for ∗ and all elements of the set A are invertible with 2−1=3 and 4−1=4.
Step by step video & image solution for Define a binary operation ** on the set A={1,\ 2,\ 3,4} as a**b=a b\ (mod\ 5) . Show that 1 is the identity for ** and all elements of the set A are invertible with 2^(-1)=3 and 4^(-1)=4. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Define a binary operation * on the set A={0, 1, 2, 3, 4, 5} as a * b=a+b(mod 6) Show that zero is the identity for this operation and each element a of the set is invertible with 6−a being the inverse of a.
View SolutionDefine a binary operation * on the set A={0,1,2,3,4,5} given by a∗b=ab(mod6). Show that 1 is the identity for *. 1 and 5 are the only invertible elements with 1−1=1 and 5−1=5
View SolutionDefine a binary operation * on the set A={0,1,2,3,4,5} as a⋅b=a+b (mod 6). Show that zero is the identity for this operation and each element a of the set is invertible with 6−a being the inverse of a. OR A binary operation * on the set {0,1,2,3,4,5} is defined as a⋅b={a+b,ifa+b<6a+b−6,ifa+b≥6 Show that zero is the identity for this operation and each element a of set is invertible with 6−a, being the inverse of a.
View SolutionDefine a binary operation * on the set A={0,1,2,3,4,5} as a⋅b=a+b (mod 6). Show that zero is the identity for this operation and each element a of the set is invertible with 6−a being the inverse of a. OR A binary operation * on the set {0,1,2,3,4,5} is defined as a⋅b={a+b,ifa+b<6a+b−6,ifa+b≥6 Show that zero is the identity for this operation and each element a of set is invertible with 6−a, being the inverse of a.
View SolutionDefine a binary operation * on the set A={0,1,2,3,4,5} given by a⋅b=ab (mod 6). Show that 1 is the identity for *. 1 and 5 are the only invertible elements with 1−1=1 and 5−1=5
View SolutionDefine a binary operation ∗ on the set {0,1,2,3,4,5} as a∗b={a+bifa+b<6; a+b−6,ifa+b≥6. Show that zero is the identity for this operation and each element a≠0of the set is invertible with 6−a being the inverse of a
View SolutionA binary operation * on the set {0,1,2,3,4,5} is defined as: a*b={a+b,a+b−6\ \ \ \ if\ a+b<6\ \ \ if a+b≥6 Show that zero is the identity for this operation and each element a of the set is invertible with 6-a, being the inverse of a.
View SolutionFor the binary operation ×7 on the set S={1, 2, 3, 4, 5, 6} , compute 3−1×74 .
View SolutionConsider the binary operation ∗ on the set {1,2,3,4,5} defined by a∗b=min.{a,b}. Write the operation table of the operation ∗.
View SolutionThe binary operation defined on the set z of all integers as a∗b=|a−b|−1 is
View Solution