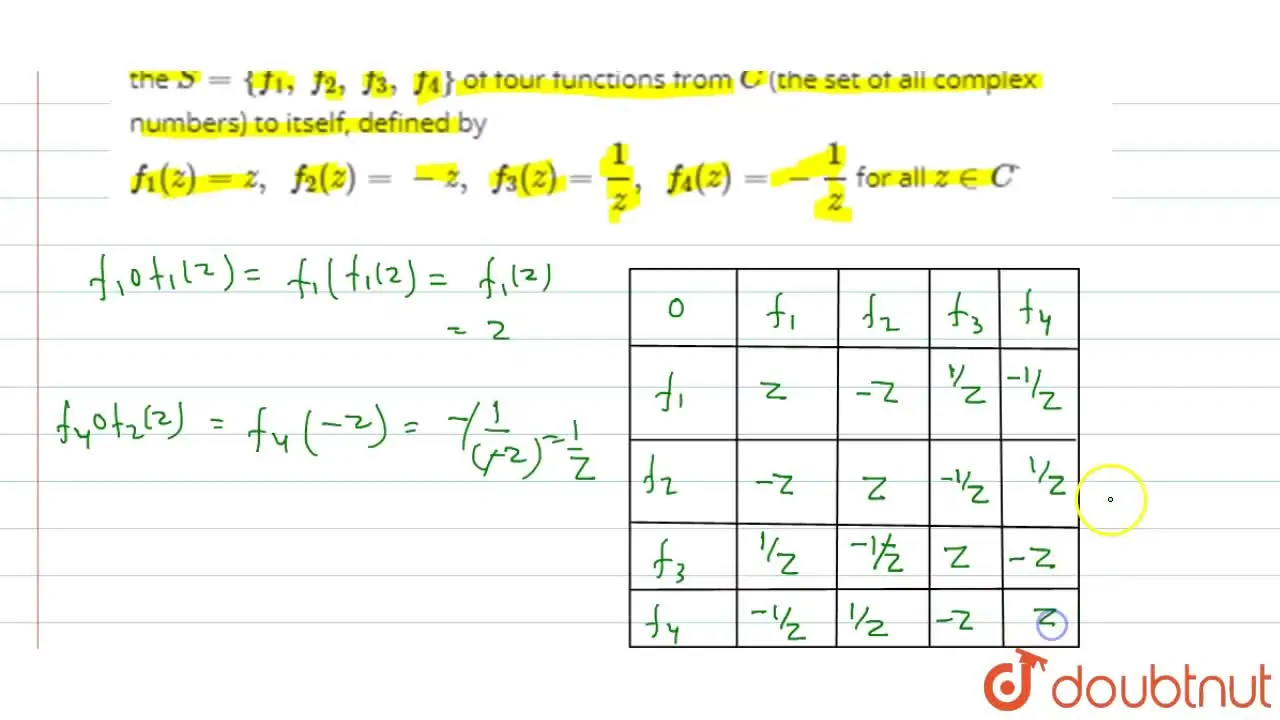
Construct the composition table for the composition of functions (o) defined on the S={f1, f2, f3, f4} of four functions from C (the set of all complex numbers) to itself, defined by f1(z)=z, f2(z)=−z, f3(z)=1z, f4(z)=−1z for all z∈C.
Video Solution
Text Solution
Given,
{f}1({z})={z},{f}2({z})=−{z}
{f}3({z})=1{z},{f}4({z})=−1{z}
f1∘f1(z)=f1[f1(z)=f1(z)]=z
⇒{f}1∘{f}1={f}1
{f}1∘{f}2({z})={f}1[{f}2({z})]
=f1(−z)=−z=f2(z)
⇒{f}1∘{f}2={f}2
{f}1∘{f}3({z})={f}1[{f}3({z})]
={f}1(1{z})=1{z}={f}3({z})
⇒{f}1∘{f}3={f}3
{f}1∘{f}4({z})={f}1[{f}4({z})]
=f1(−1z)=−1z=f4(z)
⇒{f}1∘{f}4={f}4
{f}2∘{f}1({z})={f}2[{f}1({z})]
=f2(z)=−z=f2(z)
⇒f2∘f1=f2
f2∘f2(z)=f2[f2(z)]
={f}2(−{z})={z}={f}1({z})
⇒{f}2∘{f}2={f}1
f2∘f3(z)=f2[f3(z)]
={f}2(1{z})
=−1z=f4(z)
⇒f2∘f3=f4
f2∘f4(z)=f2[f4(z)]
=f2(−1z)
=1z=f3(z)
⇒f2∘f4=f3
f3∘f1(z)=f3[f1(z)]=f3(z)
=1z=f3(z)
⇒f3∘f1=f3
{f}3∘{of}2={f}3[{f}2({z})]
={f}3(−{z})=−1{z}={f}4({z})
⇒{f}3∘{f}4={f}4
{f}3∘{f}3({z})={f}3[{f}3({z})]
={f}3(1{z})={z}={f}1({z})
⇒{f}3∘{f}3={f}1
{f}3∘{f}4({z})={f}3[{f}4({z})]
={f}3(−1{z})=−{z}={f}2({z})
⇒{f}3{of}4=−{f}2
{f}4∘{f}1({z})={f}4[{f}1({z})]
={f}4({z})=−1{z}={f}3({z})
⇒{f}4∘{f}2={f}3
{f}4∘{f}3({z})={f}4[{f}3({z})]
=f4(1z)=−z=f2(z)
⇒{f}4{of}3={f}2
{f}4{of}4({z})={f}4[{f}4({z})]
={f}4(−1{z})={z}={f}1({z})
⇒{f}4{of}4={f}1
∴ Table for above operations
Of1f2f3f4
f1f1f2f3f4
f2f2f1f4f3
f3f3f4f1f2
f4f4f3f2f1
Hence, it is clear that f1 is identity function of f1,f2,f3,f4.
Hence, inverse element is itself too.
|
Updated on:21/07/2023
Related Playlists
Similar Questions
On the set C of all complex numbers an operation 'o' is defined by z1 o z2=√z1z2 for all z1, z2∈C . Is o a binary operation on C ?
View Solutionf(z)=|z|2 is
View SolutionThe function f(z)=tan z
View SolutionC is the complex numvers f:C→R is defined by f(z)=∣∣z3−z+2∣∣. Find the maximum value of f(z),If|z|=1.
View SolutionLet A, B, C be three sets of complex numbers as defined below. A={z:|z+1|≤2+Re(z)},B={z:|z−1|≥1}andC={z:∣∣∣z−1z+1∣∣∣≥1}
View SolutionThe function f(z)=|z|2 is
View SolutionThe function f(z) = sec z is
View SolutionLet R be the relation defined on set of all complex numbers C as z1Rz2⇔|z1|=|z2| then 'R' is -
View SolutionLet f:N→Z be a function defined as f(x)=x−1000. Show that f is an into function.
View SolutionOn C, the set of complex number, define a relation R as follows:
R={(z1,z2):z1,z2∈C,|z1+z2|=|z1|+|z2|}View Solution