Consider the system of equations
x+y+z=6
x+2y+3z=10
x+2y+λz=μ
The system has no solution if
(a) λ≠3
(b) λ=3,μ=10
(c) λ=3,μ≠10
(d) none of these
λ≠3
λ=3,μ=10
λ=3,μ≠10
none of these
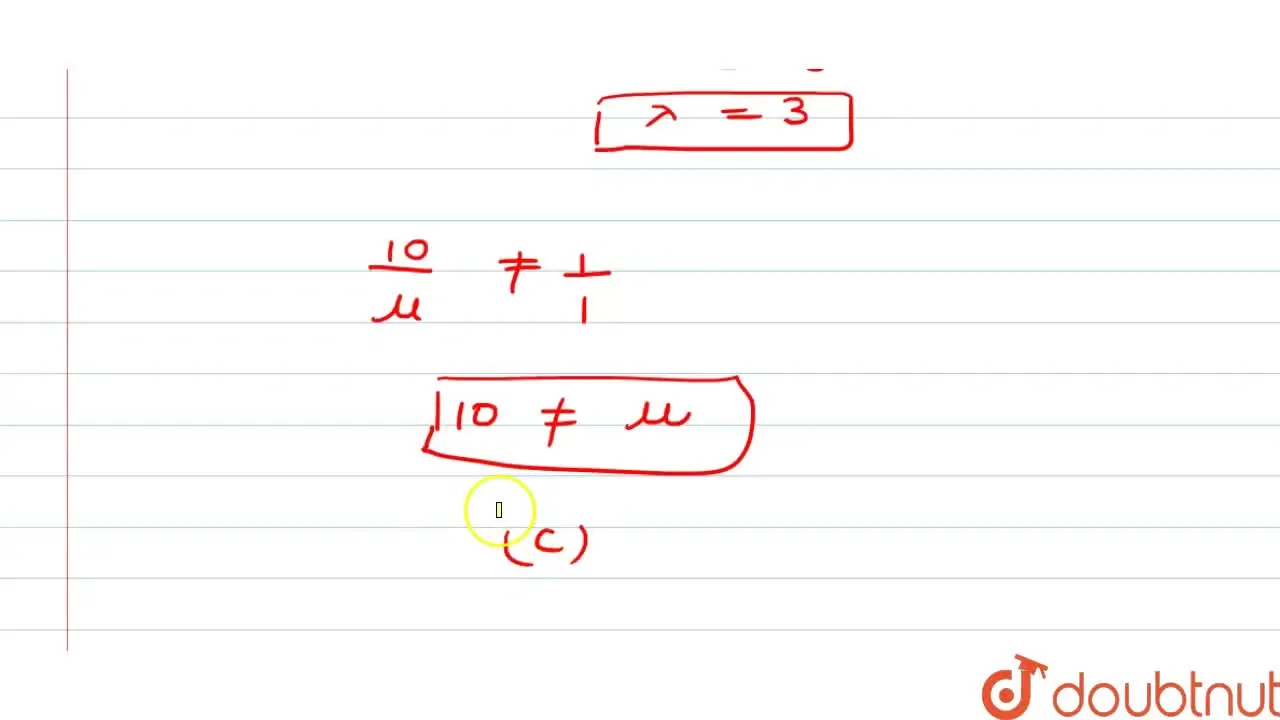
The correct Answer is:C
Δ=∣∣
∣∣11112312λ∣∣
∣∣=2λ+3+2−2−λ−6=λ−3
Δ1=∣∣
∣
∣∣6111023μ2λ∣∣
∣
∣∣=12λ+3μ+20−2μ−10λ−36
=2λ+μ−16
Δ2=∣∣
∣
∣∣16111031μλ∣∣
∣
∣∣=10λ+18+μ−10−3μ−6λ=4λ−2μ+8
Δ3=∣∣
∣
∣∣116121012μ∣∣
∣
∣∣=2μ+10+12−12−μ−20=μ−10
Thus the system has unique solutions if Δ≠0 or λ≠3 and the system has infinite solution if Δ=Δ1=Δ2=Δ3=0 ot λ=3 and μ=10. System has no solution if Δ=0 and at least one of Δ1,Δ2,Δ3 is nonzero of λ=3 and μ≠=10
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTEXERCISE - Multiple Correct Answer5videos
DETERMINANT
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
Consider the system of equations
x+y+z=6
x+2y+3z=10
x+2y+λz=μ
the system has unique solution if (a) λ≠3 (b) λ=3,μ=10 (c) λ=3,μ≠10 (d) none of theseView SolutionConsider the system of equations
x+y+z=6
x+2y+3z=10
x+2y+λz=μ
the system has infinite solutions if (a) λ≠3 (b) λ=3,μ=10 (c) λ=3,μ≠10 (d) λ=3,μ≠10View SolutionIf system of equation
x + y + z = 6
x + 2y + 3z = 10
3x + 2y + λz = μ has more than two solutions. Find (μ−λ)View SolutionIf system of equation x + y + z = 6 ,x + 2y + 3z = 10, 3x + 2y + λz = μ has more than two solutions. Find (μ−λ2)
View SolutionThe system of equations: x+y+z=5, x+2y+3z=9 and x+3y+λz=μ has a unique solution, if
(a) λ=5,μ=13
(b) λ≠5
(c)λ=5,μ≠13
(d) μ≠13View SolutionIf the system of equations
x+y+z=2
2x+4y−z=6
3x+2y+λz=μ
has infinitely many solutions, then :View SolutionThe system of equations: x+y+z=5 x+2y+3z=9 x+3y+λz=μ has a unique solution, if λ=5,μ=13 (b) λ≠5 λ=5,μ≠13 (d) μ≠13
View SolutionConsider the system of equations
2x+λy+6z=8x+2y+μz=5x+y+3z=4
The system of equations has :
No solution if :View SolutionThe system of equations x+y+z=6,x+2y+3z=10,x+2y+λz=k is inconsistent if λ=.........,k≠.........
View SolutionIf the system of linear equation x+y+z=6,x+2y+3c=14 and 2x+5y+λz=μ
A. Unique solution when λ≠8,μ≠36
B. Infinitely many solutions when λ=8,μ=36
C. No solution when λ=8,μ≠36
D. None of theseView Solution