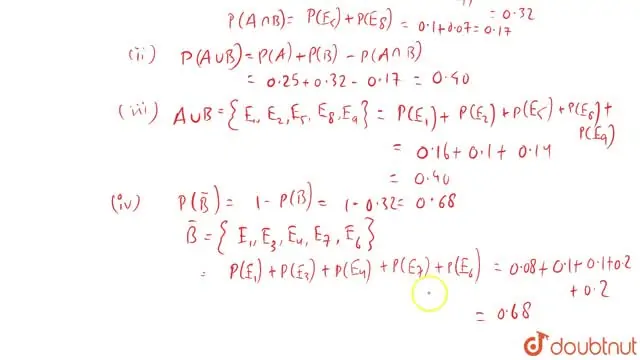A sample space consists of 9 elementary outcomes E1,E2,…..,E9 whose probabilities are
P(E1)=P(E2)=0.08,P(E3)=P(E4)=P(E5)=0.1
P(E6)=P(E1)=0.2,P(E8)=P(E9)=0.07
Suppose A={E1,E5,E8},B={E2,E5,E8,E9}
(i) Calculate P(A), P(B) and P(A∩B).
(ii) Using the addition law of probability, calculate P(A∪B).
(iii) List the composition of the event A∪B and calculate P(A∪B) by adding the probabilities of the elementary outcomes.
Calculate P(−B) from P(B), also calculate P(−B) directly from the elementary outcomes of −B,
Given S={E1,E2,E3,E4,E5,E6,E7,E8,E9}
A={E1,E5,E8},B={E2,E5,E8,E9}
P(E1)=P(E2)=0.08
P(E3)=P(E4)=P(E5)=0.1
P(E6)=P(E7)=2,P(E8)=P(E9)=0.07
(i) P(A)=P(E1)+P(E5)+P(E8)
=0.08+0.1+0.07=0.25
(ii) P(A∪B)=P(A)+P(B)−P(A∩B)
Now, P(B)=P(E2)+P(E5)+P(E8)+P(E9)
=0.08+0.1+0.07+0.07=0.32
A∩B={E5,E8}
P(A∩B)=P(E5)+P(E8)=0.1+0.7=0.17
On substituting these values in Eq. (i), we get
P(A∪B)=0.25+0.32−0.17=0.40
(iii) A∪B={E1,E2,E5,E8,E9}
P(A∪B)=P(E1)+P(E2)+P(E5)+P(E8)+P(E9)
=0.08+0.08+0.1+0.07+0.07=0.40
(iv) ∵P−(B)=1−P(B)=1−0.32=0.68
and −B={E1,E3,E4,E6,E7}
(iv) ∵ P−(B)=P(E1)+P(E3)+P(E4)+P(E6)+P(E7)
=0.08+0.1+0.1+0.2+0.2=0.68
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PROBABILITYEXERCISE - Objective Type Questions12videos
PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PROBABILITYEXERCISE - True/False7videos
PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PROBABILITYEXERCISE - Matching The Columns2videos
PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTION EXERCISE - OBJECTIVE TYPE QUESTIONS5videos
PRINCIPLE OF MATHEMATICAL INDUCTION
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - True /False5videos
RELATIONS AND FUNCTIONS
Similar Questions
A sample space consists of 9 elementary event E1,E2,E3.....E8,E9 whose probabilities are P(E1)=P(E2)=0.08 ,P(E3)=P(E4)=P(E5)=0.1, P(E6)=P(E7)=0.2 ,P(E8)=P(E9)=0.07. Suppose A={E1,E5,E8}, B={E2,E5,E8,E9}. Compute P(A), P(B) and P(A∩B). Using the addition law of probability, find P(A∪B). List the composition of the event A∪B, and calculate, P(A∪B) by adding the probabilities of the elementary events. Calculate P(¯¯¯B) from P(B), also calculate P(¯¯¯B) directly from the elementary events of ¯¯¯B.
View SolutionA sample space consists of 9 elementary event E1,E2,E3.....E8,E9 whose probabilities are P(E1)=P(E2)=0.08 ,P(E3)=P(E4)=P(E5)=0.1, P(E6)=P(E7)=0.2 ,P(E8)=P(E9)=0.07. Suppose A={E1,E5,E8}, B={E2,E5,E8,E9}. Compute P(A), P(B) and P(A∩B). Using the addition law of probability, find P(A∪B). List the composition of the event A∪B, and calculate, P(A∪B) by adding the probabilities of the elementary events. Calculate P(¯¯¯B) from P(B), also calculate P(¯¯¯B) directly from the elementary events of ¯¯¯B.
View SolutionIf P(E)=0.08, What is the probability of not E.
View SolutionIf P(E)=0.05, what is the probability of not E?
View SolutionIf P(E)=0.25, What is the probability of not E.
View SolutionIf the probability of an event E happening is 0.023, then P(¯¯¯E)=______
View SolutionIf P(A)=0.4,P(B)=0.8 and P(B/A)=0.6, then P(A∪B) is equal to
View SolutionIf E1 and E2 be two events such that P(E1)=0.3,P(E2)=0.2 and P(E1∩E2)=0.1, then find:
(i) P(¯¯¯E1∩E2) (ii) P(E1∩¯¯¯E2)View SolutionIf E1 and E2 are two events such that P(E1)=1/4, P(E2/E1)=1/2 and P(E1/E2)=1/4, then
View SolutionFor two events E1 and E2,P(E1)=12,P(E2)=13 and P(E1∩E2)=110. Find:
(i) P(E1orE2)
(ii) P(E1but notE2)
(iii) P(E2but notE1)
View Solution