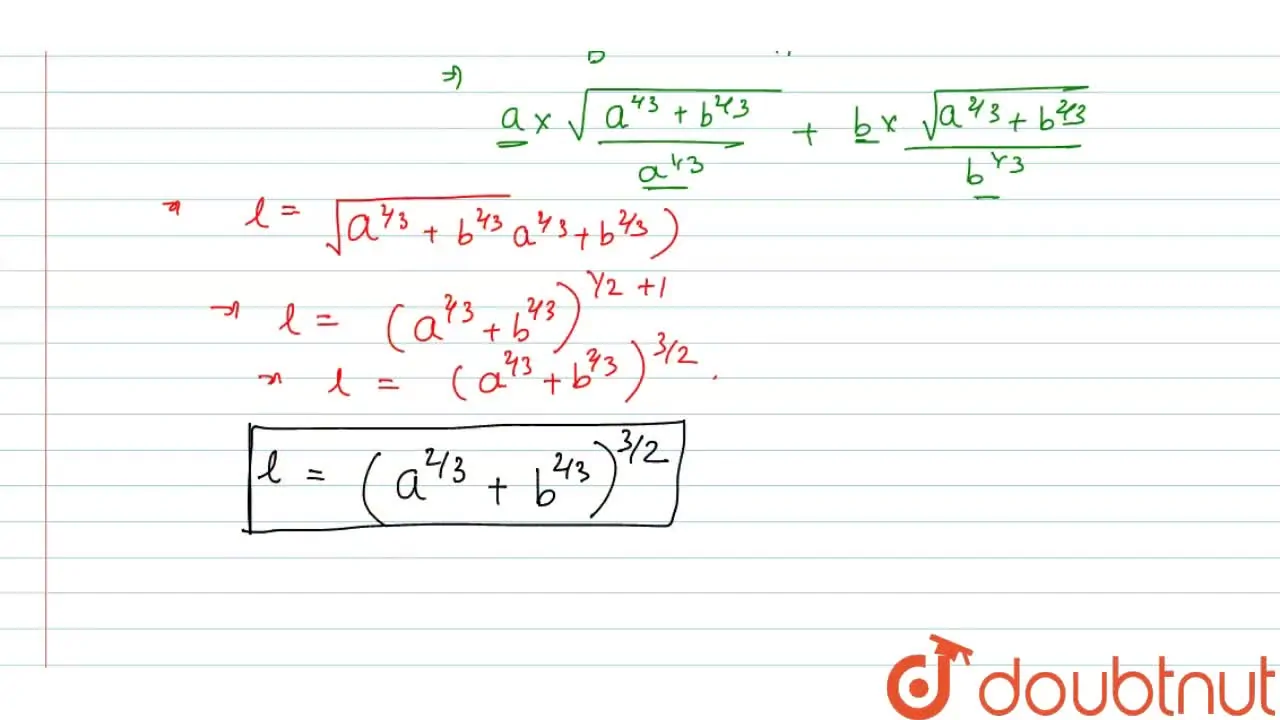
A point on the hypotenuse of a right triangle is at distances a and b from the sides of the triangle. Show that the minimum length of the hypotenuse is (a23+b23)32
Step by step video & image solution for A point on the hypotenuse of a right triangle is at distances a and b from the sides of the triangle. Show that the minimum length of the hypotenuse is (a^(2/3)+b^(2/3))^(3/2) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle. Show that the maximum length of the hypotenuse is (a23+b23)32 .
View SolutionProve that the mid-point of the hypotenuse of a right triangle is equidistant from its vertices.
View SolutionThe hypotenuse of a right angled triangle is 45 cm long. If one of the legs of this triangle is 36 cm, then find the length of other leg.
View SolutionIf A be the area of a right triangle and b one of the sides containing the right angle, prove that the length of the altitude on the hypotenuse is 2AB√b4+4A2
View SolutionThe hypotenuse of a right triangle is 25 cm. The difference between the lengths of the other two sides of the triangle is 5 cm. Find the lengths of these sides.
View SolutionFind the area of a right triangle with hypotenuse of 10 and side of 8
View SolutionThe hypotenuse of a right triangle is 13cm long. If one of the remaining two sides is of length 5cm, find the length of another side.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View Solution