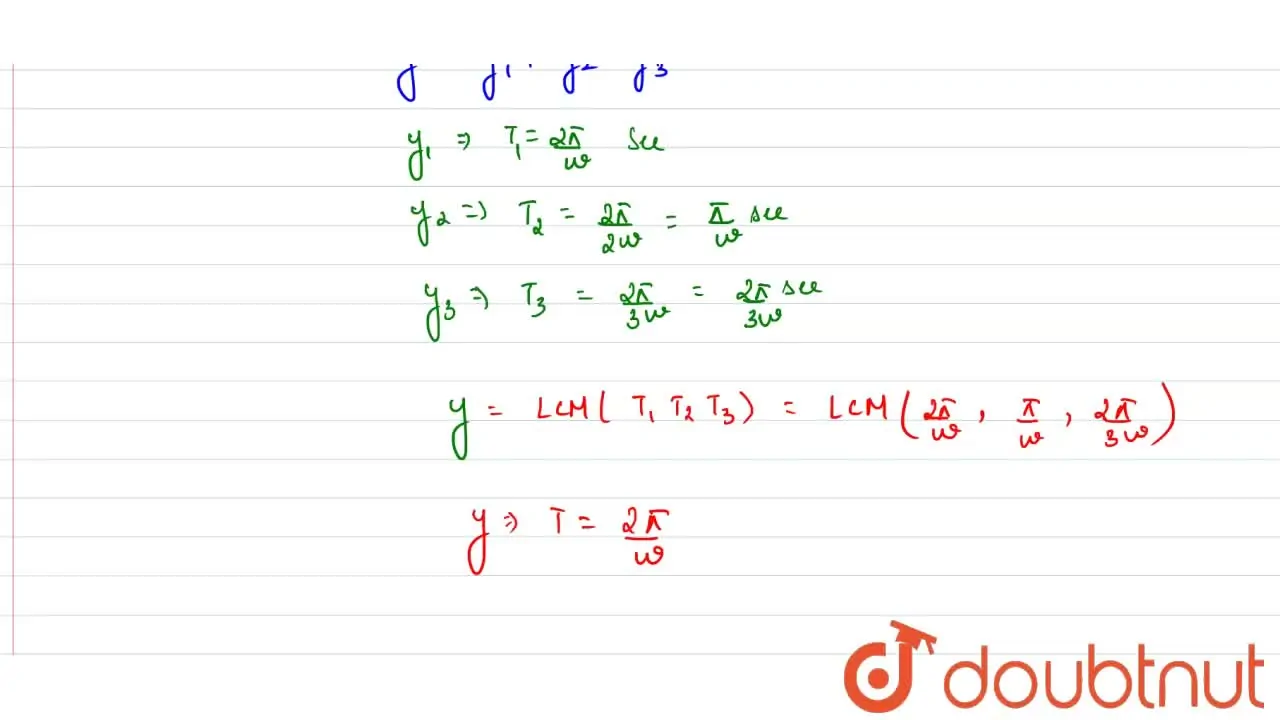
Find time period of the function,
The correct Answer is:A, B
The given function can be written as,
Here, ,
,
and
and
So, the time period of the given function is or .
Because in time , first function completes one oscillation, the second function two oscillation and the third three.
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example Type 11videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example Type 21videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
Categorise the following function of time :sinωt+sin3ωt+sin5ωt as
(a) S.H.M.
(b) Periodic but no S.H.M. .Also give the period.
Hint : Find the smallest interval of time after which the sum of 3 terms repeatsView SolutionIdentify which of the following function represent simple harmonic motion.
(i) Y=AeIωt (ii) Y=ae−ωt
(iii) y=asin2ωt (iv) y=asinωt+bcosωt
(v) y=sinωt+bcos2ωtView SolutionIf the displacement of a moving point at any time is given by an equation of the form y(t)=acosωt+bsinωt, shown that the motion is simple harmonic . If a=3m,b=4mandω=2: determine the period , amplitude, maximum velocity and maximum acceleration.
View SolutionTwo particle AandB execute simple harmonic motion according to the equation y1=3sinωt and y2=4sin[ωt+(π/2)]+3sinωt. Find the phase difference between them.
View SolutionThe SHM of a particle is given by the equation x=2sinωt+4cosωt. Its amplitude of oscillation is
View SolutionTwo simple harmonic motion are represrnted by the following equation y1=40sinωt and y2=10(sinωt+ccosωt). If their displacement amplitudes are equal, then the value of c (in appropriate units) is
View SolutionTwo simple harmonic motion are represrnted by the following equation y1=40sinωt and y2=10(sinωt+ccosωt). If their displacement amplitudes are equal, then the value of c (in appropriate units) is
View SolutionFind the amplitude and initial phase of a partical in SHM, whose motion equation is given as
y=Asinωt+BcosωtView SolutionCategorise the following function of time :sinωt+sin5ωt as
(a) S.H.M.
(b) Periodic but no S.H.M. .Also give the period.
Hint : Find the smallest interval of time after which the sum of 2 terms repeatsView SolutionA particle moves along the x-axis according to the equation x=asinωt+bcosωt. The motion is simple harmonic with
View Solution