Deduce the relation for the magnetic induction at a point due to an infinitely long straight conductor carrying current. Solution in Tamil
Magnetic field due to long straight conductor carrying current: Consider a long straight wire NM with current I flowing from N to M. Let P be the point at a distance a from point 0. Consider an element of length dl of the wire at a distance I from point O and →r be the vector joining the element dl with the point P. Let θ be the.angle between dl and →r . Then, the magnetic field at P due to the element is
d→B=μ0Id→l4πr2sinθ (Unit vector perpendicular to →dl and →r) .........(i)
The direction of the field is perpendicular to the plane of the paper and going into it. This can be determined by taking the cross product between two vectors and →dl (let it be ˆn). The net magnetic field can be determined by integrating equation with proper limits.
→B=∫d→B
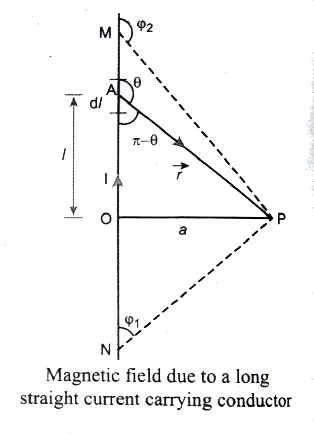
From the figure, in a right angle triangle PAO,
tan(π−θ)=al
l=−asinθ ,since tan(π−θ)=−tanθ⇒1tanθ=cotθ
l=−acotθ and r=acosecθ
Differentiating,
dl=acosec2θ,dθ
d→B=μ0I4πacosec2θdθ(a cosec θ)2sindθˆ
d→B=μ0I4πasinθdθˆn
This is the magnetic field at a point P due to the current in small elemental length. Note that we have expressed the magnetic field OP in terms of angular coordinate i.e. 0. Therefore, the net magnetic field at the point P which can be obtained by integrating d→B by varying angle from θ=φ1 to θ=φ2 is
→B=μ0I4πa∫φ2φ1sinθdθˆn=μ0I4πa(cosφ1−cosφ2)ˆn
Note that here ˆn represents the unit vector from the point O to P.
Related Playlists
Similar Questions
What are the differences between Coulomb force and gravitational force ?
02:57View SolutionCalculate the electric field due to a dipole on its axial line and equatorial plane.
03:06View SolutionHow do we determine the electric field due to a continuous charge distribution ? Explain. Electric field due to continous charge distribution
01:24View SolutionWrite the relation for the magnetic field induction at a point due to a linear conductor carrying current and hence deduce the relation for the magnetic field induction at a point due to a very long linear conductor carrying current.
02:54View SolutionThe magnetic induction due to an infinitely long straight wire carrying a current i at a distance r from wire is given by
03:48View SolutionThe magnetic induction at any point due to a long straight wire carrying a current is
11:14View SolutionUsing Ampere's circuital law, obtain an expression for the magnetic induction at a point near an infinitely long straight conductor carrying an electric current.
11:14View SolutionFind the magnetic induction due to a long current carrying conductor.
11:14View SolutionDeduce the relation for the magnetic induction at a point due to an infinitely long straight conductor carrying current.
04:30View SolutionDeduce the relation for the magnetic induction at a point due to an infinitely long straight conductor carrying current.
05:41View SolutionDeduce the relation for the magnetic induction at a point due to an infinitely long straight conductor carrying current.
01:23View SolutionMagnetic induction at a point due to a small element of current carrying conductor is
View SolutionDerive an expression for the magnetic induction at a nearby point of a straight and long current-carrying conductor. Discuss the nature of the magnetic lines of induction.
View SolutionThe shape of the magnetic lines of force due to an infinite, long, straight current carrying conductor is
View SolutionWrite an expression for magnetic induction due to a long straight conductor carrying current I.
View Solution


