A wooden block of 5.0 kg mass lies on a horizontal wooden table. A bullet of 9.0 g mass hits the block after which it moves a distance 25 cm across the table before stopping. Find the bullet's speed.
The correct Answer is:670 m/s
Take the coordinate axis to coincide with lhe direction of the bullet.s flight. Let the mass of the bullet be m and the mass of the block be M. By the law of conservation of momentum mv = (M + m) u, where v and u are the projections of the velocity of the bullet and of the block after it is hit by the bullet. A force of friction acts on the sliding block in the opposite direction to the velocity vector: T = = - µg (M + m). The acceleration of the block is a= T//(M + m) = = -µg. The block comes lo rest after travelling a distance l, therefore O−u2=2al , or u=−√2µgl. Hence
v=M+mm√2μgl
Substituting numerical values and noting that m<<M, we obtain the value of the velocity cited in the answer.
Similar Questions
A wooden block of mass M rests on a horizontal surface. A bullet of mass m moving in the horizontal direction strikes and gets embedded in it. The combined system covers a distance x on the surface. If the coefficient of friction between wood and the surface is μ , the speed of the bullet at the time of striking the block is (where m is mass of the bullet)
View SolutionThe ballistic pendulum is a block of 3.0 kg mass suspended from a thread 2.5 m long. A bullet with the mass of 9.0 g hits the block and sticks in it, the result being a deflection of the system by an angle of 18∘ (Fig). Find the bullet's speed
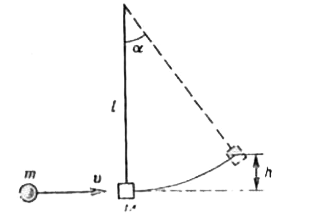 View Solution
View SolutionA bullet of mass 20 g moving horizontally at a speed of 300 m/s is fired into a wooden block of mass 500 g suspended by a long string. The bullet crosses the block and emerges on the other side. If the centre of mass of the block rises through a height of 20.0 cm, find the speed of the bullet as it emerges from the block.
View SolutionA bullet of mass 0.01 kg is fired horizontal into a 4 kg wooden block block at rest , on a horizontal surface. The coefficient of kinetic friction between the block and surface is 0.25 the combination moves 20 m before coming to rest . With what speed did the bullet strike the block ?
View SolutionA bullet of mass 10 g is fired horizontally into a 4 kg wooden block resting on a horizontal surface. The coefficient of kinetic friction between the block and the surface is 0.25. The bullet is embadded the block and the combination moves a distance of 20 m before coming to rest. Find the speed of the bullet just before striking the block. (g=10m/s2)
View SolutionA bullet of mass 10 gm moving with a horizontal velocity 100m/s passes through a wooden block of mass 100 gm. The block is resting on a smooth horizontal floor. After passing through the block the velocity of the bullet is 10m/s. the velocity of the emerging bullet with respect to the block is
View SolutionA bullet of mass 10gm moving with a horizontal velocity 100m/s passes through a wooden block of mass 100gm. The block is resting on a smooth horizontal floor. After passing through the block the velocity of the bullet is 10m/s the velocity of the emerging bullet with respect to the block is .
View SolutionA block of a mass of 10 kg rests on a horizontal table. The coefficient of friction between the block and the table is 0.05. When hit by a bullet of mass 50 g moving with speed v, that gets embedded in it, the block moves and comes to rest after moving a distance of 2 m on the table. If a freely falling object were to acquire speed 10v after being dropped from height H, then neglecting energy losses and taking g=10 S.I. , the value of H is close to:
View SolutionA bullet of mass 0.005 kg moving with a speed of 200 ms−1 enters a heavy wooden block and is stopped after a distance of 50 cm. What is the average force exerted by the block on the bullet?
View SolutionA bullet of mass 0.1 kg is fired on a wooden block to pierce through it, but it stops after moving a distance of 50 cm into it. If the velocity of bullet before hitting the wood is 10 m/s and it slows down with uniform deceleration, then the magnitude of effective retarding force on the bullet is 'x N.
The value of 'x to the nearest integer is___________View Solution

