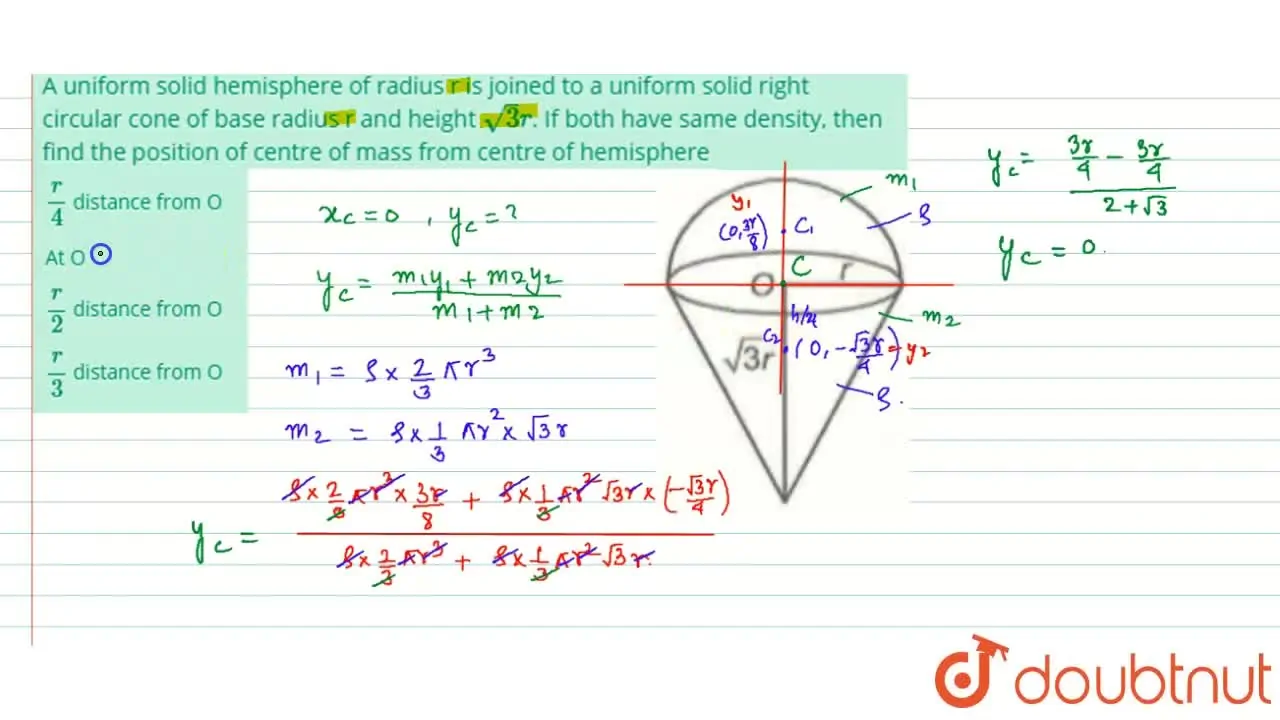
A uniform solid hemisphere of radius r is joined to a uniform solid right circular cone of base radius r and height √3r. If both have same density, then find the position of centre of mass from centre of hemisphere 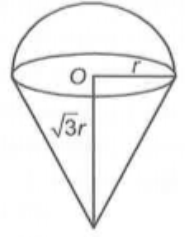
r4 distance from O
At O
r2 distance from O
r3 distance from O
Step by step video & image solution for A uniform solid hemisphere of radius r is joined to a uniform solid right circular cone of base radius r and height sqrt3r. If both have same density, then find the position of centre of mass from centre of hemisphere by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, as shown. The centre of mass of the composite solid lies at the centre of base of the cone. The height of the cone is
 View Solution
View SolutionA hemisphere of lead of radius 7 cm is cast into a right circular cone of height 49cm. Find the radius of the base.
View SolutionA hemisphere of lead of radius 7 cm is cast into a right circular cone of height 49cm. Find the radius of the base.
View SolutionA hemisphere of lead of radius 7 cm is cast into a right circular cone of height 49cm. Find the radius of the base.
View SolutionA hemisphere of lead of radius 7 cm is cast into a right circular cone of height 49cm. Find the radius of the base.
View SolutionFind the centre of mass of a uniform solid cone.
View SolutionA uniform solid sphere of mass m and radius r is suspended symmetrically by a uniform thin spherical shell of radius 2r and mass m.
View SolutionA hemisphere of lead of radius 8cm is cast into a right circular cone of base radius 6cm. Determine the height of the cone, correct to two places of decimal.
View SolutionA hemisphere of lead of radius 8cm is cast into a right circular cone of base radius 6cm. Determine the height of the cone, correct to two places of decimal.
View SolutionDetermine the centre of mass of a uniform hemisphere of radius R.
View Solution