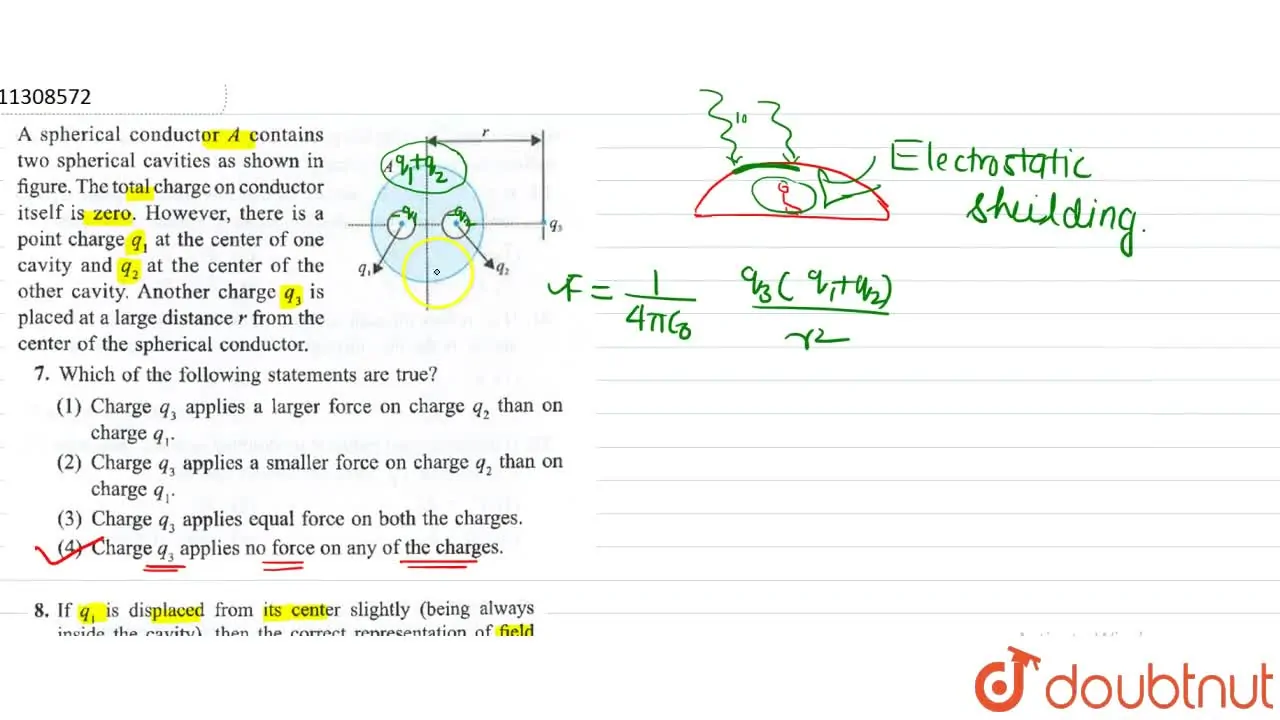
A spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
The force acting on conductor A will be
zero
q3(q1+q2)4πε0r2
−q3(q1+q2)4πε0r2
q3q1+q2q3+q1q24πε0r2
The correct Answer is:B
We see that that surface of two spherial cavities carries charges q1,−q2 respectively, and since the sphere A was not charged orginally, its sphereical surface must carry induced charges (q1+q2). Therefore, force acting on conductor A is q3(q1+q2)/4πε0r2
Related Playlists
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Subjective type7videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Multiple Correct8videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger8videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.514videos
ELECTRIC POTENTIAL
Similar Questions
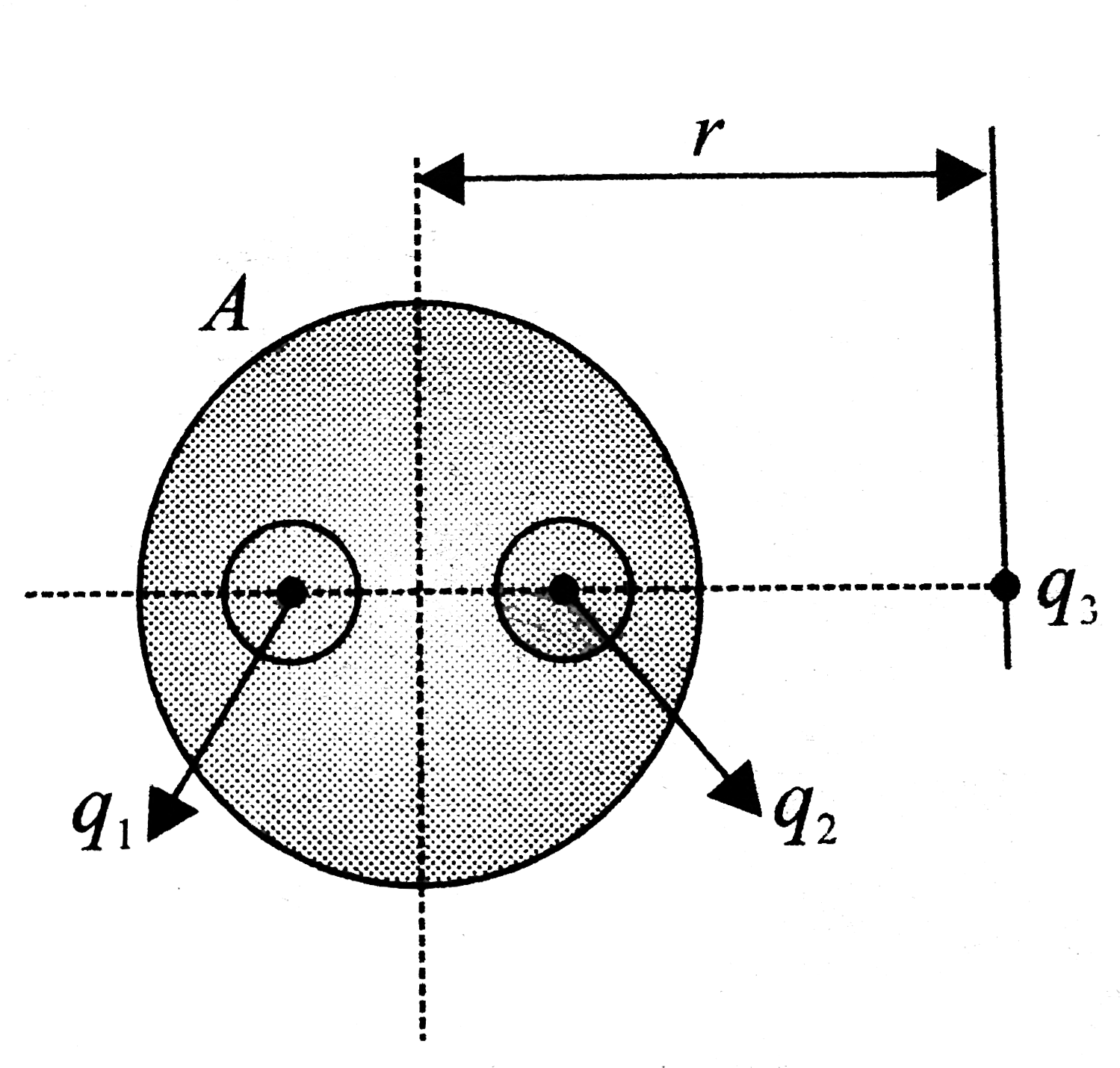
A spherical conductor A contains two spherical cavities as shown in Fig. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
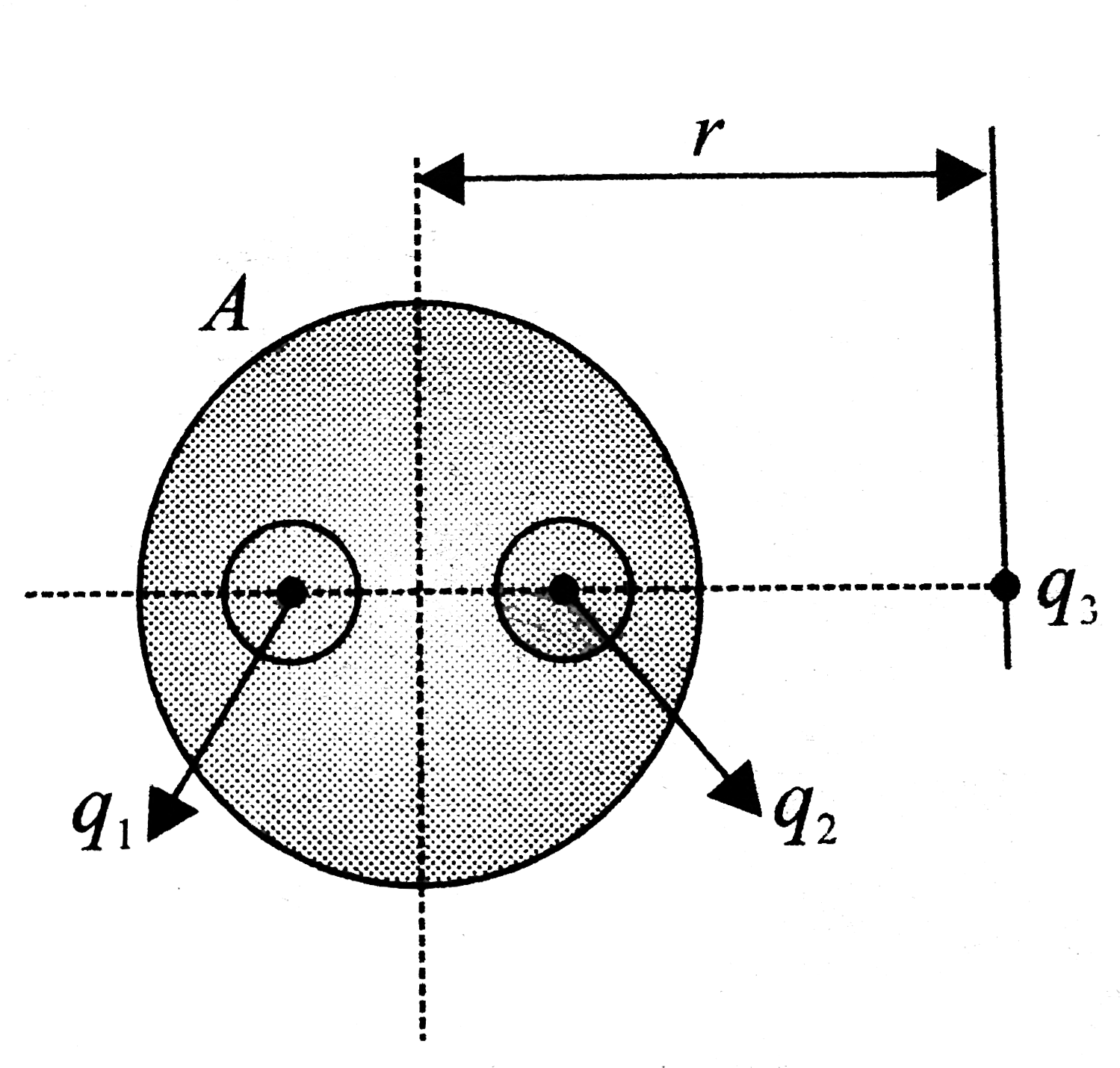
Which of the following statements are true ?View SolutionA spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
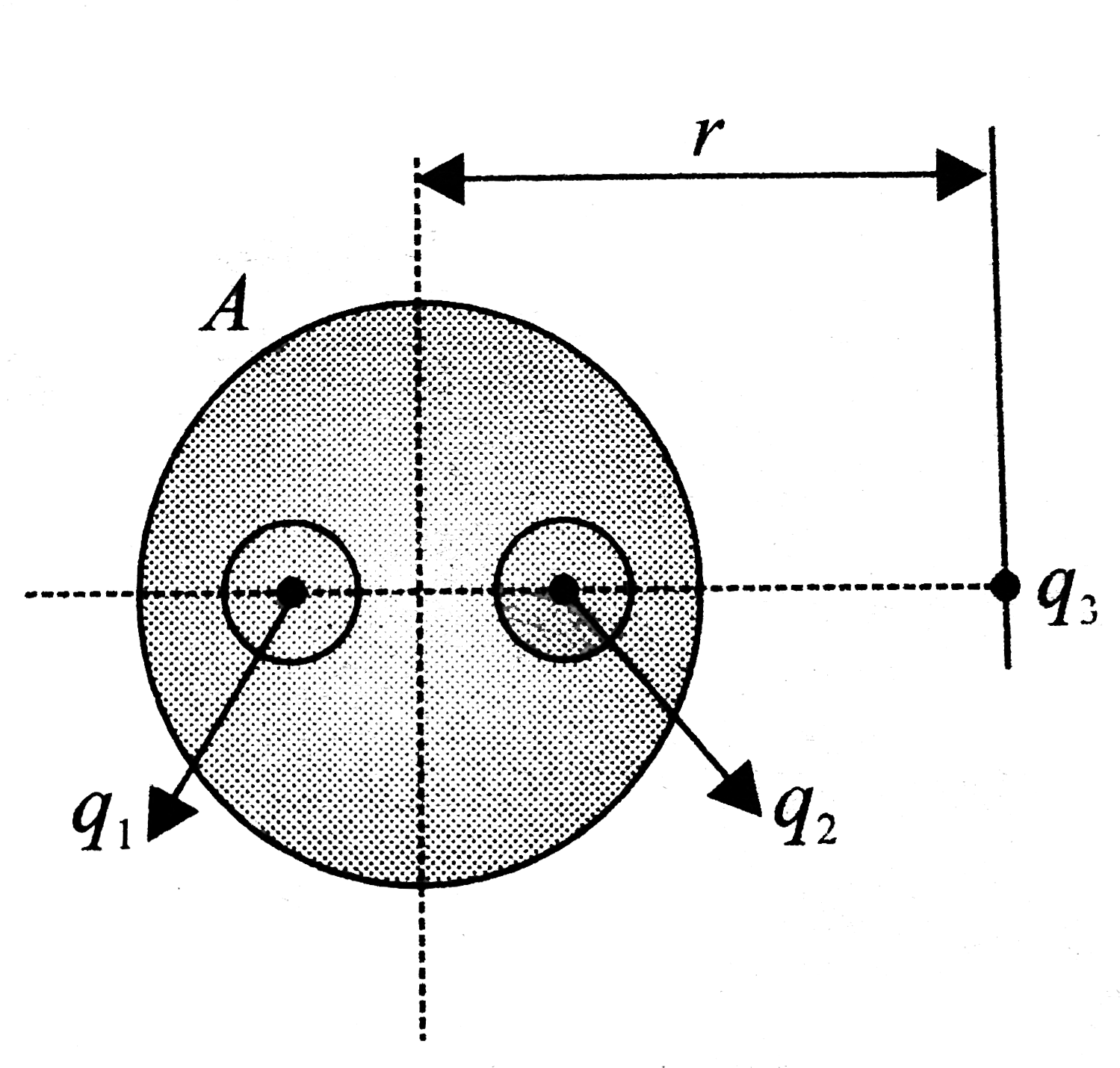
If q1 is displaced from its center slightly (being always inside the same cavity ), then the correct representation of field lines inside the same cavity isView SolutionA spherical conductor A contains two spherical cavities. The total charge on the conductor itself is zero. However, there is a point charge qb at the centre of one cavity and qc at the centre of the other. At considerable distance r away from the centre of the spherical conductor, there is another charge qd. Forces acting on qb,qcandqd are F1,F2,andF3, respectively. [Assume all charges are positive.]
 View Solution
View SolutionElectric potential at the center ofa charged hollow spherical conductor is
View SolutionThe electric field intensity at a point located at distance r(r<R) from the center of a spherical conductor (radius R) charged Q will be-
View SolutionInside a hollow charged spherical conductor, the potential
View SolutionInside a charged hollow spherical conductor. the potential :-
View SolutionA point charge +q is held at a distance R from the centre of an uncharged spherical conductor of radius a(a<R). Calculate the potential of the conductor in the presence of q.
View SolutionA point charge +q is held at a distance R from the centre of an uncharged spherical conductor of radius a(a<R). Calculate the potential of the conductor in the presence of q.
View SolutionTwo spherical cavities of radii a and b are hollowed out from the interior of a neutral conducting sphere of radius R. At the center of each cavity , a point charge is placed . Call these charges qa and qb.
The electric field inside the cavity of radius a at a distance r from the center of cavity is
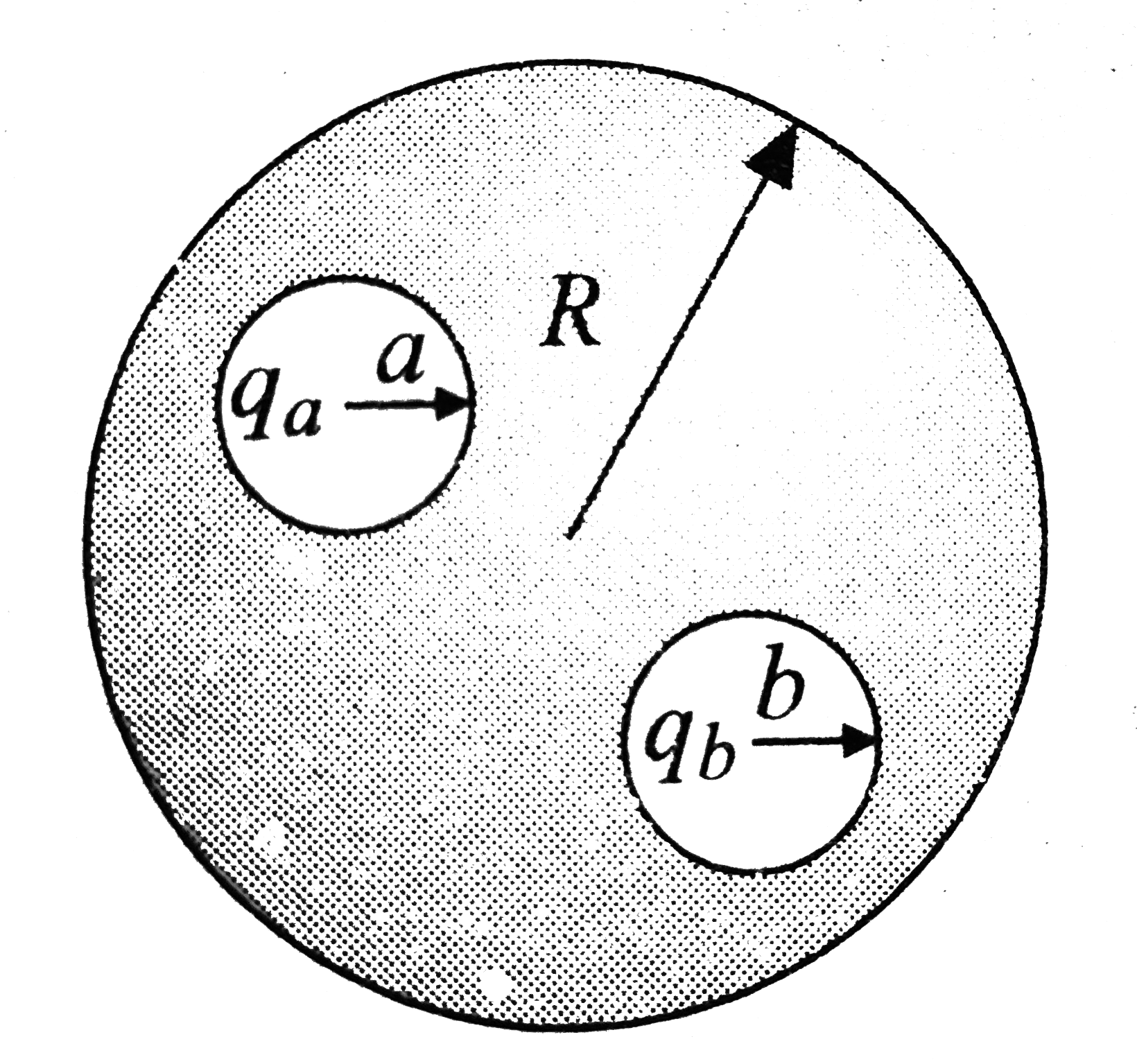 View Solution
View Solution