A body is executing S.H.M. when its displacement from the mean position is 4 cm and 5 cm, the corresponding velocity of the body is 10 cm/sec and 8 cm/sec. Then the time period of the body is
2πsec
π2sec
πsec
3π2sec
The correct Answer is:C
To solve the problem, we need to find the time period of a body executing Simple Harmonic Motion (SHM) given its displacements and corresponding velocities.
1. Understand the equations of SHM:
The displacement y in SHM can be expressed as:
y=Asin(ωt)
where A is the amplitude and ω is the angular frequency.
2. Velocity in SHM:
The velocity v can be expressed as:
v=Aωcos(ωt)
3. Use the given data:
We have two sets of data:
- When y=4cm, v=10cm/s
- When y=5cm, v=8cm/s
4. Set up the equations:
For the first position:
10=Aωcos(ωt1)(1)
4=Asin(ωt1)(2)
For the second position:
8=Aωcos(ωt2)(3)
5=Asin(ωt2)(4)
5. Square and add the equations:
From equations (1) and (2):
v2=A2ω2cos2(ωt)andy2=A2sin2(ωt)
Adding these gives:
v2+y2=A2
For the first position:
102+42=A2⟹100+16=A2⟹A2=116(5)
For the second position:
82+52=A2⟹64+25=A2⟹A2=89(6)
6. Set the equations equal:
From (5) and (6):
116−16=A2−25⟹100=A2−25
Rearranging gives:
A2=125⟹A=√125=5√5
7. Find angular frequency ω:
Substitute A back into either equation (1) or (3). Let's use equation (1):
10=Aωcos(ωt1)
Using A=5√5:
10=5√5ωcos(ωt1)⟹ωcos(ωt1)=2√5(7)
8. Find the time period T:
The angular frequency ω is related to the time period T by:
ω=2πT
Rearranging gives:
T=2πω
9. Calculate T:
If we find ω from the earlier equations, we can substitute it back to find T.
From the earlier calculations, we can derive that:
ω=2⟹T=2π2=πseconds
Final Answer:
The time period T of the body is πseconds.
---
The displacement y in SHM can be expressed as:
y=Asin(ωt)
where A is the amplitude and ω is the angular frequency.
The velocity v can be expressed as:
v=Aωcos(ωt)
We have two sets of data:
- When y=4cm, v=10cm/s
- When y=5cm, v=8cm/s
For the first position:
10=Aωcos(ωt1)(1)
4=Asin(ωt1)(2)
8=Aωcos(ωt2)(3)
5=Asin(ωt2)(4)
From equations (1) and (2):
v2=A2ω2cos2(ωt)andy2=A2sin2(ωt)
Adding these gives:
v2+y2=A2
102+42=A2⟹100+16=A2⟹A2=116(5)
82+52=A2⟹64+25=A2⟹A2=89(6)
From (5) and (6):
116−16=A2−25⟹100=A2−25
Rearranging gives:
A2=125⟹A=√125=5√5
Substitute A back into either equation (1) or (3). Let's use equation (1):
10=Aωcos(ωt1)
Using A=5√5:
10=5√5ωcos(ωt1)⟹ωcos(ωt1)=2√5(7)
The angular frequency ω is related to the time period T by:
ω=2πT
Rearranging gives:
T=2πω
If we find ω from the earlier equations, we can substitute it back to find T.
ω=2⟹T=2π2=πseconds
The time period T of the body is πseconds.
Similar Questions
A body executes SHM with an amplitude a. At what displacement from the mean positions, the potentail energy of the body is one-fourth of its total energy?
View SolutionA body executing S.H.M. has its velocity 10cm/s and 7cm/s when its displacement from the mean positions are 3cm and 4cm respectively. Calculate the length of the path.
View Solution
Knowledge Check
- Question 1 - Select One
The kinetic energy of a particle, executing S.H.M. is 16 J when it is in its mean position. IF the amplitude of oscillation is 25 cm and the mass of the particle is 5.12 Kg, the time period of its oscillations is
Aπ/5 secB2πsecC20πsecD5π sec
The force on a body executing SHM is 4 N when the displacement from mean position is 2 cm. If amplitude of oscillation is 10 cm, then the maximum kinetic energy associated with the SHM will be
View SolutionA body executing SHM has its velocity its 10cm/sec and7cm/sec when its displacement from the mean position are 3cm and 4cm , respectively. Calculate the length of the path.
View SolutionA particle is moving with shm in a-straight line. When the distance of the particle from the equilibrium position has the values 2 cm and 4 cm, the corresponding values of velocities are 5cms−1and3cms−1 respectively. Find the length of the path and the period.
View SolutionDraw a graph between displacement from mean position and time for a body executing free vibration in a vacuum.
View SolutionThe velocity-time graph of a body is shown in .
The displacement of the body in 8s is.
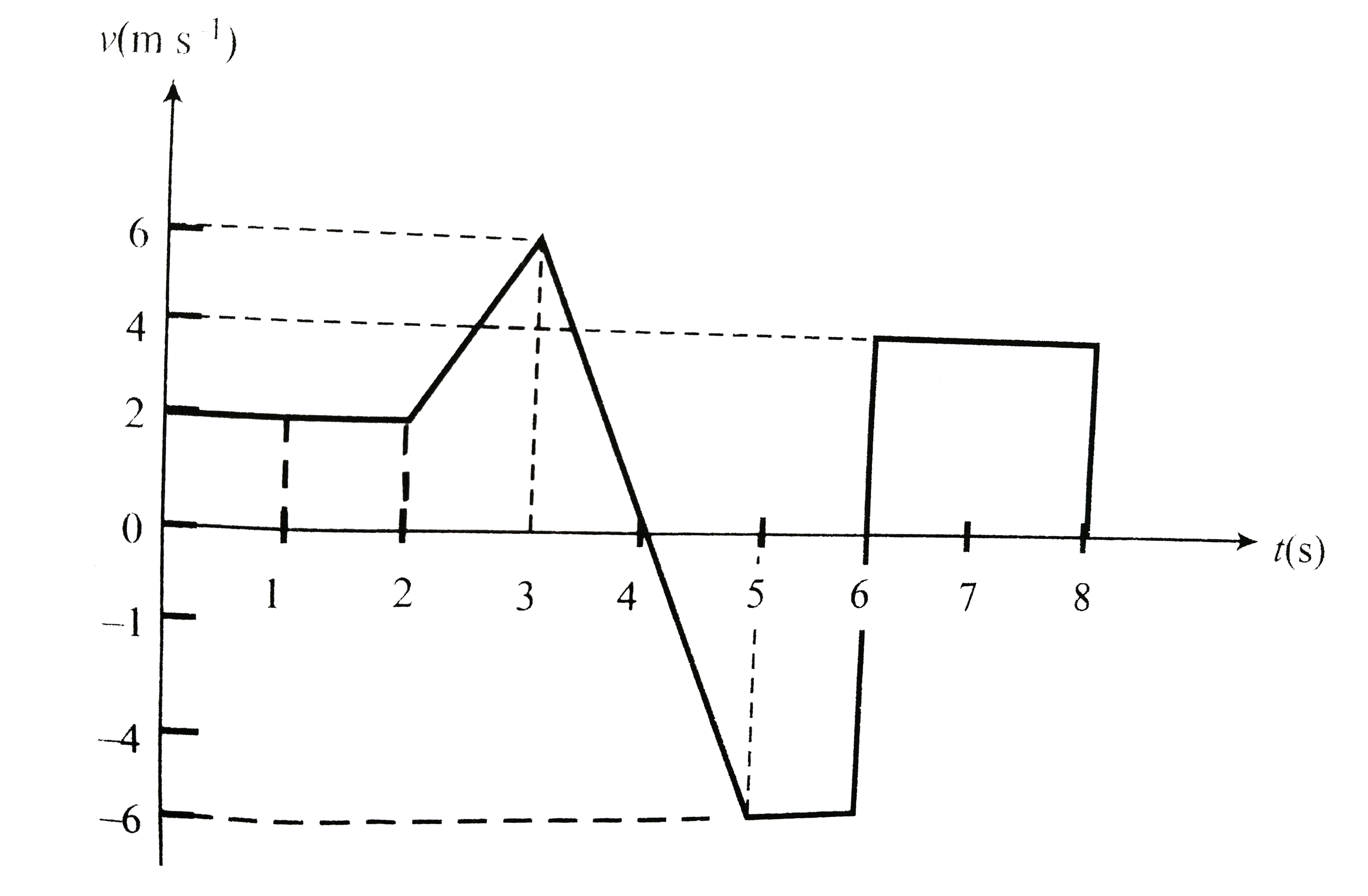 .View Solution
.View SolutionA particle executes SHM on a straight line path. The amplitude of oscialltion is 3 cm. Magnitude of its acceleration is eqal to that of its velocity when its displacement from the mean position is 1 cm. Find the time period of S.H.M.
Hint :A=3cm
Whenx=1cm , magnitude of velocity =magnitude of acceleration
i.e., ω(A2−x2)(12)=ω2x
Find ω
T=(2π)(ω)View SolutionA particle executes linear simple harmonic motion with an amplitude of 3cm. When the particle is at 2cm from the mean position, the magnitude of its velocity is equal to that of its acceleration. Then, its time period in seconds is
View Solution
