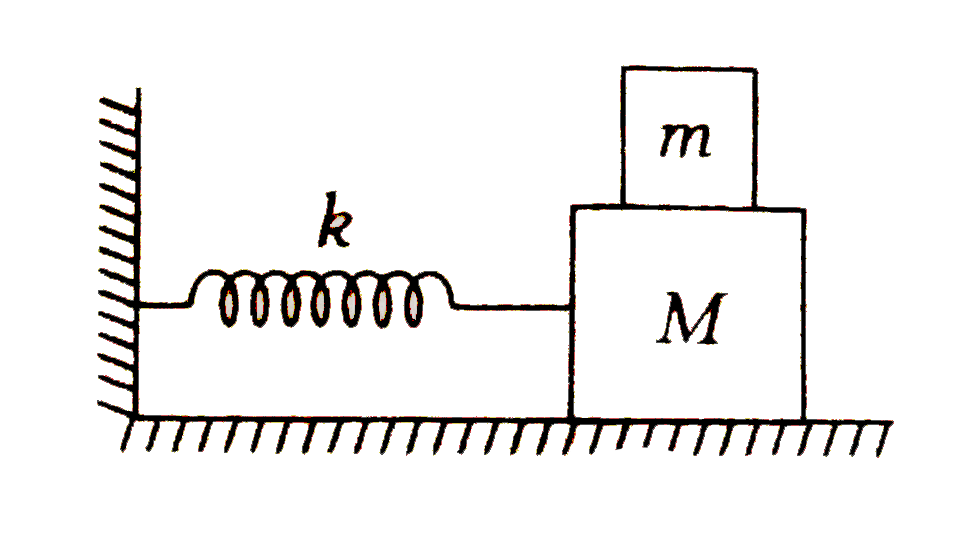
A block with mass M attached to a horizontal spring with force constant k is moving with simple harmonic motion having amplitude A1. At the instant when the block passes through its equilibrium position a lump of putty with mass m is dropped vertically on the block from a very small height and sticks to it.
(a) Find the new amplitude and period.
(b) Repeat part (a) for the case in which the putty is dropped on the block when it is at one end of its path.
The correct Answer is:A, B
(a) Before the lump of putty is dropped the total mechanical energy of the block and spring is
E1=12KA21.
Since, the block is at the equilibrium position, U=0, and the energy is purely kinetic. Let v1 be the speed of the equilibrium position, we have
E1=12Mv21=12kA21
∴v1=√kMA1
During the process momentum of the system in horizontal direction is conserved. Let v2 be the speed of the combined mass, then
(M+m)v2=Mv1
∴v2=MM+mv1
Now, let A2 be the ampllitude afterwards. Then, E2=12kA22=12(M+m)v22
Substituting the proper values, we have
A2=A1√MM+m
Further, T2=2π√M+mk
(b) When the putty drops on the block, the block is instantaneously at rest. All the mechanical energy is stored in the spring as potential energy. Again the momentum in horizontal direction is conserved during the process. But now it is zero just before and after putty is dropped. So, in this case, adding the extra mass of the putty has no effect on the mechanical energy, i.e.
E2=E1=12kA21 and the amplotude is still A1. Thus,
A2=A1
and T2=2π√M+mk
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Single Correct28videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct8videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Single Correct24videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
A particle performs simple harmonic motion wit amplitude A. its speed is double at the instant when it is at distance A3 from equilibrium position. The new amplitude of the motion is
View SolutionA horizontal spring block system of mass M executes simple harmonic motion. When the block is passing through its equilibrium position, an object of mass m is put on it and the two move together. Find the new amplitude and frequency of vibration. Given, k is the spring constant of the system.
View SolutionA horizontal spring -block system of mass 2kg executes S.H.M when the block is passing through its equilibrium position an object of mass 1kg is put on it the two move together The new amplitude of vibration is (A being its initial amplitude)
View SolutionA horizontal spring -block system of mass 2kg executes S.H.M when the block is passing through its equilibrium position an object of mass 1kg is put on it the two move together The new amplitude of vibration is (A being its initial amplitude)
View SolutionA mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
 View Solution
View SolutionA block of mass M attached to the free end of a spring of force constant k is nounted on a smooth horizontal table as shown in figure.
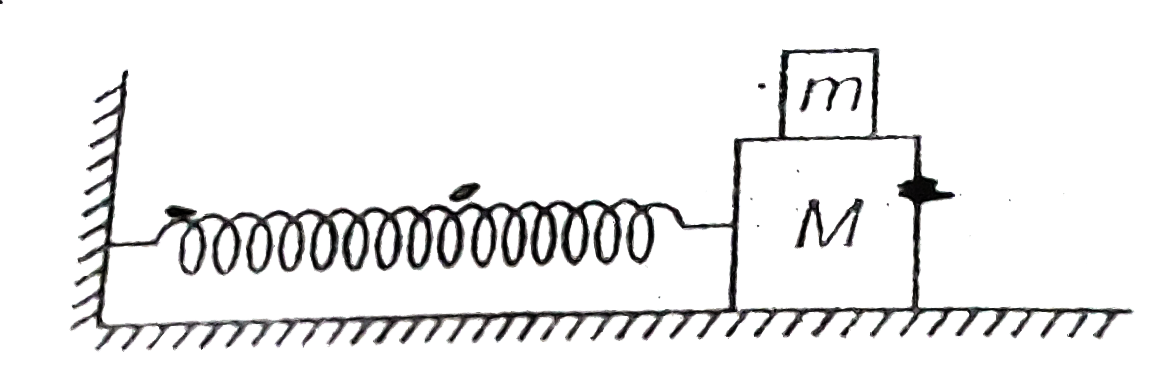
The block executes SHM with amplitude A and frequency f . If an object of mass m is put on it, when the block is passing through its equilibium position and the two move together ,then what is the new amplitude and frequency of vibration?View SolutionA particle performs simple harmonic motion with amplitude A. Its speed is tripled at the instant that it is at a distance
2A3 from equilibrium position. The new amplitude of the motion is:View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View SolutionA particle performs simple harmonic motion with amplitude A. its speed is double at the instant when it is at distance A3 from equilibrium position. The new amplitude of the motion is √33Aβ. Find β
View SolutionA mass M, attached to a horizontal spring, excutes SHM with a amplitude A1. When the mass M passes through its mean position then a smaller mass m is placed over it and both of them move together with amplitude A2, the ratio of (A1A2) is :
View Solution