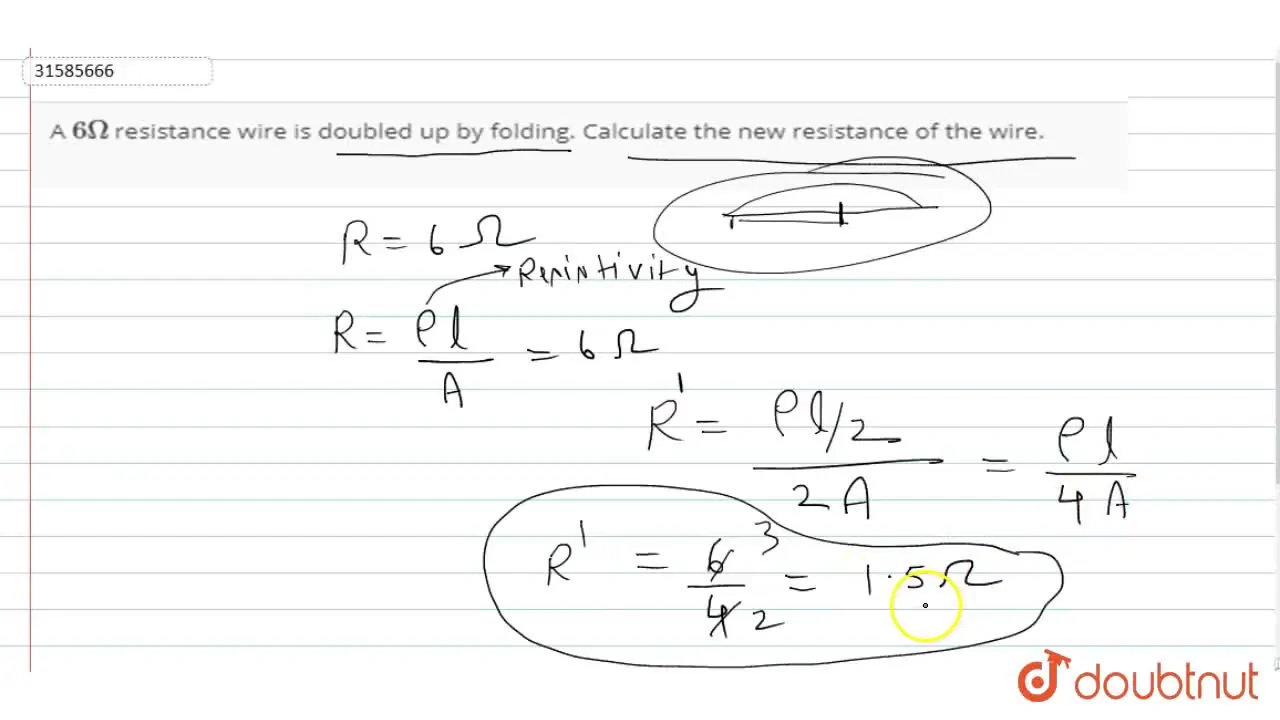
A $6 Ω$ resistance wire is doubled up by folding. Calculate the new resistance of the wire.

A

$1.6 Ω$

B

$1.8 Ω$

C

$1.5 Ω$

D

$1.9 Ω$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Suppose the length of $6 Ω$ resistance wire is l, its area of cross-section is A and its resistivity is $ρ$ . Then:
$R = \frac{ρ \times l}{A}$
or $6 = \frac{ρ \times l}{A}$ ...(1)
Now, when this wire is doubled up by folding, then its length will become half, that is, the length will become $\frac{l}{2}$ . But on doubling the wire by folding, its area of cross-section will becomes double, that its, the area of cross-section will become 2A. Suppose the new resistance of the doubled up wire (or folded wire) is R. So,
$R = \frac{ρ \times l}{2 \times 2 A}$
or $R = \frac{ρ \times l}{4 A}$ ...(2)
Now, dividing equation (2) by equation (1), we get:
$\frac{R}{6} = \frac{ρ \times l \times A}{4 A \times ρ \times l}$
or $\frac{R}{6} = \frac{1}{4}$
$4 R = 6$
$R = \frac{6}{4}$
$R = 1.5 Ω$
Thus, the new resistance of the doubled up wire is $1.5 Ω$ .

|

Class 10 PHYSICS ELECTRICITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

A $5 Ω$ resistance wire is doubled on it. Calculate the new resistance of the wire.
A $1.25 Ω$
B $2.25 Ω$
C $1.5 Ω$
D $1.00 Ω$
A 8 $Ω$ resistance wire is doubled on it. Calculate the new resistance of the wire.
A $1.5 Ω$
B $2.00 Ω$
C $1.00 Ω$
D $1.25 Ω$
A wire of $1 Ω$ resistance is stretched and its length becomes twice, then the new resistance of the wire
A $\frac{1}{4} Ω$
B $2 Ω$
C $\frac{1}{2} Ω$
D $4 Ω$

LAKHMIR SINGH & MANJIT KAUR-ELECTRICITY -NCERT

Home
Ask Doubt
Profile