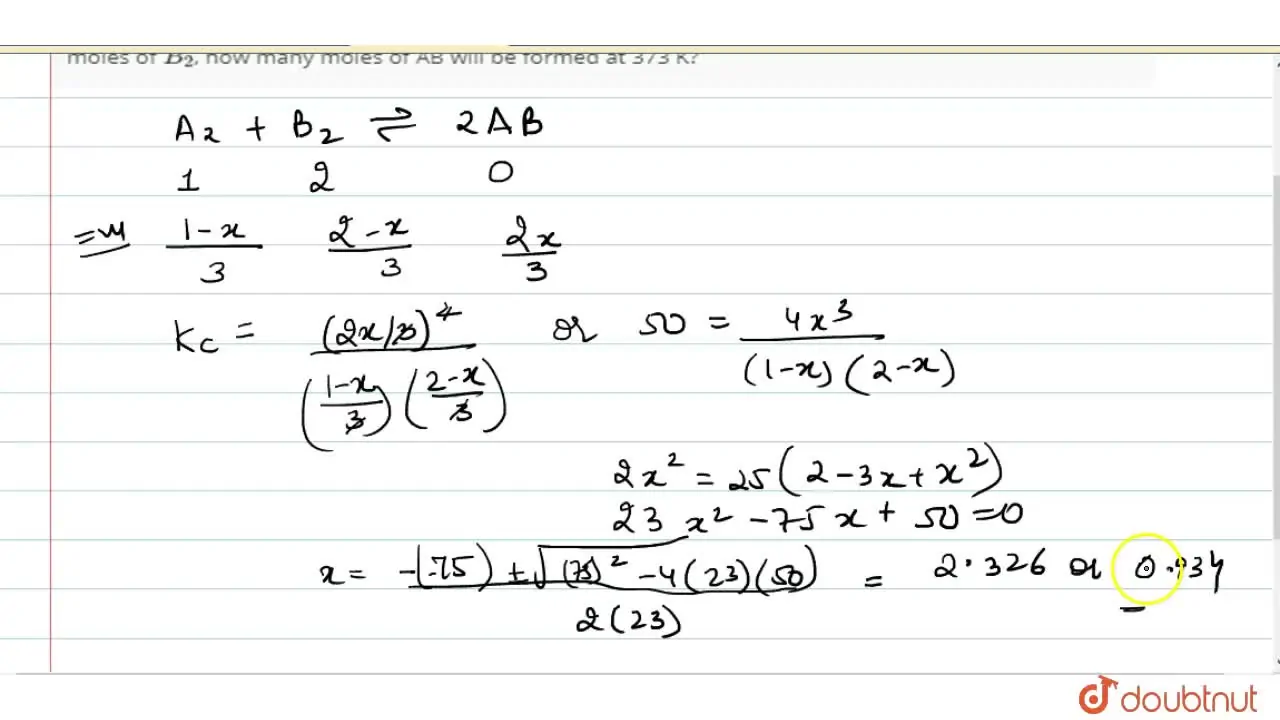
The equilibrium constant for the reaction
A2(g)+B2⇔2AB(g)
at 373 K is 50. If one litre flask containing one mole of A2 is connected to a two flask containing two moles of B2, how many moles of AB will be formed at 373 K?
Total volume available for reaction mixture =3 L
The value of equilibrium constant =50
Let x moles of both the reactants A2 and B2 react to form 2x moles of AB according to the equation given above. Therefore, the molar concentration per litre of the different species at equilibrium point is :
A2(g)+B2(g)⇐⇒2AB(g)Initial moles//litre120Moles //litre at equilibrium point1−x32−x32x3
Applying Law of chemical equilibrium Kc=[AB]2[A2][B2]=(2x3)2(1−x3)×(2−x3)2
50=4x2(1−x)(2−x)
2x2=25(2−3x+x2),23x2−75x+50=0
By solving quadratic equation .∗x=75+––––√(752−4×23×50)2×23=2.326or0.934
Related Playlists
- BOOK - DINESH PUBLICATIONCHAPTER - EQUILIBRIUMEXERCISE - Short Answer Type Question19videos
EQUILIBRIUM
- BOOK - DINESH PUBLICATIONCHAPTER - EQUILIBRIUMEXERCISE - Concept Based Questions19videos
EQUILIBRIUM
- BOOK - DINESH PUBLICATIONCHAPTER - ENVIRONMENTAL CHEMISTRYEXERCISE - Integer Type Questions4videos
ENVIRONMENTAL CHEMISTRY
- BOOK - DINESH PUBLICATIONCHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - UNIT TEST - 341videos
GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS
Similar Questions
The equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 100∘C is 50. If a one litre flask containing one mole of A2 is connected to a two litre flask containing two moles of B2, how many moles of AB will be formed at 373K?
View SolutionThe equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 100∘C is 50. If a one litre flask containing one mole of A2 is connected to a two litre flask containing two moles of B2, how many moles of AB will be formed at 373K?
View SolutionThe equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 100∘C is 50. If a one litre flask containing one mole of A2 is connected to a two litre flask containing two moles of B2, how many moles of AB will be formed at 373K?
View SolutionThe equilibrium constant of the reaction
A2(g)+B2(g)⇔2AB(g)
at 100∘C is 50. If a 1−L flask containing 1 mol of A2 is connected to a 2L flask containing 2 mol of B2, how many moles of AB will be formed at 373K?View SolutionThe Kc for A2(g)+B2(g)⇔2AB(g) at 100∘C is 50. If one litre flasks containing one mole of A2 is connected with a two litre flask containing 2mole of B2, how many mole of AB will be formed at 100∘C?
View SolutionThe value of Kc for the reaction:
A2(g)+B2(g)⇔2AB(g)
at 100∘C is 49. If 1.0L flask containing one mole of A2 is connected with a 2.0L flask containing one mole of B2, how many moles of AB will be formed at 100∘C?View SolutionThe equilibrium constant of the given reaction at 100 degree C is 49. If a one-litre flask containing one mole of A_(2) is connected to a two-litre flask containing one moles of B_(2) how many moles of AB will be formed at 373K ?
View SolutionThe equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 373 K is 50. If 1 L of flask containing 1 mole of A2 (g) is connected to 2 L flask containing 2 moles B2 (g) at 100∘C, the amount of AB produced at equilibrium at 100∘C would be
View SolutionThe equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 373 K is 50. If 1 L of flask containing 1 mole of A2 (g) is connected to 2 L flask containing 2 moles B2 (g) at 100∘C, the amount of AB produced at equilibrium at 100∘C would be
View SolutionThe equilibrium constant of the reaction A2(g)+B2(g)⇔2AB(g) at 373 K is 50. If 1 L of flask containing 1 mole of A2(g) is connected to 2L flask containing 2 moles B2(g) at 100∘C, the amount of AB produced at equilibrium at 100∘C would be
View Solution