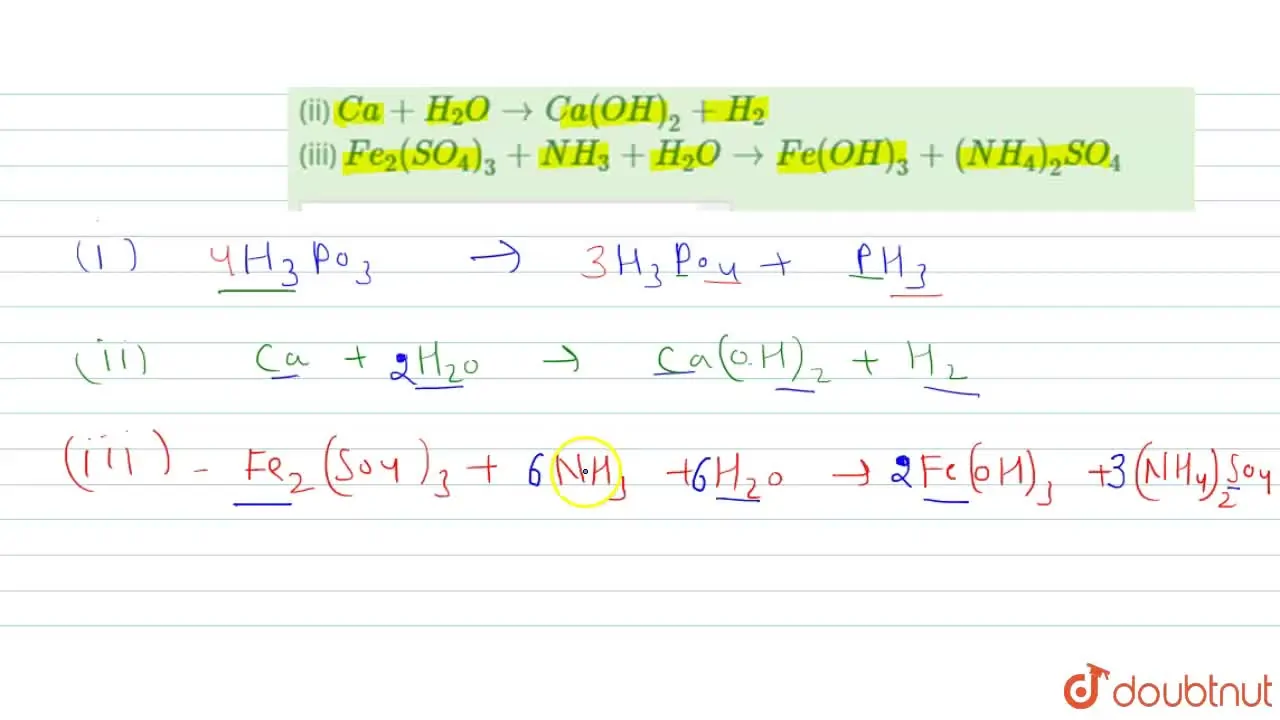
Balance the following equations.
(i) H3PO3→H3PO4+PH3
(ii) Ca+H2O→Ca(OH)2+H2
(iii) Fe2(SO4)3+NH3+H2O→Fe(OH)3+(NH4)2SO4
(i) This equation can easily be balanced by hit and trial method as follows.
The formula H3PO4 contains maximum number of atoms. Therefore, it can be selected to start with. On multiplying H3PO4 by 3 and H3PO3 by 4, we get:
4H3PO3→3H3PO4+PH3
In this equation, the atoms of each kind are the same on both sides of the equation. Hence, the equation is balanced.
(ii) This equation can be balanced by hit and trial method.
Writing hydrogen in the atomic form, we get Ca+H2O→Ca(OH)2+H
Ca(OH)2 can be selected to start with because it contains maximum number of atoms. Oxygen present in it can be balanced by multiplying H2O by 2 and hydrogen can be balanced by multiplying H by 2. Thus, we have:
Ca+2H2O→Ca(OH)2+2H
On writing the equation in the molecular form, we get the following balanced equation:
Ca+2H2O→Ca(OH)2+H2
(iii) This is a bit complicated equation. However, it can be balanced by partial equation method.
The partial equations corresponding to the probable steps of the given reaction are as follows.
Fe2(SO4)3+H2O→Fe(OH)3+H2SO4.....(i)
NH3+H2SO4→(NH4)2SO4 ..........(ii)
On balancing these partial equations by hit and trial method, we get:
Fe2(SO4)3+6H2O→2Fe(OH)3+3H2SO4..........(iii)
2NH3+H2SO4→(NH(4))2SO4..........(iv)
The intermediate species, i.e., H2S04 can be cancelled by multiplying Eq. (iv) by 3 and adding it to Eq. (iii).
Fe2(SO4)3+6H2O→2Fe(OH)3+3H2SO4
[2NH3+H2SO4→(NH4)2SO4]×3
------------------------------------------------------------------------
Fe2(SO4)3+6NH3+6H2O→2Fe(OH)3+3(NH4)2SO4
This is the final balanced equation.
Related Playlists
- BOOK - ICSECHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - REVIEW EXERCISES129videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - ICSECHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - VERY SHORT ANSWER TYPE QUESTIONS34videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 2EXERCISE - Questions62videos
SELF ASSESSMENT PAPER 2
- BOOK - ICSECHAPTER - SOME P-BLOCK ELEMENTS EXERCISE - NCERT TEXTBOOK EXERCISES (With Hints and Solutions)63videos
SOME P-BLOCK ELEMENTS
Similar Questions
Balance the following equation:
Fe+H2O⇔Fe3O4+H2View SolutionBalance the following equation:
Al+H2SO4→Al2(SO4)3+H2View SolutionBalance the following equation :
P+HNO3→NO2+H2O+H3PO4.View SolutionBalance the following equation :
Fe+H2O→Fe3O4+H2View SolutionBalance the following equations by hit and trial method.
Fe+H2O→Fe3O4+H2View SolutionBalance the equations :
P2O5+H2O→H3PO4View SolutionConsider the following reactions:
(i) PCl3+3H2O→H3PO3+3HCl
(ii) SF4+3H2O→H3SO3+4HF
(iii) BCl3+3H2O→H3BO3+3HCl
(iv) XeF6+3H2O→XeO3+6HF
Then according to given information the incorrect statement is:View SolutionBalance the following equation by oxidation number method:
K2Cr2O7+FeSO4+H2SO4→Cr2(SO4)3+Fe2(SO4)+K2SO4+H2OView SolutionComplete the following chemical equations :
(i) HgCl2+PH3→
(ii) SO3+H2SO4→
(iii) XeF4+H2O→
(b) Darw the structure of
(i) (HPO3)3
(ii) BrF3View SolutionH3PO2+CuSO4→Cu↓+H3PO4+HNO3
View Solution