The maximum and minimum values of the objective function Z = 3x - 4 y subject to the constraints
x−2y≤0,−3x+y≤4
x−y≤6,x,y≥0
are respectively

A

12,10

B

10,12

C

12,-16

D

5,12

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Given , objective funciton
Z = 3x-4y .
Subject to the constraints are
x−2y≤0
−3x+y≤4
x−y≤6
x,y≥0
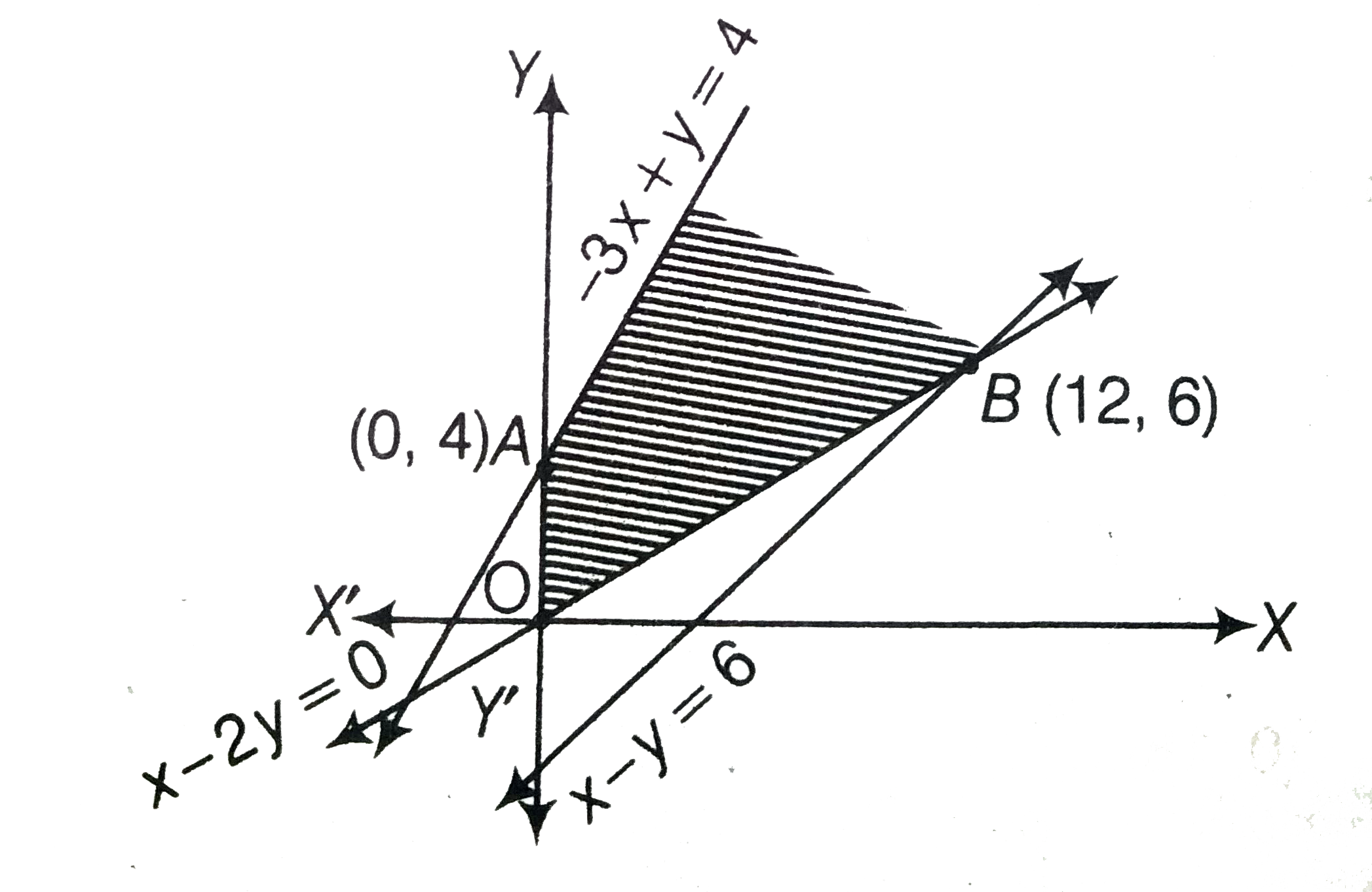
ocr_image
The feasible region which is unbounded is AOB and the carmer points are O(0,0),A(0,4),B(12,6)
ocr_image
Thus, maximum value and minimum value of Z are 12 and -16 respectively. Here, feasible region is unbounded and the half plane determined by 3x−4y>12 and 3x−4y<−16 have no point in common with the feasible region so maximum and minimum values of Z are 2 and 6 respectively

Updated on:21/07/2023
|
Class 12MATHSLINEAR PROGRAMMING

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • The maximum value of the objective function
    Z=3x+4y
    subject to the constraints x+y≤4,x≥0,y≤o is

    A16
    B18
    C20
    D25

  • The minimum and maximum values of the objective function, Z = 5x + 10y subject to the constraints x+2y≤120,x+y≥60,x−2y≥0,x,y≤0 are respectively

    A300 and 500
    B600 and 700
    C600 and 700
    D300 and 400

  • The maximum and minimum values of the objective function Z = x + 2y subject to the constraints
    x+2y≥100,2x−y≤0,2x+y≤200 occurs respectively at

    Aone point and three points
    Btwo points and one point
    Cone point and infinitely points
    Done point and one point