Show that the line 3x−4y−c=0 will meet the circle having centre at (2, 4) and the radius 5 in real and distinct points if −35<c<15.
Video Solution
Text Solution
Given line is 3x−4y−c=0 …(i)
Centre of given circle is (2, 4) and its radius is 5, therefore its equation will be (x−2)2+(y−4)2=52
or x2+y2−4x−8y−5=0 …(ii)
From (1), y=14(3x−c) Putting the value of y in (ii), we get
x2+116(3x−c)2−4x−8⋅14(3x−c)−5=0
or 16x2+9x2+c2−6cx−64x−96x+32c−80=0
or 25x2−2(80+3c)x+c2+32c−80=0 ...(iii)
Line (i) will meet the circle (ii) in real and distinct points if discriminant of equation (iii) <0
i.el., if 4(80+3c)2−100(c2+32c−80)>0
or (80+3c)2−25(c2+32xc−80)>0
or 6400+9c2+480c−25c2−800c+2000<0
⇒−16c2−320c+8400<0
⇒c2−20c−525<0
(c+35)(c−15)<=0
⇒−35<c<15.
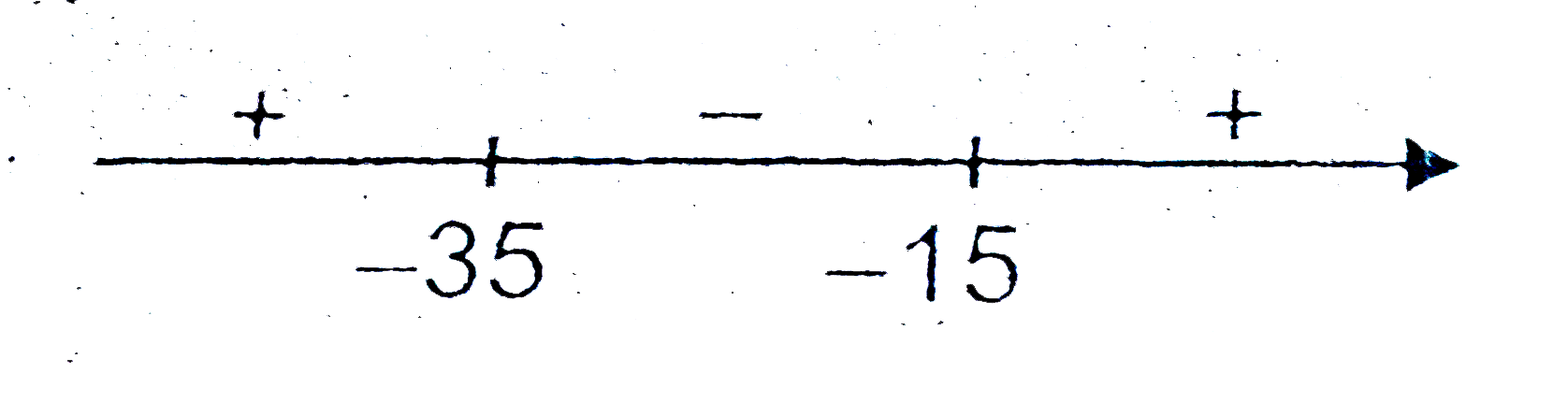
|
Topper's Solved these Questions
- CONIC SECTIONSBOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - Try ypurself
42 Videos
- CONIC SECTIONSBOOK - AAKASH INSTITUTECHAPTER - CONIC SECTIONSEXERCISE - Assignment (SECTION - A)
55 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTECHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - section-J (Aakash Challengers Qestions)
16 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - AAKASH INSTITUTECHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - section - J
6 Videos
Similar Questions
Knowledge Check
3x+4y−7=0 is common tangent at (1, 1) to two equal circles of radius 5. Their centres are the points
A(4, 5), (-2, -3)B(4, -3), (-2, 5)C(4, -5), (-2, 3)DnoneLocus of the centre of the circle touching the line 3x+4y+1=0 and having radius equal to 5 units is
Aa pair of perpendicular linesBa pair of parallel linesCa pair of straight linesDnone of these
Find the equation of the circle having centre at (3,−4) and touching the line 5x+12y−12=0.
View SolutionIf the line y=mx−(m−1) cuts the circle x2+y2=4 at two real and distinct points then
View SolutionShow that the line 3x+4y+20=0 touches circle x2+y2=16 and find point of contact
View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2,5) and the centre of the circles lies on x−2y=4. The radius of the circle is
View SolutionThe line 2x−y+1=0is tangent to the circle at the point (2,5) and the centre of the circles lies on x−2y=4 The radius of the circle is:
View SolutionThe line 2x - y + 1 = 0 is tangent to the circle at the point (2,5) and the centre of the circles lies on x-2y = 4. The radius of the circle is :
View Solution
