Question 1: If P is of order 2 x 3 and Q is of order 3 x 2, then P Q is of order
2×3
2×2
3×2
3×3
The correct Answer is:B
Step by step video & text solution for Question 1: If P is of order 2 x 3 and Q is of order 3 x 2, then P Q is of order by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MATRICESBOOK - AAKASH INSTITUTECHAPTER - MATRICESEXERCISE - Assignment (Section - B) Objective Type Questions (One option is correct)
13 Videos
- MATRICESBOOK - AAKASH INSTITUTECHAPTER - MATRICESEXERCISE - Assignment (Section - C) Objective Type Questions (More than one options are correct)
7 Videos
- MATRICESBOOK - AAKASH INSTITUTECHAPTER - MATRICESEXERCISE - Try yourself
12 Videos
- MATHEMATICAL REASONINGBOOK - AAKASH INSTITUTECHAPTER - MATHEMATICAL REASONINGEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTECHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section-J (Aakash Challengers Questions)
7 Videos
Similar Questions
Let P be the set of all non-singular matrices of order 3 over ú and Q be the set of all orthogonal matrices of order 3 over ú. Then,
View SolutionIn the quadratic equation x2+(p+iq)x+3i=0 ,where p and q are real If the sum of the squares of the roots is 8 ,then the ordered pair (p,q) is given by :
(A) (3,1)
(B) (−3,−1)
(C) (−3,1)
(D) (3,−1)
View Solution
Knowledge Check
Let P be the set of all non - singular matrices of order 3 over R and Q be the set of all orthogonal matrices of order 3 over R. Then
AP is proper subset of QBNeither P is proper subset of Q nor Q is proper subset of PCP∩Q=ϕ the void setDQ is proper subset of PSuppose P and Q are two different matrices of order 3 ×nandn×p ,then the order of the matrix P×Q is ?
A3×pBp×3Cn×nD3×3Suppose P and Q are two different matrices of order 3×n and n×p, then the order of the matrix P×Q is ?
A3×pBp×3Cn×nD3×3
A square matrix A is said to be orthogonal if ATA=I If A is a square matrix of order n and k is a scalar, then |kA|=Kn|A|.Also∣∣AT∣∣=|A| and for any two square matrix A and B of same order |AB|=|A||B| On the basis of above information answer the following question: If A=⎡⎢⎣pqrqrprpq⎤⎥⎦ be an orthogonal matrix and pqr=1,thenp3+q3+r3 may be equal to (A) 2 (B) 1 (C) 3 (D) -1
View Solution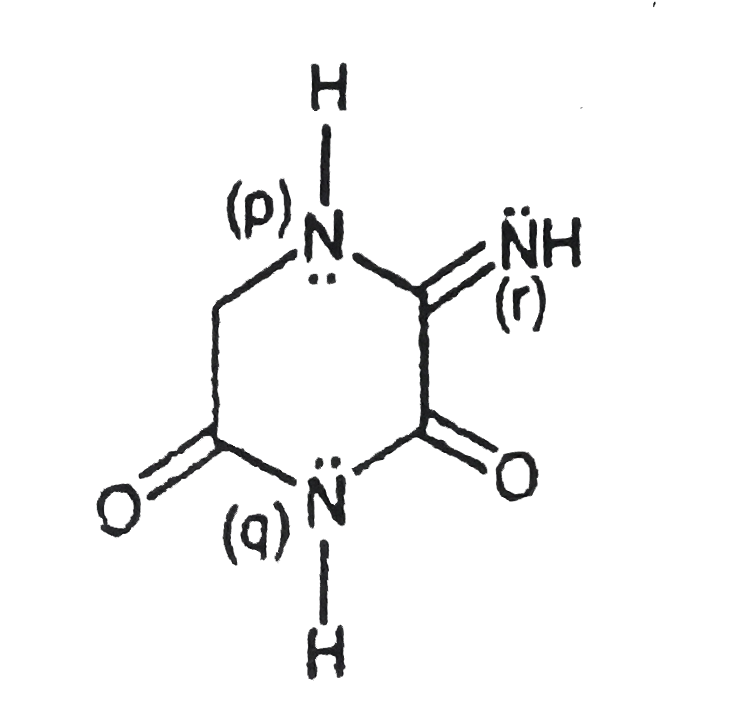
The correct basicity order of atoms p,q and r is :
View SolutionIf the quadratic equation 2x(2)−px+q=0 where p and q are real, then the ordered pair (p,q) is equal to
View SolutionIf 2+√5 is root of x2−px+q=0 where p and q are real, then the ordered pair (p,q) is equal to
View SolutionP(x)=1−1xandQ(x)=x3+x2+x then P(x)×Q(x) is
View Solution
