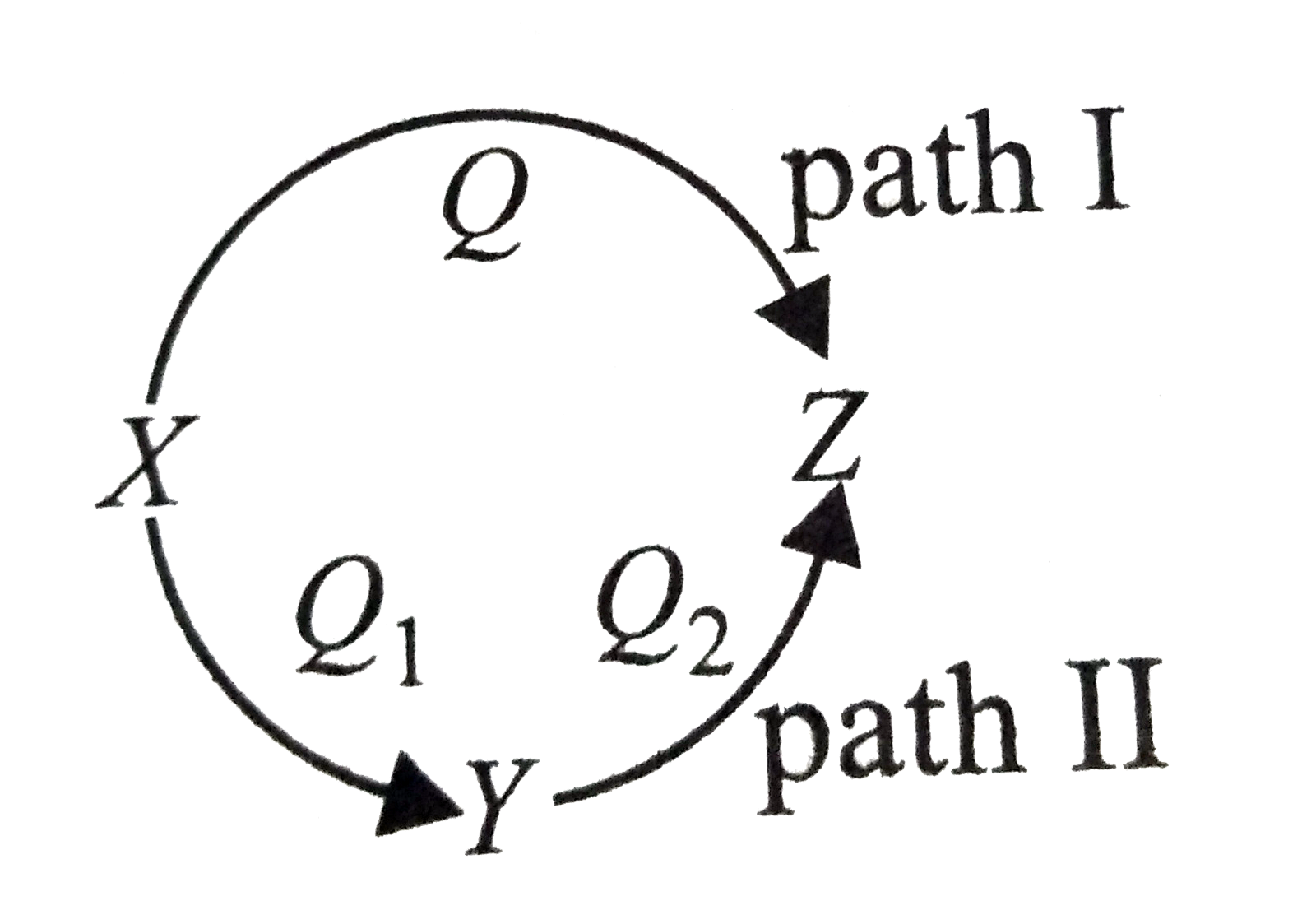
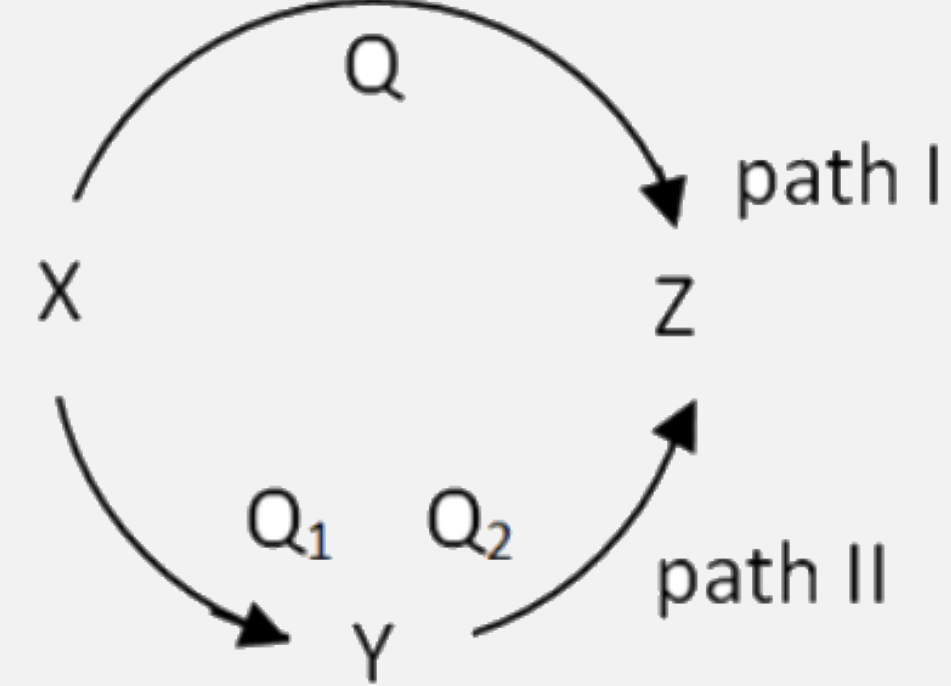
What is the relationship betweenqp and qv ?
To derive the relationship between qp (heat at constant pressure) and qv (heat at constant volume), we can follow these steps:
Step 1: Understand the Definitions
- qp: The heat absorbed or released by a system at constant pressure.
- qv: The heat absorbed or released by a system at constant volume.
Step 2: Apply the First Law of Thermodynamics
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (q) minus the work done by the system (W):
ΔU=q−W
Step 3: Consider Work Done
- At constant volume (V), no work is done by the system since work (W) is defined as PΔV. Therefore, W=0 and:
ΔU=qv
This means that at constant volume, the heat added to the system is equal to the change in internal energy.
- At constant pressure (P), the work done by the system is given by:
W=PΔV
Thus, we can write the first law as:
ΔU=qp−PΔV
Step 4: Relate qp and qv
For an ideal gas, the change in internal energy can also be expressed in terms of temperature change:
ΔU=nCvΔT
where Cv is the molar heat capacity at constant volume.
For constant pressure, the heat added can be expressed as:
qp=nCpΔT
where Cp is the molar heat capacity at constant pressure.
Step 5: Use the Relationship Between Cp and Cv
For an ideal gas, there is a relationship between Cp and Cv:
Cp=Cv+R
where R is the universal gas constant.
Step 6: Substitute and Rearrange
Now substituting Cp in the equation for qp:
qp=n(Cv+R)ΔT
This can be rewritten as:
qp=nCvΔT+nRΔT
Now, since ΔU=nCvΔT, we can replace nCvΔT with qv:
qp=qv+nRΔT
Conclusion
Thus, we arrive at the relationship:
qp=qv+nRΔT
- qp: The heat absorbed or released by a system at constant pressure.
- qv: The heat absorbed or released by a system at constant volume.
The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (q) minus the work done by the system (W):
ΔU=q−W
- At constant volume (V), no work is done by the system since work (W) is defined as PΔV. Therefore, W=0 and:
ΔU=qv
This means that at constant volume, the heat added to the system is equal to the change in internal energy.
W=PΔV
Thus, we can write the first law as:
ΔU=qp−PΔV
For an ideal gas, the change in internal energy can also be expressed in terms of temperature change:
ΔU=nCvΔT
where Cv is the molar heat capacity at constant volume.
qp=nCpΔT
where Cp is the molar heat capacity at constant pressure.
For an ideal gas, there is a relationship between Cp and Cv:
Cp=Cv+R
where R is the universal gas constant.
Now substituting Cp in the equation for qp:
qp=n(Cv+R)ΔT
This can be rewritten as:
qp=nCvΔT+nRΔT
Now, since ΔU=nCvΔT, we can replace nCvΔT with qv:
qp=qv+nRΔT
Thus, we arrive at the relationship:
qp=qv+nRΔT
Topper's Solved these Questions
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - SHORT ANSWER QUESTIONS
65 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - COMPETITION FOCUS (JEE ( main andAdvanced ) /Medical Entrance Special)
131 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - LONG ANSWER QUESTIONS
21 Videos
- STRUCTURE OF ATOMBOOK - PRADEEPCHAPTER - STRUCTURE OF ATOMEXERCISE - Competition Focus (JEE (Main and Advanced)/Medical Entrance (IX. Assertion And Reason Type Questions (Type II))
12 Videos
Similar Questions
Knowledge Check
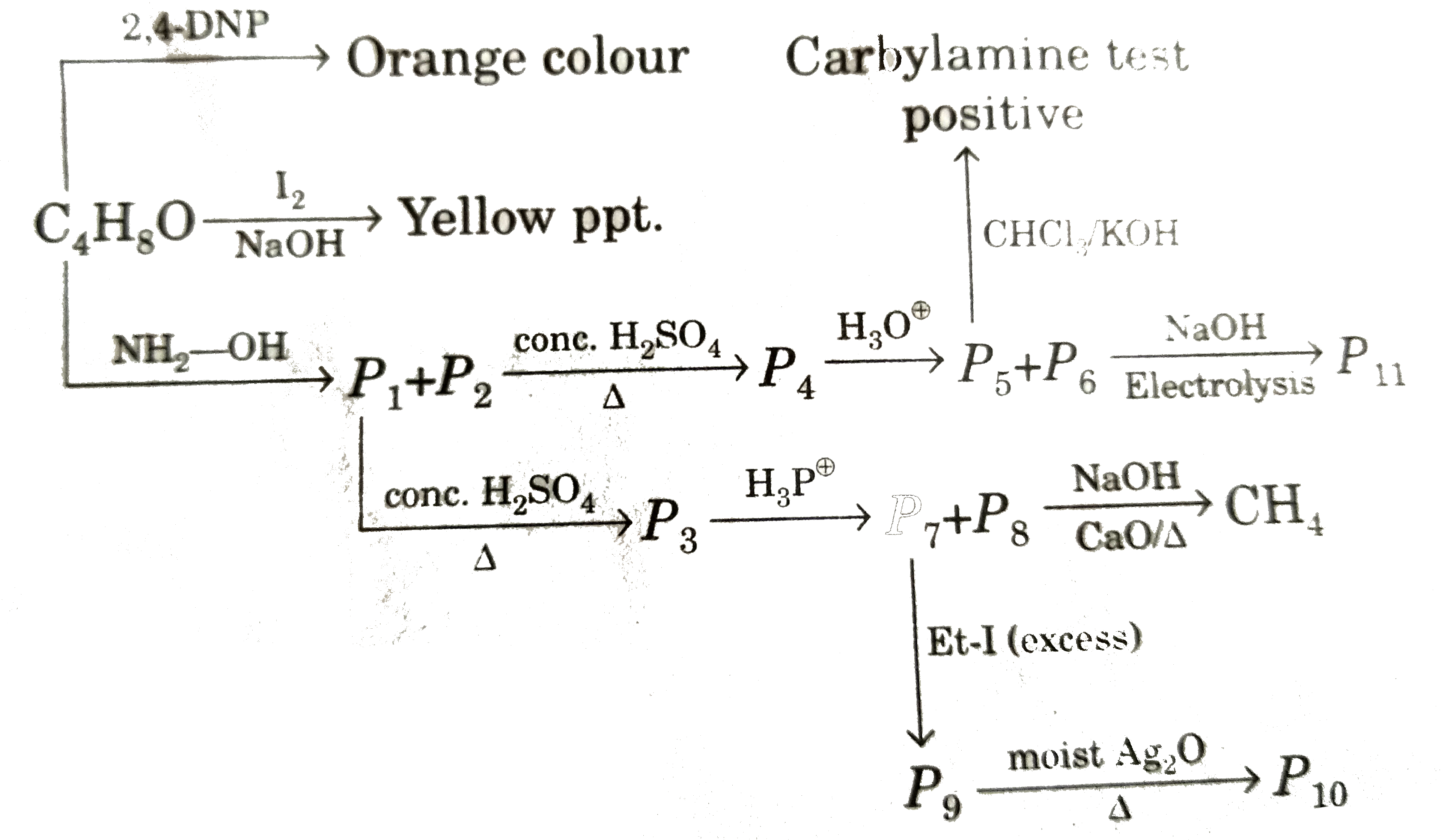
What is the relationship between P1 and P2AGeometrical isomersBOptical isormersCPositional isomersDChain isomers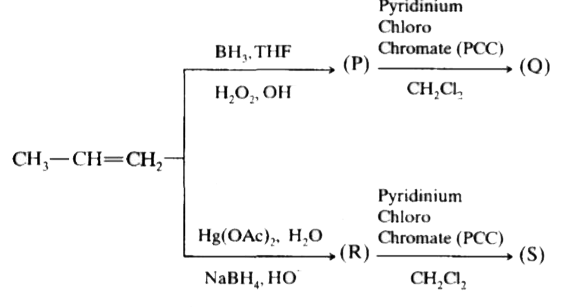
Relationship between products (Q) and (S) isApositional isomerBchain isomer.Cstereoisomer.Dfunctional isomer.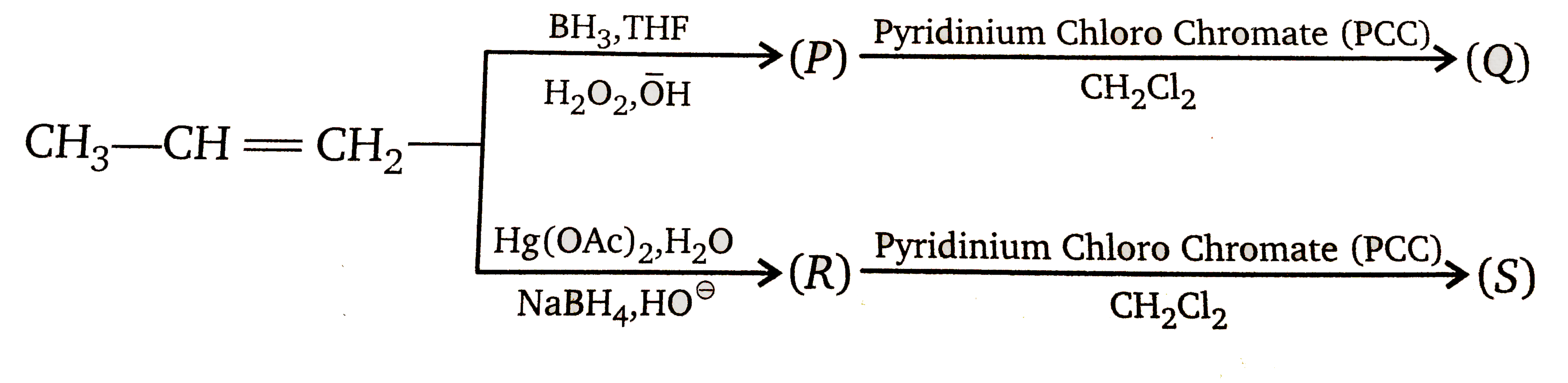
Relationship between products (Q) and (S) is :APositional isomerBChain isomerCStereoisomerDFunctional isomer
P+Q' means 'P is the sister of Q'.
'P@Q' means 'P is the wife of Q'.
'P$Q' means 'P is the son of Q'.
'P %Q' means 'P is the mother of Q'.
What is the relation between 'J and A' in the expression 'A @F$M%J+T'?
View SolutionThe resultant →Pand→Q is perpendicular to →P. What is the angle between →Pand→Q?
View SolutionWhat is the resistance between P and Q ?
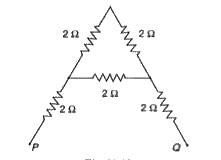
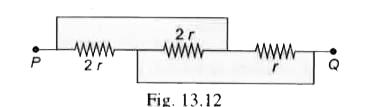
View SolutionWhat is the effective resistance between points P and Q?
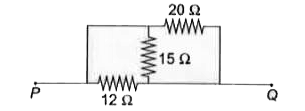
View SolutionWhat is the effective resistance between points P and Q?
View Solution