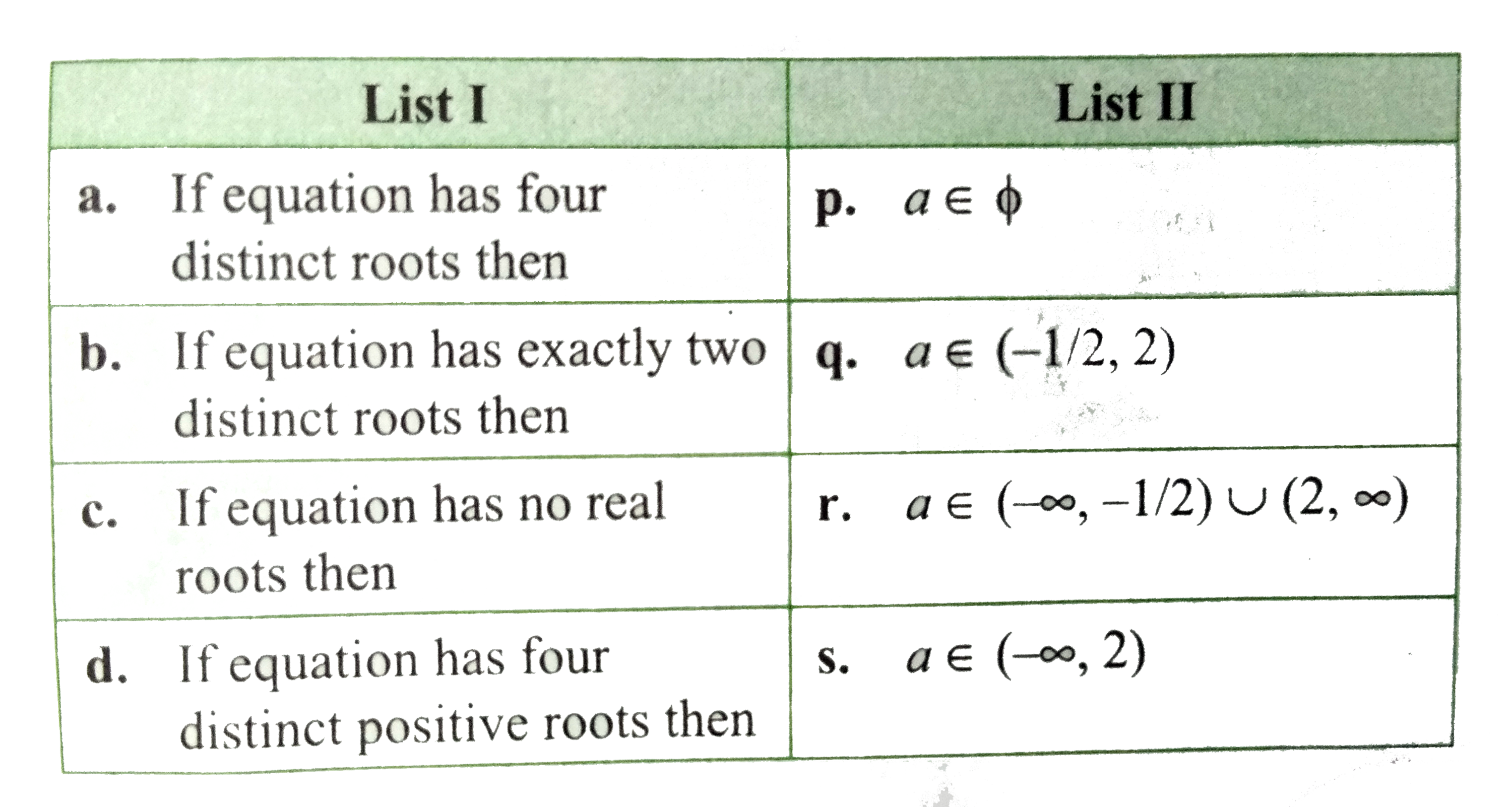
Consider equation $x^{4} - 6 x^{3} + 8 x^{2} + 4 a x - 4 a^{2} = 0, a \in R$ . Then match the following lists:

$\begin{matrix} a & b & c & d (1) & q & s & s & r \end{matrix}$

$\begin{matrix} a & b & c & d (2) & r & s & q & p \end{matrix}$

$\begin{matrix} a & b & c & d (3) & q & s & r & p \end{matrix}$

$\begin{matrix} a & b & c & d (4) & q & r & p & p \end{matrix}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

Updated on:21/07/2023

The equation $(x^{2} - 6 x + 8) + λ (x^{2} - 4 x + 3) = 0, λ \in R$ has
Areal and unequal roots for all $λ$
Breal roots for $λ < 0$
Creal roots for $λ > 0$
Dreal and unequal roots for $λ = 0$
If $α, β, γ$ are the roots of the equation $x^{4} + A x^{3} + B x^{2} + C x + D = 0$ such that $α β = γ δ = k$ and A,B,C,D are the roots of $x^{4} - 2 x^{3} + 4 x^{2} + 6 x - 21 = 0$ such that $A + B = 0$
The value of $\frac{C}{A}$ is
A $- \frac{k}{2}$
B $- k$
C $\frac{k}{2}$
D $k$
If $α, β, γ, δ$ are the roots of the equation $x^{4} + A x^{3} + B x^{2} + C x + D = 0$ such that $α β = γ δ = k$ and A,B,C,D are the roots of $x^{4} - 2 x^{3} + 4 x^{2} + 6 x - 21 = 0$ such that $A + B = 0$
The correct statement is
A $C^{2} = A D$
B $C^{2} = A^{2} D$
C $C^{2} = A D^{2}$
D $C^{2} = {(A D)}^{2}$